Is the Copenhagen interpretation falsifiable?

Question

According to the Copenhagen interpretation, physical systems generally do not have definite properties prior to being measured. The Schrödinger's cat is both dead and alive, until an observation is made.

Is this interpretation falsifiable? If it's not, why is it taken so seriously?

EDIT: Some of you made good points, so I need to explain my thought better. I remember a problem in philosophy, asking whether objects exist when we're not looking at them. In my opinion, this question is meaningless/pointless and merely a matter of complexity of language. Is it the same case with Copenhagen interpretation? Does it really say anything about the world?

My second question is about the terminology used to describe quantum states. It reminds me of the probability vs fuzzy logic controversy. Is it possible to understand quantum theory merely through probabilities, without the 'self contradictory' descriptions?

I just opened a related question: Interpretations of a scientific theory

Answer 1

The Schrödinger's cat is both dead and alive, until an observation is made.

This is a very misleading ( and cruel) gedanken experiment, using a cat as a detector for the quantum mechanical behavior of decaying particles.

There is no way one can tag which particle will decay, each has a probability of decaying, and the cat experiment stresses the probabilistic nature of quantum mechanics and confuses macroscopic perception with microscopic reality, imo.

Macroscopic objects have to be treated classically, composed as they are of order 10^23 wavefunctions which at the cat level are incoherent and therefore classical in behavior.

There is no way to distinguish between interpretations experimentally. They describe the same data. If they do not, they would not be called interpretations. An interpretation which did not agree with all microscopic measurements would be falsified and no longer be in the list.

Edit, after edit of question:

Is it the same case with Copenhagen interpretation? Does it really say anything about the world?

It uses the mathematical model on which it is based to describe existing experimental data and predict future behavior. Up to this time it is continuously validated even when extended to new kinematic regimes as with special and general relativity.

The other interpretations in the list either have their problems (cannot be extended) to new kinematic regimes as with the Bohm theory, or are too complicated conceptually to help in developing intuition in the microcosm data, and thus main stream physics teaching does not use them.

In this sense it is discussing the complexity of mathematical language.

It is possible, (as with the case of Newton's "particulate nature of light") that future experimental data in new kinematic regimes might pick another interpretation than the C one for new data from higher energy regimes or unthought-of at the present boundary conditions. This would not invalidate the usefulness of the C interpretation's simplicity in the existing data. We still use classical mechanics in the proper regime.

Is it possible to understand quantum theory merely through probabilities, without the 'self contradictory' descriptions?

In mainstream physics experience, yes.

The simplest way to understand the double slit experiment , one electron at a time, that shows that at the quantum level the electron is a quantum mechanical particle, is the Copenhagen interpretation, with probabilities.

In the top frame the dots are the footprints of electrons in the experiment "electron scattering off two slits of given geometry". It is what is expected of a particle, a specific signal at (x,y,z). The gradual accumulation though shows the interference pattern of a wave. There is no self contradictory description. Just the discovery that at the microcosm particles are not billiard balls, which have a random probability distribution when they scatter. Electrons have other attributes, which the C interpretation describes by using probabilities of interaction with the complex conjugate square of a specific wavefunction, of specific differential equations(,called wave equations because of the sinudoidal solutions they give) in the given boundary conditions of electrons scattering off two slits.

Edit:

In a comment to another answer you say your underlying bias:

My interpretation is that the world is deterministic, and the probabilities arise from lack of enough knowledge to predict the states. Isn't this interpretation simpler? Is this also a common view among quantum scientists, I mean the determinism?

At present, as you were answered in the comments, the determinism comes in the probability distributions, those can be determined absolutely given the boundary conditions.

The pilot wave theory of Bohm tries to generated classical probability distributions to explain the success of the Schrodinger equation solutions, and it succeeds at non relativistic energies, that is why it is called an interpretation. It is extremely complicated and stumbles when it comes to special relativity. As far as Occam's razor goes, it is really very complicated, theoretically, and has not caught on.

Answer 2

It has come up in the previous discussion that an interpretation is different from a theory in that a theory can be disproven, while an interpretation cannot. Indeed, if all of the interpretations of QM are complete, they all have the same predictions and therefore cannot be distinguished experimentally.

Really, an interpretation is a tool, and it can be falsified in a certain sense. Interpretations help us understand the context of a theory, to apply it, and to extend it. They help us to determine when a theory is relevant to experiment and relevant to our intuitive understanding of how the world works. If an interpretation would lead us to apply an established theory to a new situation, and then the theory fails in that situation, what was falsified? You could argue that the interpretation was. And if this failed extension of the theory leads to a tweaking of the interpretation, it could color our understanding of all of the previous successful applications of the theory.

Typically, an interpretation precedes the theory. Think about it: if you want to develop a quantitative theory of how something works, you start with your intuition of the system. You think about what are the relevant physical parameters and variables, as well as how they might interact. Only then do you write down an equation describing the system. This equation is your theory, and it makes predictions which can be falsified, provided you understand (through your interpretation) the meaning of the variables of the equation. My favorite example is $F=ma$, which one might argue is true by definition. But it not simply a definition because it is totally useless if we don't have an interpretational understanding to connect to our world of what is a force, a mass, and an acceleration. What, really, are these things? The theory is build from the interpretation.

But quantum mechanics is different. For QM, the equations historically came first. What the equations predict is so counter-intuitive that there is no agreed-upon interpretation which unambiguously connects the mechanics of QM to the world we live in, and we currently have multiple interpretations all "explaining" the same fundamental mechanics. Whenever a new experiment comes along which might be in conflict with an interpretation (such as, for example, the Afshar experiment "disproving" the interpretational principle of complementarity), then the interpretations adapt (although some adapt more naturally than others).

But does that mean that interpretations are useless for QM? Should we just "shut up and calculate"? No, of course not. As I mentioned, interpretations are tools. They can still be used to help us understand how to apply the QM to new situations and give us a context with which to extend the theory as needed. In as much as an interpretation is useful for this, it is a good interpretation. If you like Bohmian determinism, and you can use it to help you apply QM, then it is successful. If it leads you to apply or extend QM in a way that is inconsistent with experiment, then it fails. This is the manner in which an interpretation an be "falsified".

Answer 3

In fact, the stimulus to Copenhagen interpretation was something like "we only know the things we get from measurements, so we don't care what happens in between". Consider two statements:

A. We get this state with this probability upon doing measurements and we don't care what happens in between.

B. Between measurements system is at all possible states simultaneously.

If you want to falsify Copenhagen interpretation, you have to falsify statement A, not statement B.

Ninja edit: Some authors claim (I don't know where do they know it from) that when Maxwell was working on his theory of electrodynamics, he was imagining the space filled with little gears and cranks which transfer the influences. However, when you want to falsify Maxwell, you aim at his predictions (what will we get if we measure this thing in that way) not at those gears.

Answer 4

Nobody has yet come up with an experimental way to distinguish between the Copenhagen interpretation and the Many Worlds interpretation. In both cases, the undetermined possibilities are inaccessable until they are measured; and after a measurement is done all of the accessible aspects of reality are those that are consistent with the outcome of the measurement. The Many Worlds interpretation says that the other possibilities remain real but inaccessible; the Copenhagen interpretation says that they cease to be real and are therefore inaccessible. From the experimentalist's perspective there is no difference. Some would say that Ockham's Razor favors Copenhagen; some would say it favors Many Worlds; each seems absurd in its own way but both have passed all experimental tests so far.

Answer 5

An interpretation cannot be falsified by experiment but can be by logic. The CI concept of wave function collapse violates causality. This fact falsifies CI for me, as I am not willing to abandon causality for the sake of an interpretation.

Answer 6

If we place the Copenhagen interpretation within the broader class of objective collapse interpretations then yes, it is possible to 'falsify' the Copenhagen interpretation.

These interpretations claim that at all times there is a chance for a superposition state to collapse into (or towards) one state of the superposition or the other. The probability of this collapse is related to the "bigness"* of the superposition. Thus, if you create a superposition of a large rock being in two places at once it will collapse very quickly, whereas a superposition of an electron in two places at once will hardly ever collapse. Of course, once the electron is measured it is now entangled with the measurement device which is "big". This means as soon as the measurement is performed it becomes highly likely that the system will collapse immediately.

This postulate of "collapse" requires an extension to the unitary time evolution of "orthodox"* quantum mechanics which Many-worlds interpretations take very seriously. This extension means that the laws of physics for the objective collapse models are different than the laws of physics of "orthodox" quantum mechanics. The difference should be physically measurable. In fact it is.

Conventional quantum mechanics says that if you create a superposition state and this superposition doesn't interact with anything then it will persist forever. The objective collapse models say that even if the system doesn't interact with anything the system will still collapse, probabilistically, after some amount of time. This is a testable difference.

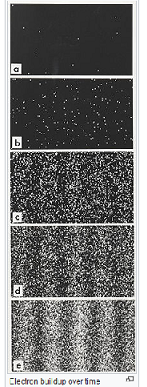
I propose the following double slit experiment. Put a particle in a superposition. Pass it through a double slit. Let the superposition propagate trough space for time $T$. Measure the location of the particle. Repeat many times. If the superposition persists then looking at all of the results you will see an interference pattern. If it does not then you will see the interference pattern wash away and the contrast decreases.

Now, vary the time $T$ and repeat. Now, vary the mass $M$ or size of the particle and superposition state

Orthodox quantum mechanics says that as $T$ or $M$ is increased we should see no change in contrast. The objective collapse models say that the interference contrast will decrease as $T$ and $M$ are increased. Thus these two particular interpretations can be experimentally distinguished.

Now, there is a VERY important point here. Above I have stipulated that it is important that the superposition does not interact with anything else (air particles, photons, magnetic fields, etc.) while it is in the superposition. This is because, due to decoherence, if the particle interacts with anything that will ALSO be a source of contrast reduction. This is why it is hard to perform these experiments in practice. It is very hard to make it so that the quantum systems don't interact with anything. And the bigger the system is the harder it is to ensure there is no decoherence.

So to perform the experiment above we must create the superposition and see if it decays as a function of time but we must also have controlled the experiment well enough so that we can rule out any normal forms of decoherence as causing the reduction in contrast. For example, There are researchers now creating bigger and bigger superposition. As they make the superposition bigger or wait longer they DO see a reduction in contrast. However, they are able to explain this as being due to typical experimental imperfections/decoherence. Thus, they have yet compared the two interpretations in a significant way.

However, that last statement is not quite right. By performing that experiment they have said something about the rate of this "spontaneous collapse". They can say that they have experimentally determined that whatever the rate of spontaneous collapse is (call it $\Gamma_{SC}$), they know that it is SLOWER than the rate of decoherence, $\Gamma_{D}$, that they can explain in their experiment.

They have experimentally verified the following constraint for their choice of $T,M,N$ etc.

$$ \Gamma_{SC}(T,M,N) < \Gamma_D $$

"Orthodox" quantum mechanics would say $\Gamma_{SC}=0$ whereas objective collapse models say $\Gamma_{SC}>0$. The goal of these superposition experiments is to increase $T$ and $M$ (thus increasing $\Gamma_{SC}$) while decreasing $\Gamma_D$ to see if they can perform a measurement that breaks this inequality which would require us to definitely add the objective collapse laws to the laws of quantum mechanics.

As I've mentioned it is very difficult to control and reduce the decoherence rate $\Gamma_D$. We are still many orders of magnitude away from performing definitive measurements ruling out objective collapse models for macroscopic objects. It may be decades or more before we are able to control quantum systems well enough to try to make really really big (like gram scale) superpositions but people will continue to work towards it.

See This article for some information about research going on now into making bigger and bigger superpositions.

*Here bigness can mean the mass of the particles involved in the superposition, the number of particles, the distance of the superposition etc.

**Here I use "orthodox" to mean unitary evolution of the wave function only. No collapse. This may be in direct conflict with usage where "orthodox" quantum mechanics means the Copenhagen interpretation. That is why I clarify here. Perhaps it would be better to say "Everettian quantum mechanics".

Answer 7

The randomness of the outcome of repeated application of Born's Rule is an essential part of the Copenhagen Interpretation. If we find that, for instance, repeated measurements of a spin alternatingly along x and y axes produces a string of up-down results whose Kolmogorov complexity is substantially less than the length of the string, itself; i.e. an algorithmically compressible string, then that counts as falsification.

Answer 8

Many Interacting Worlds is a subtle way to reconstruct Bohm quantum mechanics from many classical worlds interacting by some simple pair-wise interactions, and in principle can be distinguished from the other traditional theories. It is usually argued that the different interpretations of quantum mechanics are just a class of equivalence that differ only in language but not in observable consequences

As a matter of fact, the strictest reading of the Copenhagen interpretation has been already falsified by the Quantum Eraser experiment