I'am not sure about some things about derivative interactions. Lets say I have to following 2 interaction terms:
$$\mathcal{L} = \left(a\phi + b\phi^{2}\right)\partial_{\mu}\phi\partial^{\mu}\phi.$$
Then I can write the vertex factor for the Feynman rules as follows (following Srednicki QFT conventions):
$$V_{a} = \left( -ia \right)\left(-ik^{\mu}\right)\left(ik^{\mu}\right) $$
$$V_{b} = \left( -ib \right)\left(-ik^{\mu}\right)\left(ik^{\mu}\right) $$
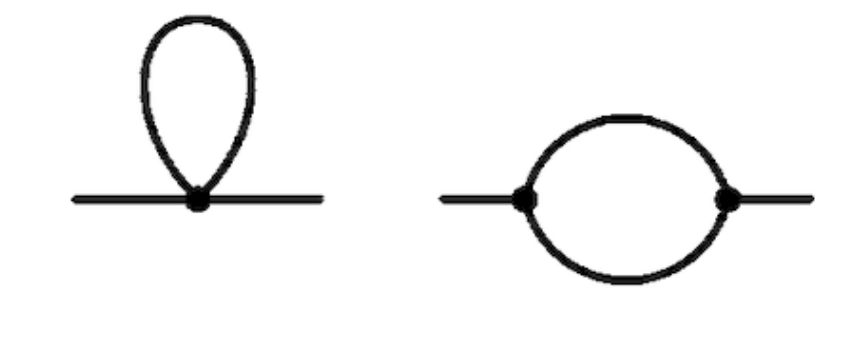
with $\left(-ik^{\mu}\right)$ for incoming momenta and a $\left(ik^{\mu}\right)$ for outgoing momenta.Now I wish to calculate the te loop corrections to the propegator which I think are these two:
with derivative fields in the loops. My question is, is the final result for the amplitude for the diagrams always proportional to $k^{2}$? Because in my calculations I have something like this:
$$\Pi_b \propto \frac{-i}{2} \int \frac{\mathrm{d}^{4}l}{\left(2\pi \right)^{4}}\frac{\left(-i\right)}{l^{2} + m^{2} - i\epsilon}\left(-il^{\mu}\right)\left( il^{\mu}\right)\left(-ib\right) = \frac{ib}{2} \int \frac{\mathrm{d}^{4}l}{\left(2\pi \right)^{4}}\frac{\left(l^{2}\right)}{l^{2} + m^{2} - i\epsilon} $$
$$\Pi_a \propto \frac{-i}{2} \int \frac{\mathrm{d}^{4}l}{\left(2\pi \right)^{4}}\frac{\left(-i\right)}{l^{2} + m^{2} - i\epsilon}\frac{\left(-i\right)}{\left(l + k\right)^{2} + m^{2} - i\epsilon}\left\{\left[-il^{\mu}\right]\left[ i\left(l + k\right)^{\mu}\right]\right\}^{2}\left(-ia\right)^{2}\\ \qquad = \frac{-ia^{2}}{2}\int \frac{\mathrm{d}^{4}l}{\left(2\pi \right)^{4}} \frac{1}{l^{2} + m^{2} - i\epsilon}\frac{1}{\left(l + k\right)^{2} + m^{2} - i\epsilon} \left[ l^{2} + \left(k\cdot l\right) \right]^{2} $$
does the above calculation looks right to you, or am I labeling the momenta wrong?
References for derivative couplings:
Chapter 7.4.2 of qft Schwartz