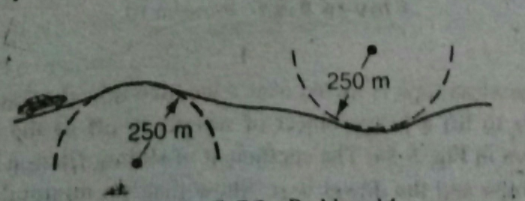
A car moves at a constant speed on a straight but hilly road. One section has a crest and dip of the same 250-m radius. (a) As the car passes over the crest, the normal force on the car is one-half the weight of the car $(mg)$. What will be the normal force on the car as it passes through the bottom of the dip? (b) What is the greatest speed at which the car can move without leaving the road at the top of the hill? (c) Moving at the speed found in (b), what will be the normal force on the car as it moves through the bottom of the dip?
Here is what I have tried so far. I know that an object on a $\theta$ degrees slope will experience a normal force of $F_n = mg \cos(\theta).$ So, if a normal force is being acted upon a body is known, we can compute the angle of inclination using this relation and so theta would be:
$$\frac{1}{2} mg = mg \cos(\theta).$$ $$\theta = \arccos( 1/2 )$$ $$\theta = \frac{\pi}{3}\ \mathrm{radians}$$
But for a dip the angle of inclination will still be the same and so the normal force's magnitude will still be $\frac{1}{2} mg.$
But I am not sure wheather this answer is correct. And I also can't solve the other two parts of the question.