3
$\begingroup$

It is intuitive to me (correct me if I am wrong), that the direction of the wave vector in (real, not modeled) laser beam is not aligned with the direction of propagation everywhere in space. It means, that spherical-wave-like behavior gets integrated into plane-wave-picture, and the error, associated with plane-wave picture $\vec{k} || \vec{r}$, is the most noticeable in tightly-focused beams.

Now, my question is:

Is there a mathematical description of how the wave vector in such beams depends on the position in the beam spot? Can I extract it somehow from, maybe, Fourier analysis?

Additional note: the direction of $\vec{k}$ is related to the polarization basis, I think.

Improve this question
$\endgroup$

2 Answers 2

Reset to default
3
$\begingroup$

A physical laser beam such as the Gaussian beam discussed by Chronicler can be expressed as a superposition of plane waves. This is best expressed in terms of Fourier Optics as $$ f({\bf x}) = \int F({\bf k}) \exp(-i{\bf x}\cdot{\bf k}) d^2k , $$ where $f({\bf x})$ is the profile of the laser beam and $F({\bf k})$ is called the angular spectrum. The exponential function represents the expression for a plane wave. Each plane wave has a propagation vector ${\bf k}$ associated with it. The laser beam as a whole has a specific propagation direction. So therefore the propagation direction of the whole laser beam is not the same is the propagation vectors of the individual plane waves. It also shows that there does not exist a direct relationship between the point in the Gaussian beam and the propagation vectors of the plane waves. It takes the whole spectrum of plane waves to compose the field of the laser beam at every point in space.

One can compute the gradient of the phase at a point in the beam and associate a propagation direction to that point based on that, but this propagation direction does not have a direct relationship to the plane waves that compose the beam.

The Gaussian beam is a solution of the paraxial wave equation, which follows from the Helmholtz equation under the paraxial approximation. It is not a solution of the Helmholtz equation. So when you have a tightly focussed beam, then the paraxial approximation does not apply.

The relationship between the propagation vector and the state of polarization of a plane wave is simply stated by saying that the polarization vector is perpendicular to the propagation vector. Under the paraxial approximation, one often assumes that the polarization vector is perpendicular to the direction of propagation of the whole beam. However, when the paraxial approximation does not apply, the state of polarization can be more complicated.

Improve this answer
$\endgroup$
7
  • $\begingroup$ But you cannot integrate beyond $k=\omega/c$. It would be unphysical. Otherwise you get into evanescent waves domain, which should not contribute in far field. So, this is already not the full spectrum... Anyways, each pw component comes with the associated weight, hence, one should be able, in principle, to map the contribution of each pw wave onto $\{x,y\}$ plane in the spot size, given that $z$ is the propagation direction. $\endgroup$
    – MsTais
    Commented Feb 16, 2018 at 4:12
  • 1
    $\begingroup$ Correct. All that it implies is that, if the field does not contain an evanescent part, then the angular spectrum is zero outside the radius $k=\omega/c$. The formalism gives the correct behaviour even if there is an evanescent part. $\endgroup$
    – flippiefanus
    Commented Feb 16, 2018 at 10:23
  • $\begingroup$ Not sure if I understand the rest of your comment correctly, but the expression I gave, tells you exactly how the different plane waves contribute at each point on the $x,y$-plane. I didn't show how the result changes as a function of $z$ (because your question doesn't seem to ask for that), but one can extend the formalism to handle that fairly easily. $\endgroup$
    – flippiefanus
    Commented Feb 16, 2018 at 10:30
  • $\begingroup$ Yes, absolutely! Thanks for clarifications! I meant, that I have never seen $\vec{k} = \hat{k}\cdot k(x,y)$ with explicit form of $k(x,y)$, where $\hat{z}$ is the direction of propagation. I understand that this information is hidden in Fourier transform, but I have not seen it being extracted in functional form. $\endgroup$
    – MsTais
    Commented Feb 16, 2018 at 16:29
  • $\begingroup$ "The formalism gives the correct behaviour even if there is an evanescent part." - can you reference to the mathematical proof of this statement. I get the same feeling, but since I can't strictly prove it, I always run into problems when talking about this. There is an ongoing debate whether to integrate of $k\in [0,\infty]$, or to cut off at $\omega/c$ when doing AS decomposition. $\endgroup$
    – MsTais
    Commented Feb 16, 2018 at 16:33
2
$\begingroup$

A laser beam can be described by a Gaussian Beam. I studied it from here: https://www.colorado.edu/physics/phys4510/phys4510_fa05/Chapter5.pdf

Its derivation is a bit brutal, but it includes the main results: between them there are the section of the beam (perpendicular to the propagation direction), the beam profile and the wavefront, which (if I understood) is what you are looking for, since the wave vector is always perpendicular to the wavefront. A gaussian beam looks like this: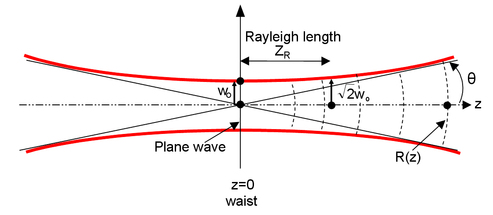gaussian beam

( I took this image from google images, it shows the profile of a gaussian beam propagating along $z$, that exhibits cylindrincal symmetry around that axis. The vertical axis is the radius of the beam, in particular the radius within which $\sim 90$% (usually) of the total power is contained)

You can see that the beam widens as $z$ grows. After a certain distance, called Rayleigh length, it starts to enlarge like a cone (the profile of the beam is described by an hyperbole). The position of the point $z_0$ where the width of the beam ($w_0$) is minimum is called waist: $w_0$ determines how fast the width of the beam will grow with $z$ (smaller $w_0$ means faster growth). The section of the beam is a gaussian, so most of the power is concentrated in the centre, while it decreases fast as the radius increases.

Finally, you can see that the wavefront is plane in $z_0$, but becomes spherical as the beam propagates along $z$: a funtion $R(z)$ describes the curvature of the wavefront.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thanks, it confirms my understanding. I need a functional relation between $\vec{k}$ and $\rho$ - position with respect to the symmetry axis of the beam (given cylindrical symmetry). $\endgroup$
    – MsTais
    Commented Feb 14, 2018 at 21:48
  • $\begingroup$ $R(z)=z+z_R^2/z$, with $z_R=(\pi n w_0)/\lambda$). In the regime where $R(z)\simeq z$, you can treat the beam's wavefront as the one of a spherical wave produced by a source in $z=0$. I tried some calculations for the other regions but it seems to be difficult solving analytically, so I have to do approximations. I don't want to post results I am not sure about at the moment. I'll integrate the answer if I find something $\endgroup$
    – Chronicler
    Commented Feb 15, 2018 at 0:00

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.