0
$\begingroup$

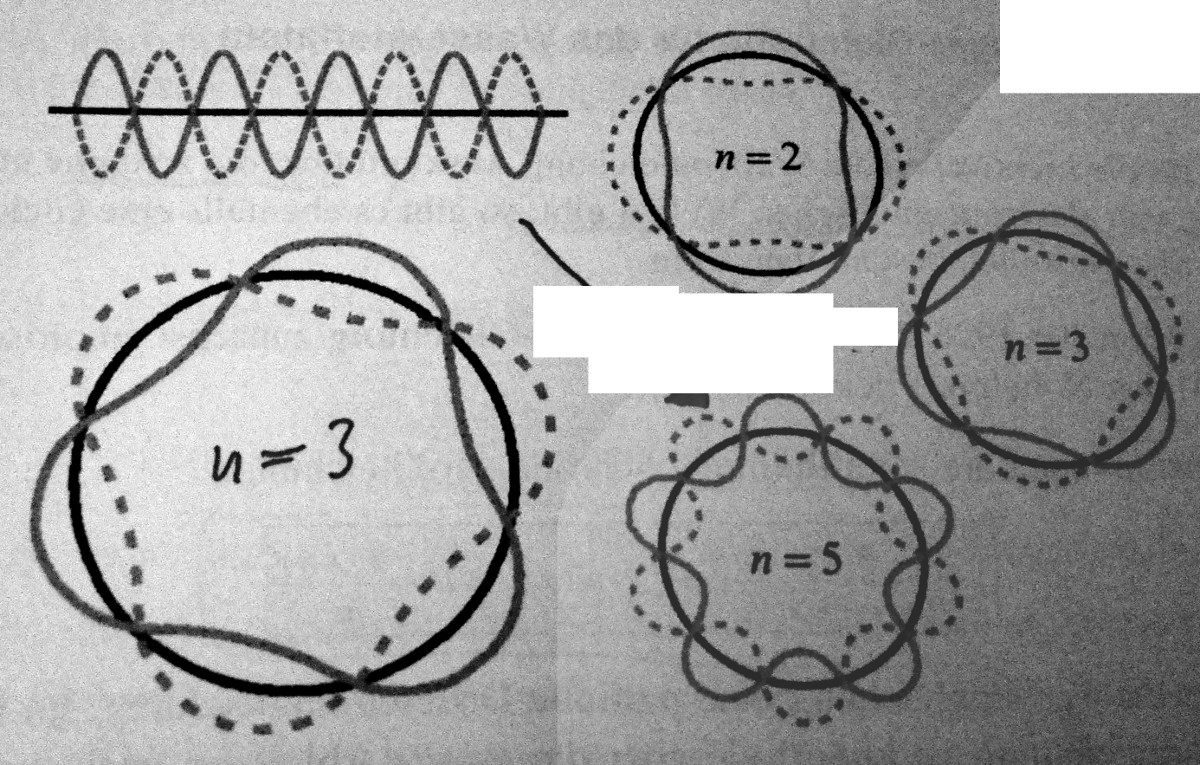
The solution for the Schrödinger equation for a particle in a box, e.g. an electron, is a standing wave with the length $\lambda/2*n$ with $n$ as the number of the orbit or the so called state. Furthermore $n$ is called the principal quantum number.

Standing waves of a particle in the box

This standing wave moves up and down with its amplitude but the knots of the standing wave do not move. Now imagine to take these waves and their discrete lengths and wrap them around a circle. What you get, in the opinion of my university professor, is the following:

enter image description here

If I got it right, an electron in a box, considering the Schrödinger equation, has the same matter wave as an electron on an atom orbit. So it moves in the same way if you hit the standing wave.

But I have to say, I do not know how that should work. Why should the particle in the box and the particle on the orbit act identically? And as you can see, there are twice as many half-periods of the wave for each $n$ in the second picture along the orbit as calculated before.

Improve this question
$\endgroup$
5
  • 1
    $\begingroup$ The boundary conditions of a de Broglie wave-on-a-circle are those of a periodic box, so the states for a circumference $L$ represent only half those for a rigid box of width $L$. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Feb 5, 2018 at 21:19
  • $\begingroup$ Why is that so? Why is $L$ now twice the value it was before? $\endgroup$
    – Kutsubato
    Commented Feb 6, 2018 at 15:41
  • 1
    $\begingroup$ Because the boundary conditions are different for the two cases. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Feb 6, 2018 at 18:51
  • $\begingroup$ What are they for the orbit instead of the box? How are they? $\endgroup$
    – Kutsubato
    Commented Feb 7, 2018 at 11:35
  • $\begingroup$ For the rigid box the boundary conditions are $\psi(0) = \psi(L) = 0$, while periodic boundary conditions require $\psi(0) = \psi(L)$ and $\frac{\partial \psi (0)}{\partial t)}= \frac{\partial \psi (L)}{\partial t}$. In each case you have two conditions, but they are different. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Feb 7, 2018 at 21:41

2 Answers 2

Reset to default
4
$\begingroup$

The "wrapping around" of the electron wave is only a heuristic argument, already made by de Broglie, to explain the discrete energy levels of an atom. For the atom, the solutions of the Schrödinger equation give you the correct wave solutions which are not related to the de Broglie wavelength and the corresponding knots in a standing wave. For example, the Schrödinger equation gives a ground state wave function of the hydrogen atom which has no knots at all.

Improve this answer
$\endgroup$
4
  • $\begingroup$ I do not get that. Aren't the solutions of the Schrödinger equation matter waves? What do they represent if they are not equal to what de-Broglie described, e.g. via $$\lambda =\frac{h}{p}$$ and aren't the solutions of the Schrödinger equation made for electrons (and not entire atoms) as well? $\endgroup$
    – Kutsubato
    Commented Feb 6, 2018 at 8:24
  • $\begingroup$ @Kutsubato - The solutions of the Schrödinger equations are de Broglie waves, i.e., one-dimensional plane waves $$\psi=\psi_0 \exp{ i(\vec k \vec r -\omega t)}$$ with the de Broglie wavelength $\lambda=h/p=2\pi/k$ only in the case of a spatially and temporally constant (zero) potential. Colloquially, also the solutions of the Schrödinger equation, the wave functions, are sometimes called "matter waves". $\endgroup$
    – freecharly
    Commented Feb 6, 2018 at 13:02
  • $\begingroup$ So the matter waves in de Broglie's theory with the de Broglie wave length are the sames matter waves as described in Schrödingers equation for a spatially and temporally constant potential that has to be zero? That is fullfilled by the particle in a box theorem, right? And the point where I was wrong is that the Schrödinger equation and its solutions are a general solution for different potentials, right? So when is the de Broglie wave length valid? Is the potential constant in multiple dimensions on an atom orbit? $\endgroup$
    – Kutsubato
    Commented Feb 6, 2018 at 15:36
  • $\begingroup$ @Kutsubato - Yes they are the same. A constant potential can always set to be zero. And for a particle in the box, you can use the plane wave solutions that are de Broglie waves. The Schrödinger equation gives you the wave solutions for a spatially dependent potential. The de Broglie wave length is only valid for wave functions in a spatially constant potential. Although the potential is constant in an hydrogen atom for a constant radius, there is no solution of the Schrödinger equation that is a Broglie wave "bending around". $\endgroup$
    – freecharly
    Commented Feb 6, 2018 at 16:49
-2
$\begingroup$

as we know the electron revolve around nucleus in closed orbit. so the whole system is behave like bound system. Electrons will come out from the orbit and become free electron if and only if it gain extra energy. same situation is there in particle in one dimension box, in which particle is allowed to move within the box only. also there is a tunneling effect in which, there is probability of particle to come out from the box after 10^24 time collision with the walls of the box. The behavior of orbital electron and particle in box is comparatively same and one use this analogy to understand behavior of electron inside the atom.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.