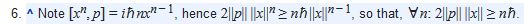According to Stone-von Neumann theorem, any two canonically conjugate self adjoint operators following the relation: $$[\hat{q},\hat{p}]=i\hbar$$ cannot be both bounded. I am confused about how we prove this part and what does it mean physically? Can anyone explain?
3 Answers
I commented that the Stone-von Neumann theorem is not a proof for the statement in the beginning of the question. The original proofs of the Wielandt-Wintner theorem (incidentally proved only in 1947-1948, while the Stone-von Neumann theorem had a satisfying proof by von Neumann already by 1931) are found in:
Wintner, A. - The Unboundness of Quantum-Mechanical Matrices (1947, The Physical Review, Vol. 71, p. 738-739)
Wielandt, H. - Über die Unbeschränktheit der Operatoren der Quantenmechanik (1948, Mathematische Annalen, p. 21).
The essence of Wielandt's proof is note 6 of the quoted Wiki page:
The significance of having unbounded operators of coordinate and momentum on the real axis (1D) is that the particle's "quantum motion" is unrestrained, in the sense that either the coordinate or the momentum can be measured to an arbitrary high value (infinite in the limit), i.e. mathematically, unbounded operators do not have a bounded spectrum.
-
$\begingroup$ Okay, but what happens if we apply same approach to energy-time uncertainty relation, since we can find the energy? We say that time operator doesn't exist as the Hamiltonian is bounded from below, but why? $\endgroup$ Commented Jan 10, 2018 at 4:40
-
$\begingroup$ This is the subject of one of the myths of QM, the so-called Pauli theorem. I will get back on this topic. It is superficially addressed by Nikolic here: arxiv.org/abs/quant-ph/0609163 $\endgroup$– DanielCCommented Jan 10, 2018 at 16:12
-
$\begingroup$ Frankly sir, Some points in the given paper doesn't seem correct, i think its quite unreliable because it tries to present everything with certainty. $\endgroup$ Commented Jan 10, 2018 at 17:09
-
$\begingroup$ I know it is partly incorrect but Found.Phys. has a weak peer-review process, so that sometimes even junk gets published. $\endgroup$– DanielCCommented Jan 10, 2018 at 21:25
-
$\begingroup$ Your supplementary questions in the comments can be linked to this question: physics.stackexchange.com/q/376822. Check my answer there. $\endgroup$– DanielCCommented Jan 10, 2018 at 23:07
Theorem: If two (not necessarily self-adjoint) bounded operators $\hat{q}$ and $\hat{p}$ on a Hilbert space satisfy the CCR $$[\hat{q},\hat{p}]~=~i\hbar~{\bf 1}, \qquad i\hbar~\in~\mathbb{C},\tag{1}$$ then $i\hbar=0$.
Indirect proof: (This is essentially the proof of Ref. 1.) Assume $$i\hbar~\neq~ 0.\tag{2}$$ Since $\hat{p}$ is bounded, we may shift $$\hat{p}^{\prime}~:=~\hat{p}+ b{\bf 1}\tag{3}$$ by a finite positive amount $b>0$, so that $\hat{p}^{\prime}$ is an invertible operator, and so that $\hat{p}^{\prime}$ and $\hat{p}^{\prime -1}$ are both bounded operators. Note that the primed operator $\hat{p}^{\prime}$ also satisfies the CCR (1). Let us drop the prime notation from now on. The spectra $$\sigma(\hat{q}\hat{p})~=~\sigma(\hat{p}\hat{q}\hat{p}\hat{p}^{-1})~=~\sigma(\hat{p}\hat{q})\tag{4} $$ of the bounded operators $\hat{q}\hat{p}$ and $\hat{p}\hat{q}$ must be equal bounded sets. On the other hand, the CCR (1) shows that the spectra are shifted $$\sigma(\hat{q}\hat{p})~\stackrel{(1)}{=}~\sigma(\hat{p}\hat{q}) +i\hbar\tag{5}$$ The only way that the eqs. (2), (4) & (5) could be not mutually contradictory is if the spectra are the empty sets. However, this contradicts the general fact (mentioned on e.g. Wikipedia and MO.SE) that
Fact: Every bounded operator has a non-empty spectrum.
$\Box$
Remark: If we additionally assume that $\hat{q}$ and $\hat{p}$ are self-adjoint, we do not need to use the above fact. Then the commutator (1) is anti-selfadjoint, so that $\hbar\in\mathbb{R}$ must be real. Moreover the bounded operator $$ \hat{s}~:=~\hat{q}\hat{p}-\frac{i\hbar}{2}~\stackrel{(1)}{=}~\hat{p}\hat{q} +\frac{i\hbar}{2}~=~\hat{s}^{\dagger}, \tag{6}$$ is selfadjoint, and hence (from the spectral theorem) has a non-empty real spectrum $$ \emptyset~\neq~\sigma(\hat{s})~\stackrel{(6)}{=}~\sigma(\hat{q}\hat{p})-\frac{i\hbar}{2}~\stackrel{(1)}{=}~\sigma(\hat{p}\hat{q}) +\frac{i\hbar}{2}, \tag{7}$$ which is eq. (5) without the loophole of empty sets. $\Box$
References:
- A. Wintner, Phys. Rev. 71 (1947) 738.
-
$\begingroup$ Can you tell me what would have been the result when operator was applied on translated state if it was indeed bounded? $\endgroup$ Commented Jan 10, 2018 at 4:37
-
$\begingroup$ Sir, I am an undergrad who just started studying quantum mechanics, and i am still confused as to how we can say from this that the operator must not be bounded? $\endgroup$ Commented Jan 10, 2018 at 16:38
-
$\begingroup$ This proof is wrong on so many levels. You cannot cherry-pick a normalized eigenstate. This is not guaranteed to exist, just because the operator is self-adjoint and bounded (the coordinate operator for the particle in a finite box is an example). (5) is nonsensical, because the operator norm is the supremum of all q's, so q' cannot be bigger than itself! Actually, (3) is wrong, for the norm of (by absurd existing)$|q'\rangle$) is equal to the norm of $|q\rangle$, because the complex exponential of a self-adjoint operator is an isometric operator!! $\endgroup$– DanielCCommented Jan 10, 2018 at 21:43
-
$\begingroup$ Ups, the theorem that I'm thinking of seems to only work with an additional assumption that the operator is compact. Updated answer with new proof. $\endgroup$– Qmechanic ♦Commented Jan 10, 2018 at 22:48
-
$\begingroup$ @Qmechanic. The new proof is also wrong. Where does (4) come from? The first equality is true, iff p is unitary (bounded and isometric), but p was assumed to be self-adjoint. The only unitary & self-adjoint operator is the unit operator. If $ \hat p = \hat 1$, then the CCR makes no sense. $\endgroup$– DanielCCommented Jan 11, 2018 at 23:45
Conjugate variables/operators are related by Fourier transform, that is, the (quantum) states of one observable are the Fourier transform of the other's and as such, only one of them can have a compact support (unless it's a zero function). This is known as the Uncertainty relation in Fourier transforms. Intuitively, it means the spread of a variable and its Fourier dual are inversely proportional, which physically translates into e.g. the position being localized (concentrated) and the momentum delocalized (spread out). For a proof approach see Qmechanic's answer.
Physically, all such types of variables/observables are incompatible (non-commuting $XP - PX \neq 0$, where $P \propto F^{-1} X F$ with $F: L^2(\mathbb{R}) \to L^2(\mathbb{R})$ ), as in they cannot be measured simultaneously to arbitrary precision. In other words, the uncertainties in the two variables are always bounded by the average of their commutator (even if you made the measurements separately on an ensemble of infinitely many identically prepared quantum systems). These uncertainties are an intrinsic property of any quantum state.
-
1$\begingroup$ What is the Fourier transform of an operator? The operator $\exp(-X^2)$ is bounded. What is its FT? is it $\exp(-P^2)$? If so, it is bounded too, contradicting your claim. $\endgroup$ Commented Jan 9, 2018 at 21:33
-
$\begingroup$ Can you explain this in terms of energy and time, since they are also canonically conjugate, they also follow an uncertainty principle, but energy can be calculated? $\endgroup$ Commented Jan 10, 2018 at 4:41
-
$\begingroup$ @HarshdeepSingh that's a somewhat special type of uncertainty relation, but it is well-discussed on physics SE, see e.g.,: physics.stackexchange.com/questions/53802/… $\endgroup$– EllieCommented Jan 11, 2018 at 14:39
-
$\begingroup$ @AccidentalFourierTransform Of course, $FT$'s a unitary operator and if you conjugate any bounded operator with it, it should remain bounded. Say for position and momentum, position operator conjugated with $F,$ i.e. $p\propto F^{-1}xF$ with $F$ here the $L^2$ normalized FT, gives you the momentum operator. On the other hand, for what concerns the uncertainty relation in QM, say again for position and momentum, the Fourier transform is applied to the quantum states themselves (and then the compactness discussion follows), the uncertainty in terms of operators is expressed as the CCR. $\endgroup$– EllieCommented Jan 11, 2018 at 21:30
-
$\begingroup$ @AccidentalFourierTransform Additionally, I've slightly rephrased things to avoid any confusion. $\endgroup$– EllieCommented Jan 11, 2018 at 21:42