Is it simply an assumption that spacetime fills the region under the event horizon?

Question

For the black-hole solution of the Schwarzschild metric to be at all possible would require spacetime to fill the region under the event horizon. Is this requirement just an unspoken or unexamined assumption or is there some mathematical or theoretical reason that it must be true?

Given the AMPS paradox with its postulation of a firewall at the horizon (space and time seem to "somehow" end there), recent LIGO data tentatively indicating signs of firewalls or other exotic physics at the horizon and some versions of string theory predicting a structure there, I'm left wondering if such an assumption (if that's all it is) is justified.

Answer 1

You can take Minkowski space and remove all points with $t\ge 0$ (for some Minkowski coordinate time $t$). Then what you have left is a perfectly well-behaved manifold that is a solution to the Einstein field equations. I suppose it could be considered an "assumption" that time will not just end at some arbitrarily chosen time $t=0$, but there is no clear reason to worry about the assumption, because $t=0$ isn't special.

The same logic applies to the event horizon of a black hole. There is nothing special about the event horizon. It doesn't have unusually high curvature or any other unusual properties. The only reason we single it out is because it has certain light-cone relationships with respect to distant regions of spacetime (such as the singularity and null infinity).

It's true that semiclassical gravity tends to predict that crazy stuff happens at the event horizon of a black hole. This is a reason to be very skeptical about all predictions of semiclassical gravity. Note that no prediction of semiclassical gravity has ever been verified, although some of its predictions have been falsified. When it makes obviously false predictions, its practitioners try to fix up the theory by doing renormalizations.

Answer 2

We don't yet understand what goes on behind the horizon; it is a topic of active research. The reason to expect spacetime to exist behind the horizon is classical: we can solve Einstein's equations and find the Schwarzschild solution, which extends smoothly across the horizon. This is the classical picture, and we can ask whether quantum corrections can change the answer drastically. One can argue that large quantum effects will show up when the curvature is large, and that for large black holes the curvature at the horizon is small. According to this line of reasoning one expects the classical picture to hold behind the horizon (as long as we don't come too near to the singularity, where the curvature blows up). This is the textbook argument for why there should be spacetime behind the horizon.

However, this argument may be too naive, and perhaps quantum effects can be large even if the curvature is small. This was suggested for example by AMPS. We do not yet know whether AMPS implies there is a firewall, or whether some loop hole (like complementarity) avoids this conclusion. (There are also other reasons besides AMPS to be skeptical of the classical answer.) It may even be that the answer depends on the precise quantum state of the black hole, namely that for some states (like the eternal black hole / thermofield double) there is a smooth interior, while for other states there is a firewall.

Answer 3

From the naive effective field theory perspective, one expects the classical spacetime to be the scenario where a bunch of particles live (photons, gravitons, electrons, ...). This is the semiclassical gravity picture, and it is assumed to be some low energy limit of quantum gravity. Note that these particles can backreact in the geometry, as long as the backreaction is small, through their interaction with gravitons. Thus, one expects the Equivalence Principle to hold, and this means that the horizon is not a special place locally, implying smoothness of the geometry.

Although semiclassical gravity (+ the Equivalence Principle) encodes Hawking's calculation of a black hole radiating thermodynamically, people believe that this effective description cannot capture some very important effects of the whole theory, such as very tiny corrections to the density matrix that make information to be conserved or even the counting of microstates of a stable black hole.

Nonetheless, and even though it is true that the authors you mention argue against the smoothness of the horizon (it is still an open theoretical problem), there are some very reasonable theoretical arguments in the context of holography that go against the AMPS and other proposals, at least within the context of typical black hole states that reach equilibrium. One of the most famous ones is the Papadodimas-Raju proposal, which shows how one can define the black hole interior with a smooth horizon, using some new state-dependent operators.

It would be very nice if we had any experimental hint regarding this problem, and although I doubt we can see if there is a firewall or not using LIGO data, it would be quite amazing if someone could actually extract some information from it.

Answer 4

Q: "For the black-hole solution of the Schwarzschild metric to be at all possible would require spacetime to fill the region under the event horizon. Is this requirement ...".

It would not "fill" the region, it must be slightly smaller to be a black hole.

For example: The Earth's Schwarzschild radius is 8.87 mm and the Sun's is ~2.95 km; because those objects are larger they are not black holes, if they were almost exactly equal they would be a black hole until they increased their diameter beyond the $r_s$, if they were smaller they would be black holes (the escape velocity is greater than $c$). Small black holes are therefore much more dense than large ones.

Singularities and black holes

The Schwarzschild solution appears to have singularities at $r = 0$ and $r = r_s$; some of the metric components "blow up" at these radii. Since the Schwarzschild metric is only expected to be valid for radii larger than the radius $R$ of the gravitating body, there is no problem as long as $R > r_s$. For ordinary stars and planets this is always the case. For example, the radius of the Sun is approximately 700000 km, while its Schwarzschild radius is only 3 km.

The singularity at $r = r_s$ divides the Schwarzschild coordinates in two disconnected patches.

The exterior Schwarzschild solution with $r > r_s$ is the one that is related to the gravitational fields of stars and planets. The interior Schwarzschild solution with $0 ≤ r < rs$, which contains the singularity at $r = 0$, is completely separated from the outer patch by the singularity at $r = r_s$. The Schwarzschild coordinates therefore give no physical connection between the two patches, which may be viewed as separate solutions.

The singularity at $r = r_s$ is an illusion however; it is an instance of what is called a coordinate singularity. As the name implies, the singularity arises from a bad choice of coordinates or coordinate conditions.

When changing to a different coordinate system (for example Lemaitre coordinates, Eddington–Finkelstein coordinates, Kruskal–Szekeres coordinates, Novikov coordinates, or Gullstrand–Painlevé coordinates) the metric becomes regular at $r = r_s$ and can extend the external patch to values of $r$ smaller than $r_s$. Using a different coordinate transformation one can then relate the extended external patch to the inner patch.

When choosing coordinate conditions, it is important to beware of illusions or artifacts that can be created by that choice. For example, the Schwarzschild metric may include an apparent singularity at a surface that is separate from the point-source, but that singularity is merely an artifact of the choice of coordinate conditions, rather than arising from actual physical reality.

A coordinate singularity occurs when an apparent singularity or discontinuity occurs in one coordinate frame, which can be removed by choosing a different frame.

Both the Schwarzschild metric and the AMPS firewall fail to consider the ergosphere, the horizon caused by the rotation of the black hole. The Kerr metric and the Kerr–Newman metric are believed to be representative of all rotating black hole solutions, in the exterior region.

The ergosphere touches the event horizon at the poles of a rotating black hole and extends to a greater radius at the equator. With a low spin of the central mass the shape of the ergosphere can be approximated by an oblate spheroid, while with higher spins it resembles a pumpkin-shape. The equatorial (maximum) radius of an ergosphere corresponds to the Schwarzschild radius of a non-rotating black hole; the polar (minimum) radius can be as little as half the Schwarzschild radius (the radius of a non-rotating black hole) in the case that the black hole is rotating maximally (at higher rotation rates the black hole could not have formed).

As a black hole rotates, it twists spacetime in the direction of the rotation at a speed that decreases with distance from the event horizon. This process is known as the Lense-Thirring effect or frame-dragging. Because of this dragging effect, an object within the ergosphere cannot appear stationary with respect to an outside observer at a great distance unless that object was to move at faster than the speed of light (an impossibility) with respect to the local spacetime.

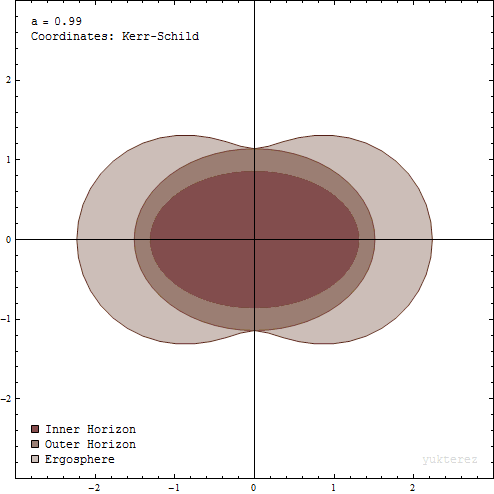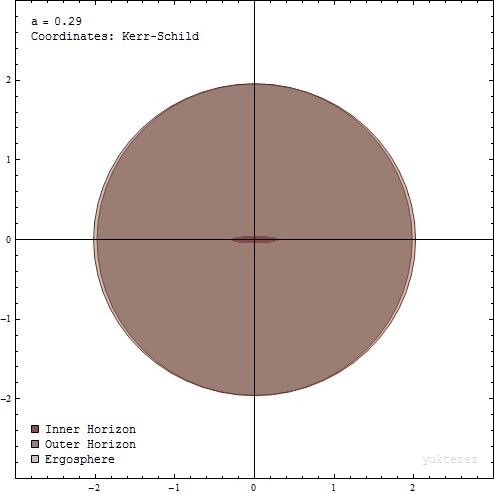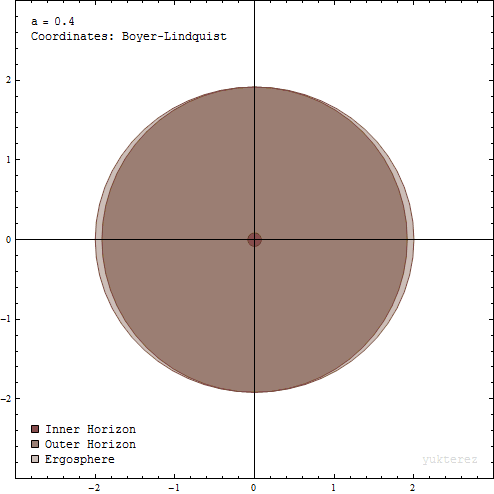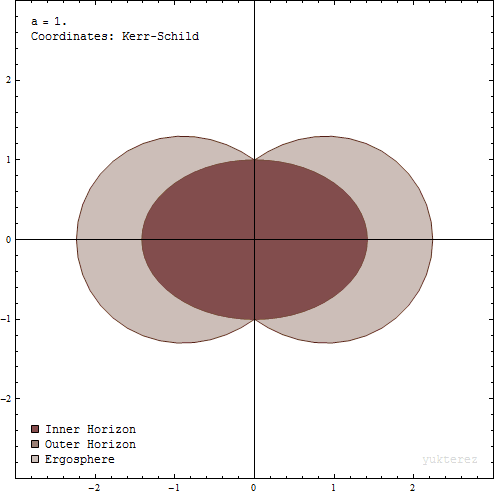
There's a chance of a non-rotating black hole occurring, and one where the region under the event horizon is filled, it's also reasonable that it is an unusual case. Usually the object would be smaller than $r_s$ and rotate due to in-falling matter.