Photoelectric effect absorption coefficient decreases with energy, why?

Question

Consider the diagram below: 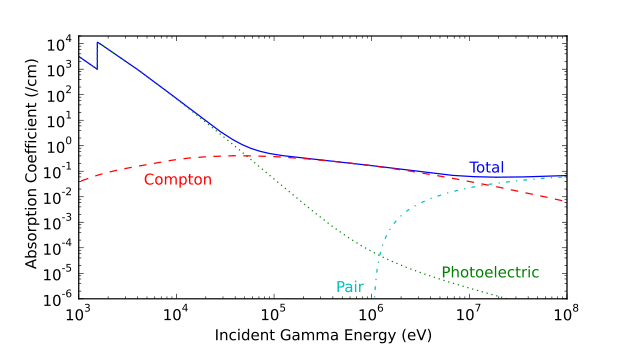(Author: Joshua Hykes source: Wikipedia)

From this diagram we can see that the absorption coefficient for the photoelectric effect generically decreases with the increase in energy of the photon. What is the physical reason for this decrease. (i.e. why does the probability of photoelectric absorption decreases with increasing photon energy).

Note: I am aware that the large spin is due to more inner electrons been able to be lifted.

Edit For Clarity

I will here rephrase the question: in the above diagram there is a general decrease in the absorption coefficient due to the photoelectric effect (i.e. the absorption of a photon by an electron and subsequent emission of that electron) with energy (ignoring the occasional absorption edge). This is of course linked to a decrease in the probability of the photoelectric effect occurring (and a corresponding decrease in the cross section). My question is: what is causing this decrease with increasing energy. (For the purpose of this question I am ignoring the contribution due to the Compton effect and pair production and simply focusing on the cross section due to the photoelectric effect.)

Answer 1

This is in the x-ray region and beyond. The wavelength of the light is smaller than the size of the electron orbitals, and decreasing when the photon energy goes up. When the electric field oscillates a lot on the length scale of the wave function, positive and negative contributions to the integrals in the transition to the excited state (the photoabsorption cross section) will cancel out.

Answer 2

The photoelectric effect (one core electron in, one photon in; one energetic free electron out, no photon out) is weaker at higher input photon energies because the output electron roughly needs to take all of the photon's momentum, but the photon does not give enough energy to do so. At lower photon energies, however, the momentum distribution of the input core electron is significant, allowing this transition more (as could be calculated rigorously with the overlap integral in Fermi's Golden Rule); you effectively see a "broadened resonance" near the ionization energy.

Your plotted energies are simply above this photoelectric resonant frequency/energy, so there is less coupling as you go to higher energies.

Answer 3

Pieter's answer is nice, +1. This is just to give a little more detail.

You can estimate the cross-section using first-order perturbation theory, and it involves $|\langle i|eEz|f \rangle|^2$, where i is the electron's initial state (bound in an atom), and f is its final state (ionized). At 10 eV, the electron's wavelength is 0.4 nm. At 100 keV, it's 4 pm. So at the higher energies, the electron's final wavefunction is an extremely rapidly oscillating sine wave, and when you take an inner product of that with the atomic wavefunction, it essentially cancels out to zero.

Of course there are a lot of other things going on, including the density of states in the final state, competition with other processes, and increases in the PE probability at K-shell edges. But I think this is the main reason for the very rapid decrease with energy.