potential energy in vertical motion.

Question

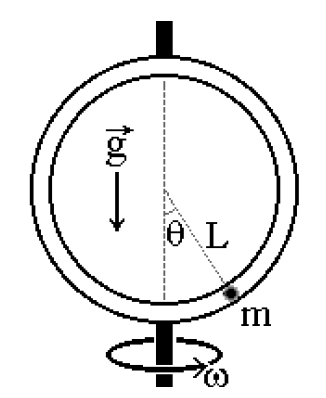
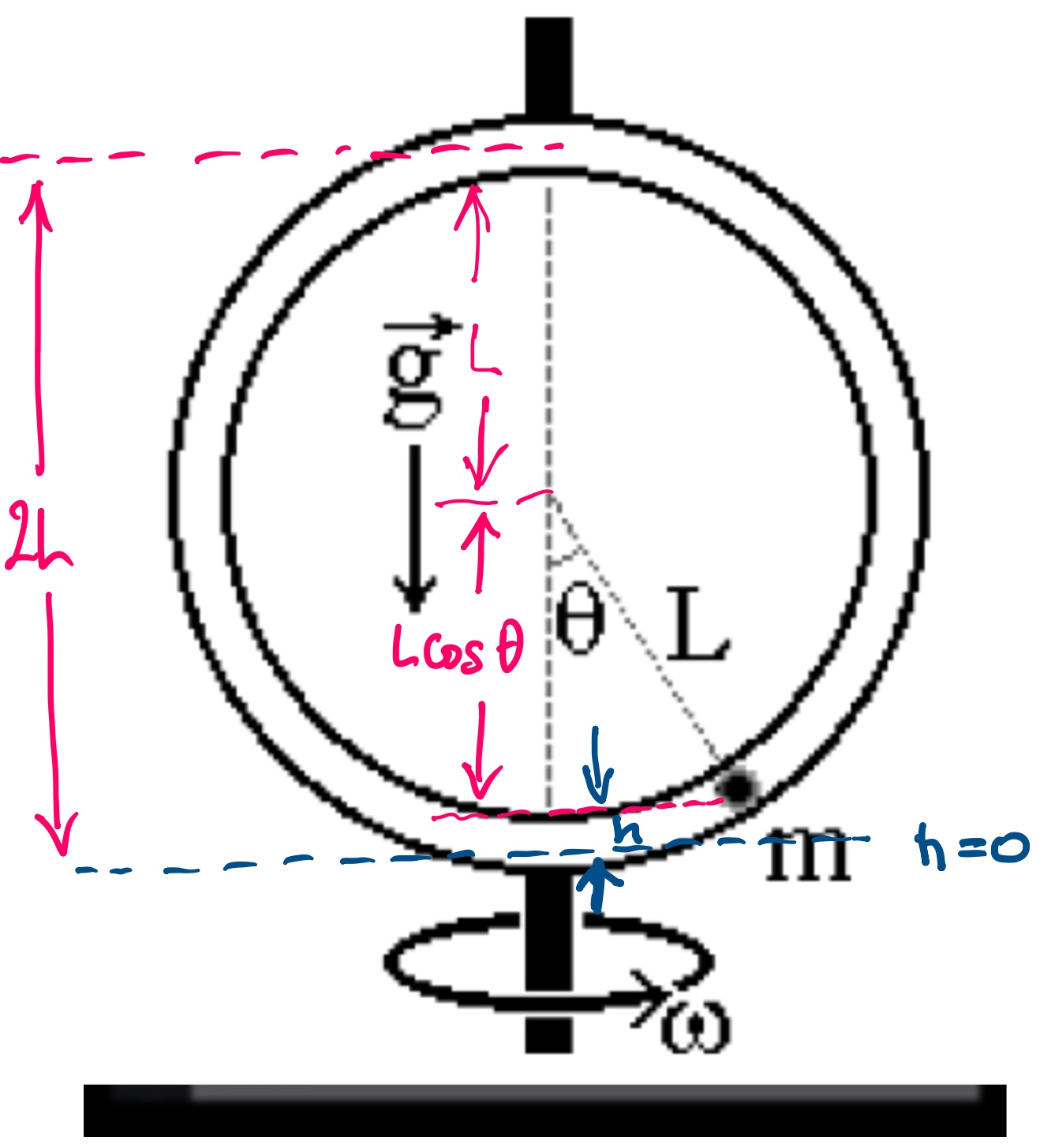
A ball of mass $m$ is moving inside a vertical hollow hoop with radius $L$ and with angular velocity $\omega$.

I was asked to find a term for the total potential energy of the ball.

Intuitively, I thought of the term $u=mgL(1-cos\theta )$ since when $\theta=0$ I expect it to be $0$ and when $\theta=180$ to be $2mgL$.

Can someone help me understand if and why this term works? how can I get to it with looking on the energy in the system?

Hint: the gravitational potential energy near Earth's surface is $U = mg(y-y_0)$, where the constant $y_0$ is chosen arbitrarily. Set up both a Cartesian coordinate grid and a polar coordinate grid that share their origin at the center of the loop. What is $y$ for a particle on the loop expressed in polar coordinates? — JM1, Commented Dec 24, 2017 at 7:42

Farcher · Accepted Answer · 2017-12-24 08:00:44Z

1

Gravitational potential energy = meh

answered Dec 24, 2017 at 8:00

Farcher

99.9k5 gold badges83 silver badges215 bronze badges

Add a comment |

1 Answer 1