Wing vibration discrete systems problem

Question

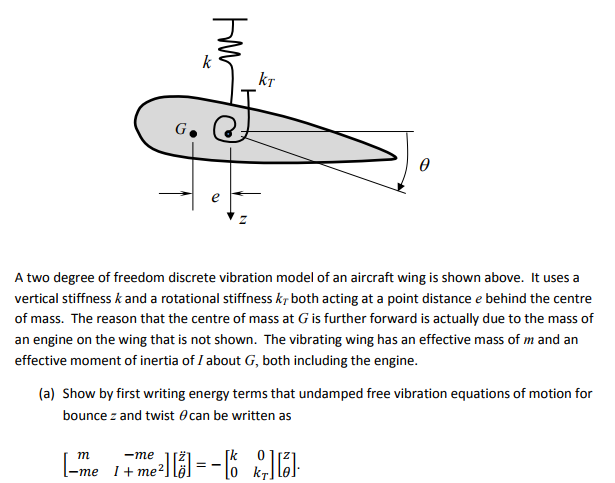
I am trying to wrap my head around the energy terms that arise from the following discrete systems vibration problem:

---

The potential energy terms seem simple enough. I include the elastic potential of the spring with stiffness $k$ and the torsional stiffness of the wing $k_T$:

$$ V = \frac{1}{2}kz^{2} + \frac{1}{2}k_T \theta^{2} $$

The kinetic energy terms of the wing is something I'm uncertain about. I visualise the wing in much the same way as a rolling wheel - I include the translational motion of the entire wing in the z direction due to the spring (down being +ve) and the rotational motion of the wing about some point to the right of $G$ a distance $e$ away. The parallel axis theorem must be used here as the rotation does not occur about the center of gravity $G$:

$$ T = \frac{1}{2}(I+me)\dot\theta^{2} + \frac{1}{2}m\dot z^{2} $$

However, my kinetic energy terms seem incomplete since the Langrange equations do not match what is provided in the mass matrix in (a). What am I missing? I thought about including the tangential velocity of the wing mass would make sense but the rotational energy of the system is already accounted for by the $\frac{1}{2}(I+me)\dot\theta^{2}$ term. What have I not taken into account?

Answer 1

For the kinetic energy, do the analysis in the center of mass frame. The key observation is that the vertical height of the center of mass is $$ z_\mathrm{cm} = z - e \sin\theta \; . $$ Thus, for small oscillations, the translation velocity is approximately $\dot{z}-e\dot{\theta}$. We can then write the total kinetic energy as the sum of translational and rotational energy: $$ T = \underbrace{\frac{1}{2} I \dot{\theta}^2}_{\text{rotation KE}} + \underbrace{\frac{1}{2} m \left( \dot{z} - e \dot{\theta} \right)^2}_{\text{translation KE}} $$ I think this gives the required answer.