What would be the total electric flux $\Phi_E$ through an infinite plane due to a point charge $q$ at a distance $d$ from the plane?
I think it should be $\frac{q}{2\epsilon_0}$, but I cannot justify that. My teacher says the flux is infinite due to the infinite area of the sheet which I cannot believe.
In general the flux through an oriented open or closed surface $\:\mathrm{S}\:$ due to a point charge $\:Q\:$ is
\begin{equation}
\Phi_{\mathrm{S}}=\dfrac{\Theta}{4\pi}\dfrac{Q}{\epsilon_{0}}
\tag{01}
\end{equation}
where $\:\Theta\:$ the solid angle by which the charge $\:Q\:$ sees the surface. In our case this solid angle is half the complete $\:4\pi\:$ solid angle, that is $\:2\pi\:$, so
\begin{equation}
\Phi_{\mathrm{S}}=\dfrac{2\pi}{4\pi}\dfrac{Q}{\epsilon_{0}}=\frac12\dfrac{Q}{\epsilon_{0}}
\tag{02}
\end{equation}
You can find special cases for the solid angles by which a point sees rectangular parallelograms in my answer therein :What is the electric field flux through the base of a cube from a point charge infinitesimally close to a vertex?.
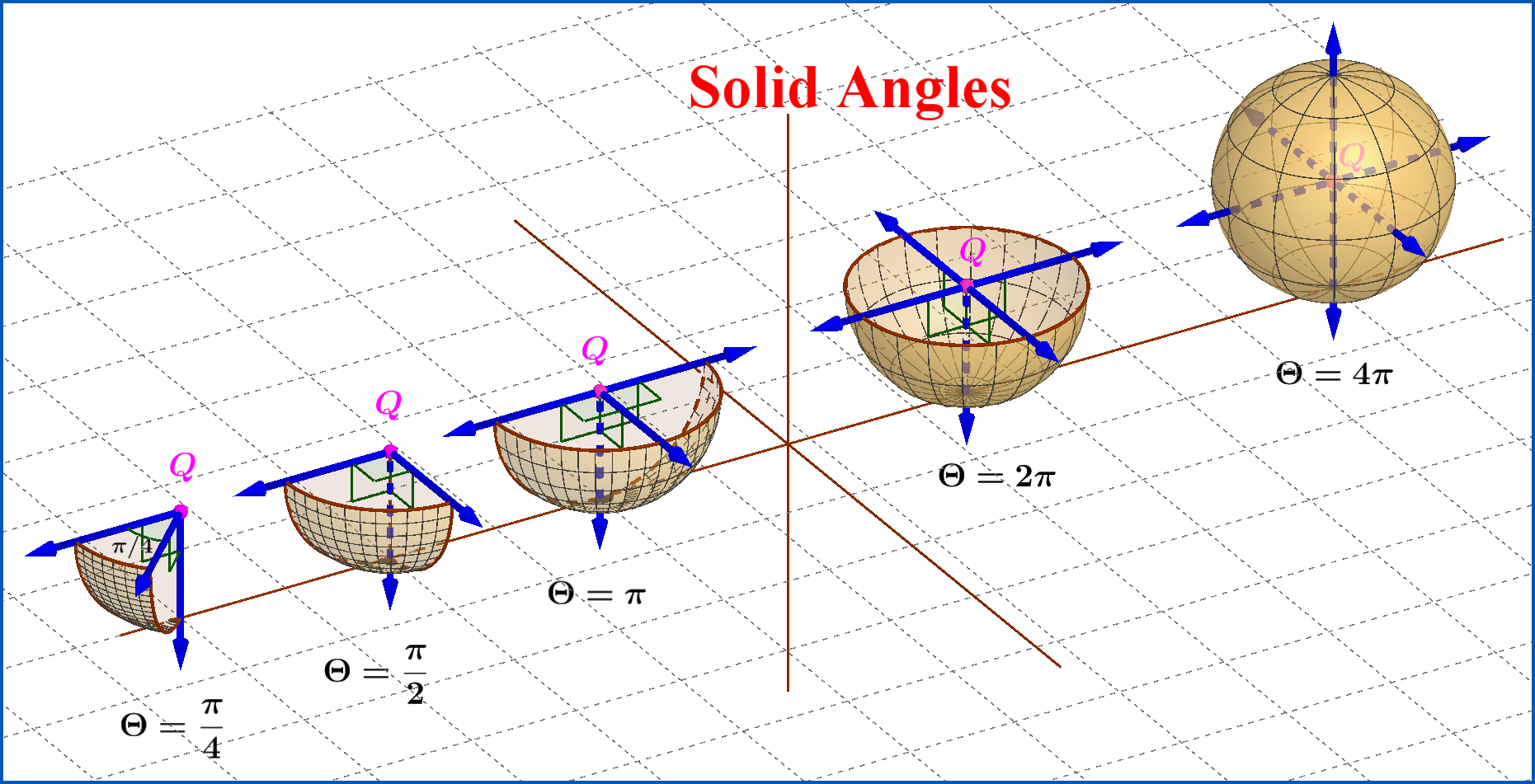
The infinite area is a red herring. The electric flux from a point charge does not measure area, because of the inverse-square dependence of the electric field itself; instead, it measures solid angle (a well-known standard fact of electromagnetism), and this is bounded above by $4\pi$, so no regular surface can accumulate infinite flux from a point charge.
If you want, you can show this explicitly through direct integration: putting the charge at $(0,0,d)$ and the plane in the $xy$ plane integrated through polar coordinates, the flux is given by \begin{align} \Phi & = \iint \mathbf E(\mathbf r)\cdot\hat{\mathbf z}\:\mathrm dS \\ & = \int_0^\infty\int_0^{2\pi} \frac{q}{4\pi\epsilon_0}\frac{r\hat{\mathbf r}-d\hat{\mathbf z}}{(r^2+d^2)^{3/2}}\cdot\hat{\mathbf z}\:r\:\mathrm d\theta \:\mathrm dr \\ & = -\frac{qd}{4\pi\epsilon_0}\int_0^\infty\frac{r}{(r^2+d^2)^{3/2}}\mathrm dr , \end{align} which is easily seen to converge (and which can, moreover, be integrated explicitly with the substitution $r=d\tan(\phi)$ to give the primitive $d/(r^2+d^2)^{1/2}$).
Consider a Gaussian surface in the shape of an infinite cuboid with $2$ faces as infinite sheets with separation $2d$ and the other $4$ faces at infinity with the charge $Q$ at the centre of the cuboid(,i.e., at a distance of $d$ from both the infinite sheets).
Then, by Gauss’ law, if the flux through an infinite sheet due to $Q$ is $\Phi$, by symmetry, the flux through each infinite sheet comprising $2$ faces of the cuboid will be $\Phi$ and through the rest of the faces will be zero as electric field dude to $Q$ is zero at infinity:
$$2\Phi+4\times0=\frac{Q}{\epsilon_0}$$ $$\therefore\boxed{\Phi=\frac{Q}{2\epsilon_0}}$$
Consider the result for the electric flux $\Phi$ through a square sheet of side $2a$ due to a point charge $Q$ placed on its axis at a distance $d$ from its centre:
$$\Phi=\frac{Q}{\pi\epsilon_0}\sin^{-1}\left(\frac{a^2}{a^2+d^2}\right)$$
In the given question, it is clear that $a=\infty$, hence:
$$\Phi=\lim_{a\to\infty} \frac{Q}{\pi\epsilon_0}\sin^{-1}\left(\frac{a^2}{a^2+d^2}\right) $$ $$\therefore\boxed{\Phi=\frac{Q}{2\epsilon_0}}$$
I think its answer is $q/\epsilon_0$ where $\epsilon_0$ is permittivity of free space. Because when flux through gaussian surface enclosing charge $q$ is $q/\epsilon_0$ and flux through any body near to this charge like plane in this case will be of course $q/\epsilon_0$.
