1
$\begingroup$

If I have an infinite plane charged sheet with a uniform charge density $\sigma$ and I want to know the electric field at a point $P$ at a distance $\vec r$ away from the sheet, how would I do that?

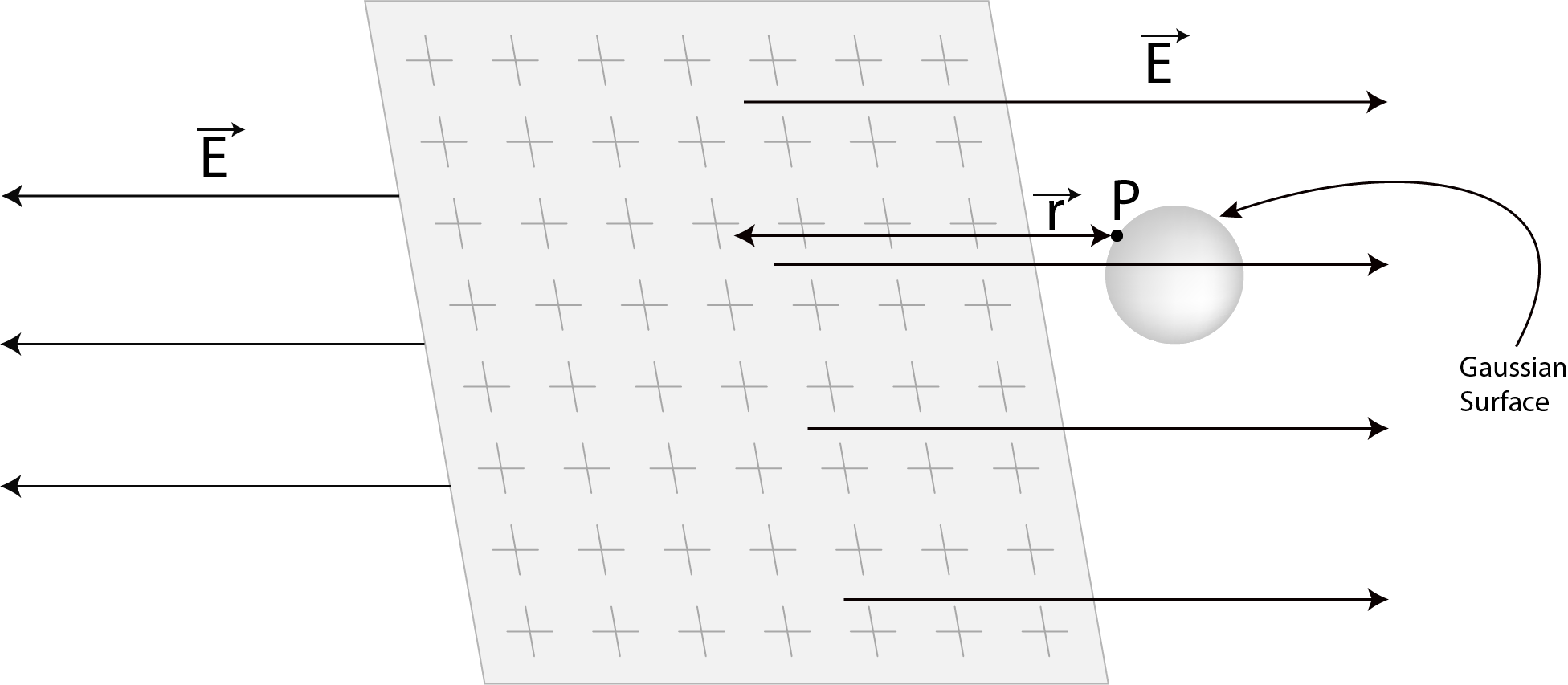
An infinite charged positive sheet with

I approach it like this knowing that this is completely wrong:

I take that point $P$ and draw a Gaussian surface (a sphere in the image) that passes through that point. And since the charge enclosed by the surface is zero, I conclude that the net flux $\phi_E$ through this surface is also zero. And since the net flux $\phi_E$ is zero, the field $\vec E$ is also zero.

Which I know is wrong but I don’t know why?

Improve this question
$\endgroup$
8
  • $\begingroup$ "And since the net flux $\phi_E$ is zero, the field $\vec E$ is also zero." This is just no true. Just look at your diagram to see an electric field entering the volume enclosed by the Gaussian surface on the left and leaving that volume through the Gaussian surface on the right. $\endgroup$
    – Farcher
    Commented Sep 25, 2017 at 11:47
  • $\begingroup$ Of course, that is true, even I know that from the diagram but then why do I end up with zero field? $\endgroup$
    – Sillysack Buttowski
    Commented Sep 25, 2017 at 12:19
  • $\begingroup$ How did you end up with the field being zero? You did not. All you ended up with was $\int \vec E \cdot d\vec A=0$ which you could expand as $\int_{\text{left area}} \vec E \cdot d\vec A=-\int_{\text{right area}} \vec E \cdot d\vec A$ which does not mean that $\vec E=0$ $\endgroup$
    – Farcher
    Commented Sep 25, 2017 at 12:25
  • $\begingroup$ How did you get $\int_{\text{left area}} \vec E \cdot d\vec A=-\int_{\text{right area}} \vec E \cdot d\vec A$ from $\int \vec E \cdot d\vec A=0$. What does that mean? Could you elaborate? $\endgroup$
    – Sillysack Buttowski
    Commented Sep 25, 2017 at 12:33
  • $\begingroup$ Look at your diagram. Imagine that for every electric field line entering your surface there is the same line leaving the surface. Gauss's law can be thought of a counting the difference between the electric field lines entering the surface and electric field lines leaving the surface. As there are no charges within your surface every electric field lines which goes in must come out. $\endgroup$
    – Farcher
    Commented Sep 25, 2017 at 12:39

2 Answers 2

Reset to default
1
$\begingroup$

Gauss' law is always true but not always useful; your example falls in the latter category. To infer the value of $\vec E$ from $\oint \vec E\cdot d\vec S$ you need a surface on which $\vert \vec E\vert $ is constant so that $$ \oint \vec E\cdot d\vec S= \oint \vert \vec E\vert \, dS = \vert \vec E\vert \oint dS = \vert \vec E\vert S \, . \tag{1} $$ $\vec E$ is not constant on your sphere, meaning you cannot use (1) and pull $\vert \vec E\vert$ out of the integral and recover $\vert\vec E\vert$ through $$ \vert \vec E\vert = \frac{q_{encl}}{4\pi\epsilon_0 S}\, . $$ In your specific example, this is why $\oint \vec E\cdot d\vec S=0$ even though $\vert \vec E\vert$ is never $0$ at any point on your Gaussian surface. The $0$ results from the geometry of $\vec E\cdot d\vec S$ everywhere on the sphere rather than $\vert \vec E\vert=0$.

To proceed you need to use a Gaussian pillbox with sides perpendicular to your sheet because, by symmetry, the field must also be perpendicular to your sheet. Thus $\vec E\cdot d\vec S$ for all sides of the pillbox is easy to compute. If your pillbox passes through the sheet, it will enclose non-zero charge and, using simple geometry, one easily shows that the flux through the back cap will add to the flux through the front cap and you can recover the usual result.

Improve this answer
$\endgroup$
7
  • $\begingroup$ "The $0$ results from the geometry of $\vec E \cdot d\vec S$ everywhere on the sphere rather than $\vert \vec E \vert = 0$." What does that mean? $\endgroup$
    – Sillysack Buttowski
    Commented Sep 25, 2017 at 13:37
  • $\begingroup$ Also, why does the field strength come out to be independent of distance? That is very nonintuitive as the field should vary according to the inverse square law. $\endgroup$
    – Sillysack Buttowski
    Commented Sep 25, 2017 at 13:40
  • $\begingroup$ $\vec E\cdot d\vec S$ varies in magnitude across the geometrical surface so that the sum (or more properly the integral) is $0$, not because $\vec E=0$. If you think about it, how does the physics change if you are $2m$ or $3m$ above the sheet? How would you know anyways since the sheet is infinite and the surface charge density constant?. $\endgroup$
    – ZeroTheHero
    Commented Sep 25, 2017 at 13:45
  • $\begingroup$ Maybe we will never be ever to verify this and I will just have to believe the math. $\endgroup$
    – Sillysack Buttowski
    Commented Sep 25, 2017 at 13:59
  • $\begingroup$ @SillysackButtowski I'm not sure what you mean by "verifying this". How can it matter if you are at 1 or 100 meter above an infinite plate? Based only on the plate itself you'd have no way of knowing your "altitude" since everything is exactly the same at all heights in all directions. $\endgroup$
    – ZeroTheHero
    Commented Sep 25, 2017 at 14:05
0
$\begingroup$

When using Gauss's theorem , you should pay attention that:

1-The Gaussian surface must be chosen in a way that the electric field passing through every point on the surface has the same magnitude and direction as the unit vectors of your coordinates. Therefore I suggest you choose a cube or a cylinder as your Gaussian surface. Otherwise, it can be difficult to solve the integral .

2- You should note that the Gaussian surface should include the charged plane as well as the given point .

3-You can have Electric field inside a Gaussian surface and zero flux at the same time . Like a point charge placed at the center of a sphere .

Improve this answer
$\endgroup$
1
  • $\begingroup$ Please elaborate #1, I don't really understand that. For #2, why should the plane be included in the Gaussian surface? For #3, wouldn't flux actually be positive or negative for a point charge at the center of a sphere. $\endgroup$
    – Sillysack Buttowski
    Commented Sep 25, 2017 at 12:24

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.