0
$\begingroup$

Torque, $\tau$ is defined as:

$$\vec{{\tau}} = \vec{r}\times\vec{F}$$

Intuitively, it's the rotational effect of force. However, using the above definition of torque we can calculate the torque due to a force acting on any particle about a reference point.

Consider projectile motion for instance. A particle was projected at an angle $\theta$ with a speed $u$. I calculated the torque due to weight at the highest point of the particle's trajectory about the point of projection to be $-mu^2\sin^2\theta$.

The thing that confuses me is that here there's torque but no rotation. What is the purpose of torque in projectile motion then?

Improve this question
$\endgroup$
1
  • $\begingroup$ The angular position of the projectile with respect to the selected center around which rotation is measured changes, so there is 'rotation', and the question is founded on an misapprehension. Not that there is any point in working the problem in that way unless you are about to generalize to orbital motion but it is an internally consistent way to approach the problem. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Sep 25, 2017 at 15:54

1 Answer 1

Reset to default
2
$\begingroup$

The equations that you have obtained for the trajectory of a projectile can be obtained by considering the torque which is acting on a mass $m$ and equating the torque to the rate of change of angular momentum.

Consider a mass which is projected at an angle $\theta$ to the horizontal from location $O$ at position $\vec r_{\rm O} = 0 \hat i+0 \hat j$ with velocity $\vec v_0 = \dot x_0 \hat i + \dot y_0 \hat j$ at time $t=0$.

At time $t$ the new position of the mass is $\vec r = x \hat i + y \hat j$ and it has a velocity $\vec v = \dot x \hat i + \dot y \hat j$.

The constant force acting on the mass is $-mg \hat j$.

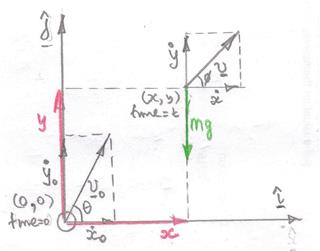
enter image description here

At time $t$ the torque acting on the mass about location $O$ is

$\tau_{\rm O} = (x \hat i + y \hat j) \times (-mg\hat j) = -mgx\,\hat k$

where $\hat k$ is the unit vector coming out of the screen.

At time $t$ the angular momentum of the mass about location $O$ is

$L_{\rm O} = (x \hat i + y \hat j) \times m(\dot x \hat i + \dot y \hat j) = m(x \dot y - \dot x y)\, \hat z \Rightarrow \dfrac{d\vec L_{\rm O}}{dt} = m(x \ddot y - \ddot xy)\,\hat z$

$\vec \tau_{\rm O} = \dfrac{d\vec L_{\rm O}}{dt} \Rightarrow -gx = x \ddot y - \ddot xy$.

As the last equation must be true for all $x$ and $y$ this means that

$\ddot x =0$ and $\ddot y = -g$

which are the equations that you get by applying Newton's second law to the horizontal and vertical motions of a projectile.

In this case you could say that the torque has caused a change of direction, "rotation", of the velocity vector or you could say that the position vector $\vec r$ has been "rotated" but the key idea is that the torque has changed the angular momentum.

Improve this answer
$\endgroup$
7
  • $\begingroup$ 4Qer, where is the unit vector k? And how did you get -gx in the next line? $\endgroup$
    – LÜHECCHEgon
    Commented Jun 10, 2023 at 1:58
  • $\begingroup$ How did you deduce 𝑚(𝑥𝑦˙−𝑥˙𝑦)𝑧̂ $\endgroup$
    – LÜHECCHEgon
    Commented Jun 10, 2023 at 2:54
  • $\begingroup$ Cross product $(\hat i + hat j)\times (-\hat j) = - \hat k$ and differentiate with tespect to time $\frac {d}{dt}(x\dot y-\dot x y) = \dot x \dot y +x\ddot y -\ddot x y -\dot y \dot x $. $\endgroup$
    – Farcher
    Commented Jun 10, 2023 at 7:08
  • $\begingroup$ Where did u get the intuition for 𝑥𝑦˙−𝑥˙𝑦? $\endgroup$
    – LÜHECCHEgon
    Commented Jun 10, 2023 at 7:26
  • $\begingroup$ $\vec L_{\rm O} = \vec r \times m\vec {\dot r} =(x \hat i + y \hat j) \times m(\dot x \hat i + \dot y \hat j) = m(x \dot y - \dot x y)\, \hat z $. $\endgroup$
    – Farcher
    Commented Jun 10, 2023 at 7:29

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.