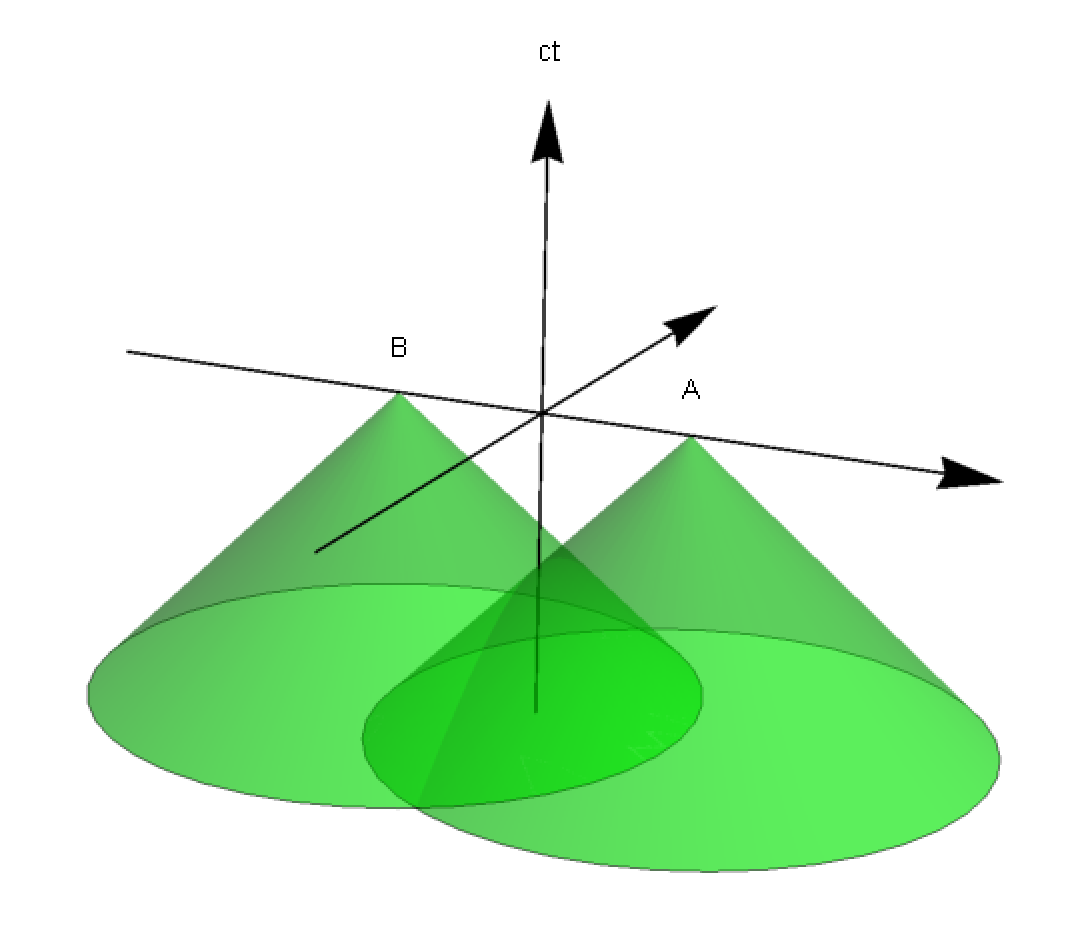
If I, an inertial observer, see an event reach two points, A and B, at time t=0, what does the surface of possible source points in spacetime look like, assuming the event propagates at the speed of light? I think it's a hyperbolic surface in some way but having a hard time visualizing.
1 Answer
$\begingroup$
$\endgroup$
5
The constraint is that any source event must be in both $A$ and $B$'s past lightcones. Hence, the potential source is the overlap between the past lightcone from $A$, and the past lightcone from $B$.
If the event is travelling at the speed of light, then it will be on the lightcone, and hence you are looking at the intersection of the edges of the two cones. I've included a diagram for the 2d case.
-
$\begingroup$ Any idea what that surface is though? Is it hyperbolic? $\endgroup$– gctCommented Aug 30, 2017 at 22:45
-
1$\begingroup$ A hyperbola is the intersection of a plane and a cone. en.wikipedia.org/wiki/Conic_section As all intersection points do lie on a vertical plane bisecting $\vec{AB}$ then this must be a hyperbola. (It's a line not a surface, though) $\endgroup$ Commented Aug 30, 2017 at 22:51
-
$\begingroup$ I'm pretty sure the light cones are actually hyperbolic though: $x^2+y^2+z^2-(ct)^2 = 0$, so I'm curious if it still works out the same. $\endgroup$– gctCommented Aug 30, 2017 at 22:55
-
$\begingroup$ I agree on the line of intersections being a hyperbola, based on the reasoning in the comment by @JMLCarter In 3D, it becomes intersections of spheres, which would be a hyperboloid. $\endgroup$– CDCMCommented Aug 30, 2017 at 23:02
-
1$\begingroup$ Light cones are (hyper)cones, since there's a zero on the RHS of that equation. Consider the Euclidean 3D case. $x^2+y^2=z^2$. That's a pair of cones whose apices touch at the origin, symmetrical about the Z axis. $\endgroup$– PM 2RingCommented Aug 30, 2017 at 23:08