0
$\begingroup$

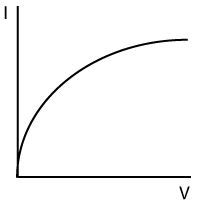
As we know from the nonlinear I-V characteristics of a filament lamp, that the resistance of a filament changes with change in current/voltage.

enter image description here

From the relation V=IR, it should imply that, say with increase in current, the resistance should decrease, but at the same time V is increasing aswell. So, how to be certain that increase in V is more than that of I, such that R increases?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

The resistance of a particular component is defined to be the applied voltage divided by the resulting current. As you can see from the plot you attached, you can continue to increase V as much as you'd like, but the resulting current appears to saturate at some constant value. Therefore, the ratio $R = V/I$ increases with increasing $V$.

Improve this answer
$\endgroup$
1
  • $\begingroup$ So, this means, when I increase $I$, $V$ will increase by more amount, resulting in an increase in current, even with the increase of current. Ah, I get it. $\endgroup$
    – AgentRock
    Commented Aug 28, 2017 at 14:45

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.