Is a static Schwarzschild observer geodesic?

Question

I know the answer to this question is no: a static observer is defined to be following the flow of the Killing vector field $\xi = \partial_t$, with appropriate normalization of the 4-velocity, such that $$ \label{1} \dot{t}=\left( 1-\frac{R_s}{r} \right)^{-1/2} = \text{const}, \, \dot{r}=0, \, \dot{\theta}=0, \, \dot{\phi}=0$$ Here $\dot{-}$ denotes derivative wrt proper time. These world lines are not geodesics: if they were freely falling, they would fall toward the center and the spatial coordinates would be functions of (proper) time; they need some kind of thrust to stay in the same point in space.

But: from Carroll, the explicit Schwarzschild geodesic equations are

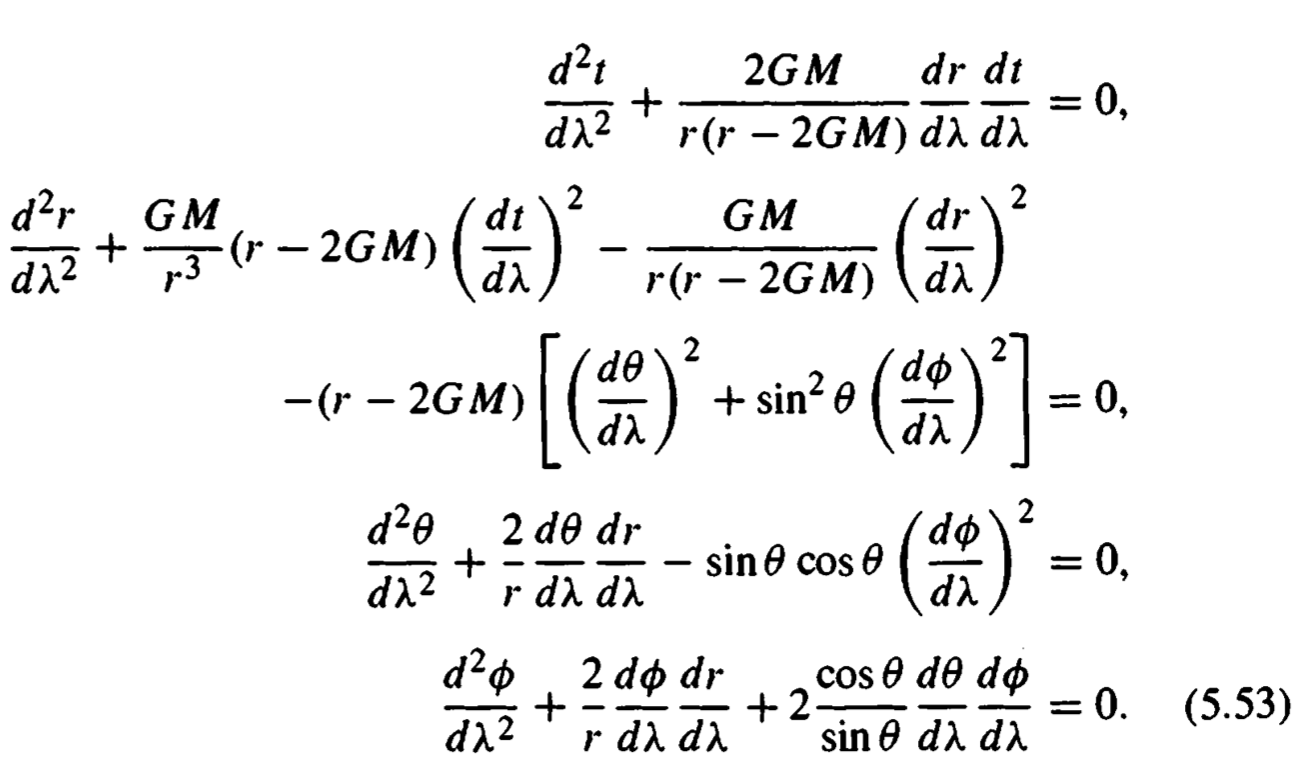
which reduce to 4 identities $0=0$, considered the above equation for $\dot{t}, \dot{r}, \dot{\theta}, \dot{\phi}$. Where is the problem?

Answer 1

You are correct in saying that, with your definition of static observer, the curve on which the observer moves is not a geodesic curve. As physically expected, a geodesic trajectory in Schwarzschild universe requires motion, around the center of attraction or directed into it, in a way that is similar to Newton's classical gravity (but with much more complicated equations).

I think you are doing a trivial error. In your definition of the four velocity, you say that $\dot t$ is the only non vanishing velocity, and it is constant at all values of the parameter that you use to describe the motion. The dot is the derivative with respect to said parameter.

This means that, in your equations, $$ \frac{dt}{d\lambda}=\left(1-\frac{R_s}{r}\right)^{-1/2}, $$ and all other terms are zero. As you can see, the second equation of motion is non vanishing (unless you stationate at r=2GM apparently, but you can't have r=2GM in Schwarzschild coordinates as the metric is singular there and you have to switch to another coordinate system). So, in general, the second equation is not solved and the curve is not geodesic.