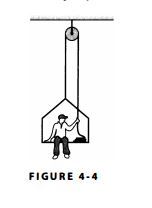
You need to think of the man and the chair separately. This is because if you combine the man and the person into one thing, you get a single equation with Newton's second law with two unknown values (the acceleration and the upwards tension forces).
The man has a tension force acting upwards on him, a normal force upwards on him, and his weight downwards. The chair has tension acting upwards, the same magnitude of a normal force downwards, and it's weight downwards. We can exploit that each of the tension forces are the same (which is something that is incorrect in your diagram)$^*$, as well as the fact that the man and chair will have the same acceleration. Therefore, by Newton's second law,
$$ma=T+N-mg$$
$$Ma=T-N-Mg$$
Where $m$ and $M$ are the masses of the person and the chair respectively.
Since you are given what $m$, $M$, and $N$ are, these are two equations with two unknown values. Note that the 100 lbs corresponds to $N$, not $T$. I will leave the math to you to find what $a$ and $T$ are.
Also, note that the sum of these equations is what we get by treating evening as one system that I was discussing at the beginning of this answer.
$$(m+M)a=2T-(m+M)g$$
Notice how we cannot get actual values for $a$ or $T$ with this equation and the given information. We need the previous two equations (or this one and just one of the previous ones) to solve the problem.
Your question title seems to be concerned with more of understanding a cause-and-effect relationship between the forces. I would say what happens is that the man pulls on the rope. This causes two things. 1) It applies an upward force to the man 2) It applies an equal upward force to the chair. Each of these two things have an opposite effect on the normal force between the person and the chair. The first lessens it, and the second makes it larger. The net effect is given in the problem as a 100 lb normal force.
It might be instructive to solve for $N$ and $a$ rather than $T$ and $a$.
$$N=\frac{T(m-M)}{m+M}$$
$$a=\frac{2T}{m+M}-g$$
As we can see, the normal force between the man and the chair is directly proportional to the force the man applies to the rope. We can explore this problem thinking of different scenarios. For example, if the man does not pull on the rope ($T=0$), then $N=0$, so the man and chair will fall with an acceleration of $-g$, as expected.
We also see that in order for the man to accelerate upwards he must apply more than half of the total weight of himself and the chair, which makes sense since the whole system is pulled upwards with a force of $2T$. In other words, he gets double out of what he puts in essentially. This also let's us see that if $a>0$, then $N>\frac{g(m-M)}{2}$, which is true in your problem. You can play around with the equations like this and learn other things about the system.
$^*$ If we assume a massless rope, and a massless, frictionless pulley, then the tension throughout the string is uniform. This is why the tension forces acting on the man and the chair are the same.