Get an idea of average velocity when you just know the distance covered

Question

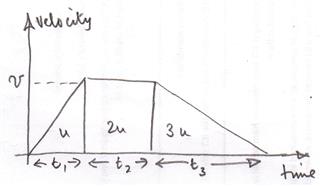
Can we calculate average velocity when we just know the distance covered? For example, when a car moves with a certain acceleration for a distance $u$ then it moves with a constant velocity for a distance $2u$ and at last it deaccelerates for a distance $3u$.

I tried to solve it by taking the intervals of time $T_0$ to $T_1$ for which the car accelerates, then $T_1$ to $T_2$ for which it moves with constant velocity and at last $T_2$ to $T_3$ for which it decelerates and finally comes to rest.

But that doesnt help. I do not have any idea about the intervals of time. So how can I compute the average velocity? Is there some other way to compute the average velocity?

Answer 1

To get the average velocity you need the change in displacement and the time taken for the change.
Unless the displacement is zero you cannot find the average velocity without a time being given or in the case of this example, the maximum velocity.

If the accelerations are constant the velocity-time graph looks like this.

As the area under the graph is the displacement so you can relate $t_1, t_2,t_3$ to one another and hence find the average velocity in terms of the maximum velocity $v$.

Answer 2

There are a number of parameters relevant to the motion:

• time taken, $t$

• initial velocity, $u$

• final velocity, $v$

• acceleration, $a$

• distance moved, $s$

These are related by the SUVAT equations:

$$ v = u + at $$

$$ s = ut + \tfrac{1}{2} at^2 $$

$$ v^2 = u^2 + 2as $$

So given values for some of the parameters we can calculate others. But in your question you specify that all we know is the distance moved, and in this case there is too little information to calculate the values of the other parameters.

Suppose we look at your specific example:

if a car moves with a certain acceleration for a distance $s$ then it moves with a constant velocity for a distance $2s$ and at last it decelerates for a distance $3u$.

In the first stage if we know that the car starts from rest and accelerates with some acceleration $a$ then we can calculate the time taken and final velocity because we know:

$$ s = ut + \tfrac{1}{2} at^2 $$

so given that $u=0$ we get:

$$ t = \sqrt{\frac{2s}{a}} $$

And the average velocity is then distance travelled divided by time taken.

So you can work out what you can calculate by considering what you know and what the SUVAT equations tell you.