0
$\begingroup$

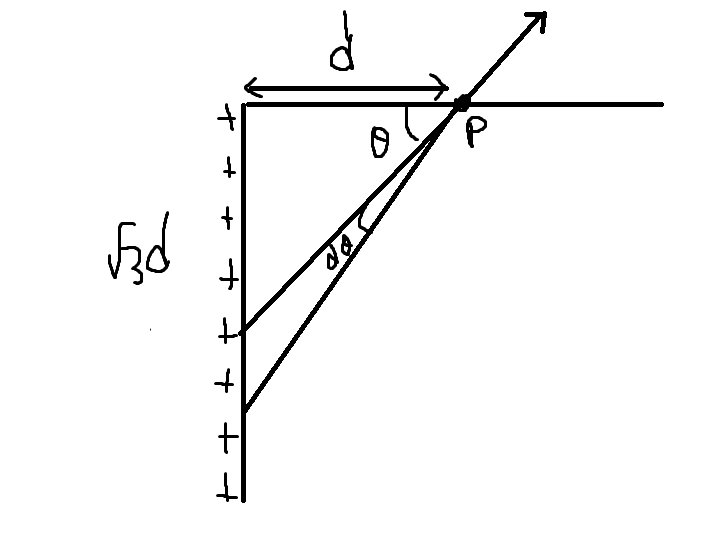
enter image description here

I have to find the direction of electric field due to this rod at point P

$dE_x =[Kdq/(d/cos\theta)^2]cos\theta$ and $dq = \lambda (d/cos\theta) d\theta$ Writing similar equations for $E_y$, then integrating these two from 0 to $pi/3$ followed by $tan^{-1}E_y/E_x$ gives me the wrong answer

I know I can get the correct answer by integrating along the length of the rod but why is using a polar system giving me the wrong answer? (I understand in this case I have chosen my intervals incorrectly since they don't even sum up to $\sqrt 3 d$).

My main question is:

Is it necessary that the intervals chosen for a Riemann sum have to be of equal size? What are the conditions/rules while choosing intervals?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
0
$\begingroup$

I think you have taken the dq component to be wrong.We know that $\frac{y}{d}=\tan \theta$.Therefore,$dy=d\times \sec^2 \theta \ d\theta$.In your method also,you have to divide dq by one $\cos \theta$ because you didn't take into account that $ld\theta$ where l is the hypotenuse in the big triangle does not give dy but gives $dy\cos \theta$ .Try to think of the geometry yourself.

Improve this answer
$\endgroup$
2
  • $\begingroup$ How could you prove that the perpendicular dropped from the "point of contact" of the hypotenuse with the wall onto the next line, intersects that line exactly where the arc $ld\theta$ does? Because only in that case will $dycos\theta$ become equivalent to $ld\theta$ in the limit $d\theta$ tending to zero $\endgroup$
    – xasthor
    Commented Jun 14, 2017 at 19:05
  • $\begingroup$ The two lines dropped from P to the wall will actually be parallel because the angle between them is infinitesimal and the arc $l d\(theta )$ is actually not an arc but a straight line perpendicular to the top line.Now, is the geometry clear?Anyways,did you try computing the answer with the correction factor? $\endgroup$
    – Rishabh Jain
    Commented Jun 14, 2017 at 19:57

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.