Why quasiparticles do not decay in finite system in random phase approximation?

Question

I have tried to apply the conventional recipe of calculating electron self-energy part $\Sigma$ in the random phase approximation (RPA) to the case of finite system and obtained $\mathrm{Im}\,\Sigma=0$, i.e. that the quasiparticle does not decay.

Details:

Let's take the formulas for the on-shell self-energy part $\Sigma(k,\xi_k)$ from G.D. Mahan, "Many-particle physics" (1993), pages 396-397:

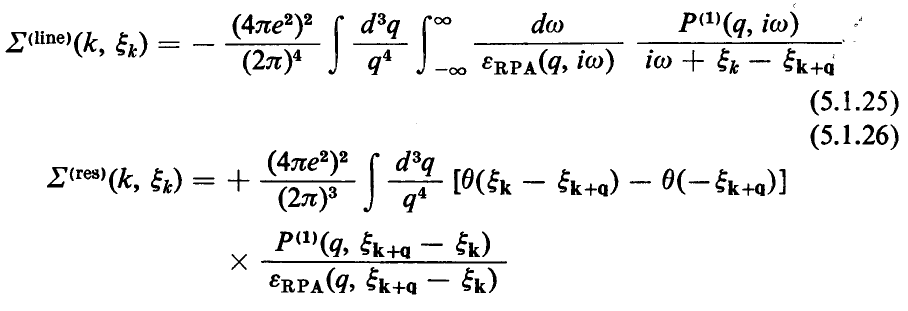Here $P^{(1)}(q,i\omega)$ is the electron gas polarizability and $\varepsilon_\mathrm{RPA}(q,i\omega)=1-v_qP^{(1)}(q,i\omega)$ is the dielectric function. As said on these pages, $\Sigma(k,\xi_k)$ is a sum of line and residue contributions, $\Sigma=\Sigma^\mathrm{(line)}+\Sigma^\mathrm{(res)}$. Moreover, $\Sigma^\mathrm{(line)}$ is a real quantity so imaginary part of $\Sigma$ comes only from $\Sigma^\mathrm{(res)}$.

What if the system is finite:

In this case the single-electron energies $\xi_k$ and $\xi_{\mathbf{k}+\mathbf{q}}$ are discrete, and the polarizability can be calculated as $$ P^{(1)}(q,i\omega)=\frac1V\sum_{\mathbf{k}}\frac{n_F(\xi_k)-n_F(\xi_{\mathbf{k}+\mathbf{q}})}{i\omega+\xi_k-\xi_{\mathbf{k}+\mathbf{q}}}. $$ As seen, $P^{(1)}(q,i\omega)$ has singularities at the discrete energies $i\omega=\xi_{\mathbf{k}+\mathbf{q}}-\xi_k$. Then, the quantities $$ \frac{P^{(1)}(q,\xi_{\mathbf{k}+\mathbf{q}}-\xi_k)}{\varepsilon_\mathrm{RPA}(q,\xi_{\mathbf{k}+\mathbf{q}}-\xi_k)}=\frac1{[P^{(1)}(q,\xi_{\mathbf{k}+\mathbf{q}}-\xi_k)]^{-1}-v_q} $$ entering $\Sigma^\mathrm{(res)}$ are just $-1/v_q$ and are purely real, because $[P^{(1)}]^{-1}\rightarrow0$ at the singularity points.

So we get: $\mathrm{Im}\,\Sigma(k,\xi_k)=\mathrm{Im}\,\Sigma^\mathrm{(res)}(k,\xi_k)=0$ which means vanishing decay rate of a quasiparticle. Is this result physically reasonable in the case of a finite system?

Answer 1

This makes sense: you can only decay into a continuum. Said differently, a complex pole would imply that the spectral weight of the decaying particle extends over a finite region of energy space. In a finite system, the spectrum always consists of a finite sum of Dirac deltas, never a continuum. So to see decay, either one should work directly in the continuum limit, or one can calculate the spectral response for a finite system and see how that approaches the continuum limit.

It is an interesting question to ask how the complex pole of the continuum limit arises as a sequence of finite system sizes. Mathematically, this should have to do with branch cuts being related to the possibility of having complex poles (at least in the context of Green's functions), and since branch cuts can be seen as a continuum of poles, it is clear that they can only arise in the thermodynamic limit of a system.

If one prefers equations, consider that a Green's function for a finite system is always of the form $$ \hat G(E) = (E-\hat H)^{-1} = \sum_n \frac{|n\rangle \langle n |}{E-E_n} $$ where the sum is finite. Hence, the analytic continuation is trivial: $$ \hat G(z) = \sum_n \frac{|n\rangle \langle n |}{z-E_n}. $$ It is trivial to see that this has no complex poles! However, if the sum is infinite, then the latter expression is no longer necessarily an analytic expression. The analytic Green's function will generically have (a) branch cut(s) along the real axis, and complex poles can exist off the real axis.