The particle moves on a circle, so its motion can be written in polar coordinates as:
$$\begin{pmatrix} x\\y
\end{pmatrix} = r \begin{pmatrix}
\cos\theta\\
\sin\theta
\end{pmatrix}$$
with constant $r$. To match the image, I chose the x-axis pointing to the left and y-axis up.
The velocity is then given by the derivative:
$$\begin{pmatrix}v_x\\v_y
\end{pmatrix}= r \dot{\theta}\begin{pmatrix}-\sin\theta\\ \cos\theta\end{pmatrix}$$
Acceleration in the x-direction is assumed to be constant ($=a_x$) which makes $v_x$ linear in time. Taking into account that $v_x(t=0)=0$ we have:
$$v_x=a_x t = -r\dot{\theta}\sin\theta$$
This equation can be readily integrated which gives you an equation for $\theta$ as a function of time:
$$a_x \int_0^t t~dt = -r\int_0^\theta\sin\theta~d\theta$$
$$\frac{a_x t^2}{2} = r (\cos\theta-1)$$
If you put this back into the equation for the velocity components, you can find the velocity as a function of time. Putting $t=\sqrt{-\frac{2 r}{a_x}}$ gives you the velocity at point B.
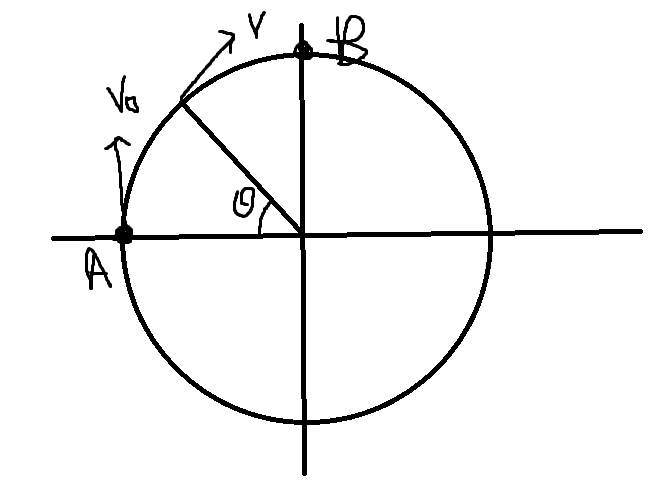 A particle moves along A->B with a constant acceleration in the x direction. I'm supposed to find the velocity at B
A particle moves along A->B with a constant acceleration in the x direction. I'm supposed to find the velocity at B