We have two particles on frictionless rails:
S1: with Mass $m_1$ and Velocity $v_1=0\frac{m}{s}$
S2: with Mass $m_2$ and Velocity $v_2$
$`m_1 >= m_2`$
So particle S2 is moving toward particle S1 which is initially at rest with a constant speed $V_2$
S1 and S2 collide: So the question is how can we choose a ratio $\frac{m_1}{m_2}$ in order to get a maximum distance L covered on the rail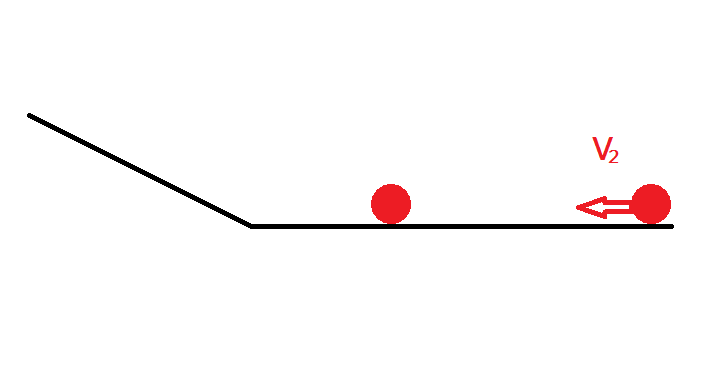
Knowing that: $L= \frac{4m_{2}^{2}V_{2}^{2}}{g(m_{1}+m_{2})^2}$
The answer is 1 but i just don't understand why?
