By looking at a diagram, how does one tell whether it represents a $s$-channel process or a $t$-channel process i.e., without finding the amplitude? I'm familiar with Mandelstam variables but I've trouble understanding what a $s$-channel or $t$-channel process would mean and how to tell the difference.
$\begingroup$
$\endgroup$
0
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
3
I think the easiest way is to check the agreement beetween fermions/antifermions and the directions of their arrows.
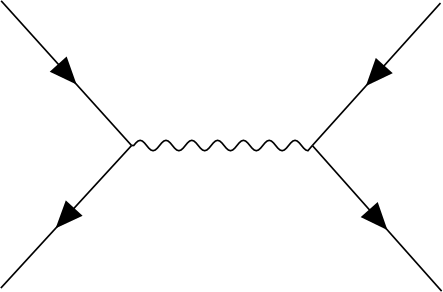
Take this for example:
You can label all the fermions $e^-$ and it's t-channel. If you label the top left and bottom right $e^-$ and the other two $e^+$, it's s-channel.
-
$\begingroup$ If the diagram represents $e^-$–$e^-$ scattering then it doesn't follow either of the usual conventions for labeling the axis. It would have time increasing down the page. $\endgroup$ Commented May 3, 2017 at 17:51
-
-
3$\begingroup$ Sure, but a reader could easily be confused by it. It's a small correction to refer to positron-positron scattering instead of electron scattering and then the example is plausible for one the common conventions. $\endgroup$ Commented May 3, 2017 at 21:17
$\begingroup$
$\endgroup$
For a two-body scattering process denoted by $$a_1(p_1)+a_2(p_2)\to a_3(p_3)+a_4(p_4)$$ the tree-level Feynman diagrams can be classified into three categories. A tree-level scattering diagram is called the $s$-channel, the $t$-channel and the $u$-channel process if the squared 4-momentum of carried by the mediator is $s=(p_1+p_2)^2$, $t=(p_1-p_3)^2$ and $u=(p_1-p_4)^2$ respectively. Thus by identifying the 4-momentum of the exchange particle, it is easy to decide whether the diagram is $s$, $t$ or $u$-channel.
Reference: Mark Thomson's lecture notes (Page 13) https://www.hep.phy.cam.ac.uk/~thomson/partIIIparticles/handouts/Handout_1_2011.pdf