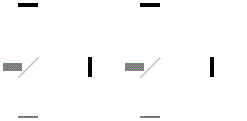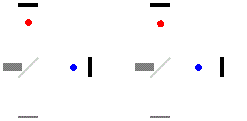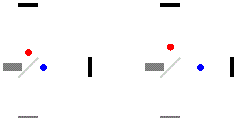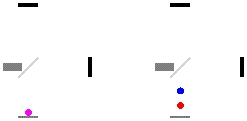1
$\begingroup$

When discussing the Michelson and Morley experiment (as though the aether existed) we say that the different beams of light would take different times to travel the two distances due to the aether wind and so would arrive out of phase.

I'm having a lot of trouble intuitively seeing how they take different times. I can't seem to grasp that the beam moving against/with the wind will be this take a longer time. I think this is as no equation made from it directly shows this conclusion. When I think about it I always get confused by the fact that the beam would be sped up and then a lower down, so in my mind it would still arrive at the same time.

If anybody could try and possibly explain why they arrive at different times in a simple way that would be lovely!

Improve this question
$\endgroup$
2
  • $\begingroup$ It might help for us to know what your level of math preparation is. I say that because many (most?) presentations of the experiment do exhibit the math, and I am wondering if the person who put together the material you are using did so for a reason. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Apr 24, 2017 at 16:27
  • $\begingroup$ Im currently at A-level standard (so the year before university). I've understood the more basic mathematical approaches but I can't visualise in my head why this equation results in a longer time. Do you understand what I am saying or should I try and reword myself? $\endgroup$
    – Jake
    Commented Apr 24, 2017 at 21:36

2 Answers 2

Reset to default
1
$\begingroup$

Suppose the aether is flowing past the Earth at a speed $v$, then when the light is travelling with the flow its net speed is $c+v$ and when it's travelling against the flow the net speed is $c-v$.

We'll call the length of the arm $\ell$, so for the trip with the flow the time taken is:

$$ t_1 = \frac{\ell}{c+v} $$

and for the trip against the flow the time taken is:

$$ t_2 = \frac{\ell}{c-v} $$

The average speed is then just the distance travelled, $2\ell$, divided by the total time taken, $t_1 + t_2$:

$$\begin{align} v_{av} &= \frac{2\ell}{t_1 + t_2} \\ &= \frac{2\ell}{\frac{\ell}{c+v} + \frac{\ell}{c-v}} \\ &= \frac{c^2 - v^2}{c} \\ &= c\,\left(1 - \frac{v^2}{c^2}\right) \end{align}$$

So even though you're quite correct that one leg of the trip is speeded up and the other leg is slowed down, the average velocity doesn't stay constant.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Why is the speed of the mirror not taken into account? Would it not be moving and so the distance be longer/shorter? $\endgroup$
    – Jake
    Commented Apr 24, 2017 at 21:40
  • $\begingroup$ @JakeRose The whole thing is done in a frame where the mirror is stationary. You can do it in a frame where the aether is stationary and the mirror is moving, or in a frame where both are moving if you like: galilean relativity tells you that the answer depends only on the relative velocity. $\endgroup$
    – user107153
    Commented Apr 24, 2017 at 23:52
0
$\begingroup$

I seem to have misunderstood your question. Your reasoning is correct. If you take time needed to cover a distance whilst going against the aether vs the time needed to cover a distance whilst going with the aether, then the time will be the same.

Given $Velocity=\frac{Displacement}{Time}$ where $d$ is the distance between mirror and light source

Case 1: Light source pointed with movement of aether

Time needed to complete first leg of journey: $t_1=d\div(V_{light}-V_{aether})$

Time needed to complete second leg of journey, after reflection: $t_2=d\div(V_{aether}+V_{aether})$

Case 2: Light source pointed against movement of aether

Time needed to complete first leg of journey: $t_3=d\div(V_{light}+V_{aether})$

Time needed to complete second leg of journey (after reflection): $t_4=d\div(V_{light}-V_{aether})$

Clearly the total time taken in Case 1 is the same as Case 2; $(t_1+t_2)=(t_3+t_4)$ but that's not what the Michelson-Morley experiment is about.

The key here is realising that the experiment's setup made light travel in directions perpendicular to each other through different times. Not one with the aether and one against aether, but perpendicular to each other and then measuring the interference pattern when they recombine. If one of the light beams had "assistance", the timing would be off. They did this by suspending the entire setup in liquid mercury and then rotating it. In other words, one of the light rays would have a different arrival time because it would be influenced by the aether movement, if there was any.

Here is a gif from Wikipedia that illustrates the difference perpendicular light rays would have if there was aether movement.

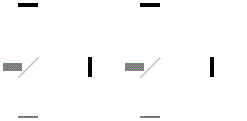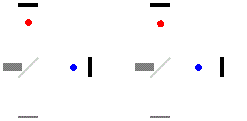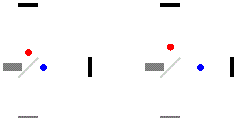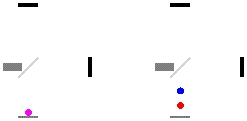
enter image description here

If there was aether movement in the horizontal direction (like in the gif), then the time taken for the horizontal light beam to reach the beam-splitter/mirror would be

$T_{horizontal}=t_{right}+t_{left}$

where $t_{right}=d\div(V_{light}-V_{aether})$

and $t_{left}=d\div(V_{light}+V_{aether})$

giving you $T_{horizontal}=\frac{d(2V_{light})}{V_{light}^2-V_{aether}^2}$

Think of the aether shifting the "endpoint" of the beam, that's why you see the blue dot bounce after the red dot bounces. Whereas the time taken for the vertical light beam to reach the beam-splitter/mirror would be

$T_{vertical}=t_{up}+t_{down}$

where $t_{up}=d\div V_{light}$

and $t_{down}=d\div V_{light}$

giving you $T_{vertical}=\frac{2d}{V_{light}}$

This is clearly different from the horizontal case, making the light beams out of phase and causing some interference. In this case, the horizontal motion of the aether doesn't affect vertical velocity.

Improve this answer
$\endgroup$
8
  • 1
    $\begingroup$ Is t2 and t4 really d/(2*Vaether), or is that a typo? It seems odd to me that the velocity would not include Vlight. Also, I think it might make sense to finish off the equations to compare the velocities seen in M-M, rather than leaving it as an exercise to the reader. I think it might make the gif more poignant (it's an awesome gif, btw!) $\endgroup$
    – Cort Ammon
    Commented Apr 24, 2017 at 21:16
  • $\begingroup$ Yes, thanks for the catch, it was a typo. I left out the hard maths like length contraction, Lorentz transforms or fringe shift calculations because the OP asked for a simple and intuitive way of understanding how M-M's experiment worked. I'm also not terribly confident of my mastery of the math. Or perhaps you are referring to some simple math that my answer is missing ? Would you mind being more specific ? Thanks. $\endgroup$
    – HsMjstyMstdn
    Commented Apr 24, 2017 at 22:08
  • $\begingroup$ I was referring to the simple math. If the aether theory was correct, we would see periods of t1+t2 or t3+t4 in the parallel direction and 2d/Vlight in the perpendicular direction. It took me a bit of muddling about in the math to convince myself that t1+t2 did not equal 2d/Vlight. It might be worth doing the last little bit of math show what velocities were expected from M-M by those who believed in the aether. That math might provide more context for the intuitive gif that follows. If you like, I can edit it in. (I didn't want to edit it until I was confident the above error was a typo) $\endgroup$
    – Cort Ammon
    Commented Apr 24, 2017 at 22:23
  • $\begingroup$ I'll give it a try (might be good for me, too). $\endgroup$
    – HsMjstyMstdn
    Commented Apr 24, 2017 at 22:26
  • 1
    $\begingroup$ Yes, that ties the math in quite nicely. Thank you! $\endgroup$
    – Cort Ammon
    Commented Apr 25, 2017 at 0:32

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.