1
$\begingroup$

My question is similar, if not identical, to this one, but I don't find the answer satisfying, given the context of experiment.

First, here is an outline my understanding of the motivation behind the experiment:

Galilean invariance states that the laws of motion are identical across inertial frames of reference.

However, it was also known (or suspected), that the speed of light is independent of the speed of its source (as is the case in sound waves).

This presented the apparent opportunity to violate Galilean invariance, and the Michelson Morley experiment is a famous example of an attempt to do just this.

Based on the assumptions and working knowledge of the scientists of the time, if the interferometer was traveling through the ether, then the interference pattern should differ when the contraption was rotated, and this would confirm that the contraption was moving with respect to the ether (i.e. with respect to absolute space), and allow a calculation of the velocity of the Earth (assuming the contraption and the Earth were in the same inertial frame).

The key motif of the Lorentz transformation can be derived from this analysis, which is based upon the Pythagorean theorem:

$1/\sqrt{1-v^2/c^2}$.

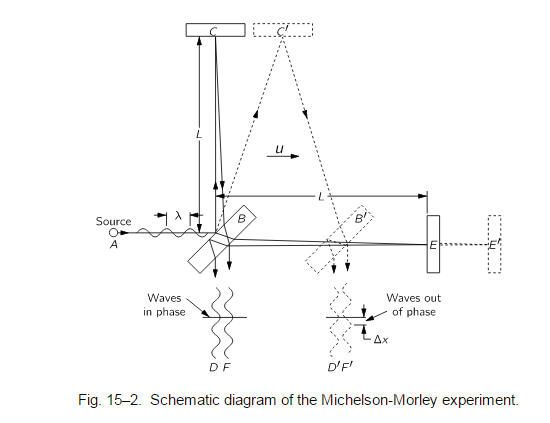
Here is a schematic of the Michelson-Morley apparatus, borrowed from here

Interferometer

The idea here is that, since we had not yet discovered that the speed of light is invariant across all observers within and across all inertial frames, we would expect two particular round trip times for both beams of light, and these times would depend upon which direction the contraption was moving, and at what speed.

I can fully follow and understand the calculation for the B - E' - B' roundtrip.

I can also fully follow the calculation for the B - C' - B' roundtrip, but I can't understand it. In particular, I don't understand why the first half of this round trip strikes the center of the mirror C'. Remember, those scientists already understood that the speed of light is independent of the speed of its source, so it wouldn't be like bouncing a ball against the roof of a moving vehicle, where the ball inherits the forward speed of the vehicle. Rather, the beam should strike a point behind the center of C' (i.e. to its left). In other words, to an outside observer, the beam would be expected to go straight up and down.

The only way I can reconcile this is that Feynman here is not talking about a laser beam, but rather a point source where the light spreads out in all directions. And in that case, the analysis is done for the particular photon whose angled path through space was such that it struck the center of C'. However, I don't think this is the correct answer to my question, as the same issue crops up later in the discussion of a moving light clock that involves a single photon.

Another way of asking this question is as follows:

According to the way of thinking at the time of Michelson-Morley

If I were to aim a laser beam at a very distant target, and both myself and the target were moving rapidly in a direction perpendicular to the line between myself and the target, then if the distance between myself and the target was great enough, then, by the time the laser beam reaches the target, it will have dodged the laser beam.

Yet, according to my reading of Feynman, their expected calculations imply that the light is carried along by the velocity of the source, which contradicts what they apparently already knew at the time.

What am I missing here?

Improve this question
$\endgroup$
6
  • $\begingroup$ probably this question will be closed.... $\endgroup$
    – Sidarth
    Commented Feb 20, 2016 at 2:31
  • $\begingroup$ Compute for yourself how much the beam should have been deflected by the Earth's motion thought the putative aether and compare that to the size of the optical elements. (I think that MM used an $11 \,\mathrm{m}$ optical path for each leg.) $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Feb 20, 2016 at 2:33
  • 2
    $\begingroup$ And certainly neither Michalso or Morley had any idea what a laser was... $\endgroup$
    – Jon Custer
    Commented Feb 20, 2016 at 2:44
  • $\begingroup$ @dmckee, the question isn't a practical consideration one, it's a theoretical one. I'm asking why they expected the beam to inherit the velocity of the interferometer, as it appears to do in the diagram. $\endgroup$
    – spacediver
    Commented Feb 20, 2016 at 2:55
  • 1
    $\begingroup$ The size of the effect is a theoretically relevant idea because the beams they used (as @Jon notes not lasers but some incoherent source manipulated until what remained was coherent enough) were subject to diffraction. If the expected deflection left enough light from the diffraction halo to distinguish the changing fringes all would be well. And frankly Michelson had been working on earlier version of this beast for a decade or more. He knew we wasn't going to measure (or have problems with) the beam deflection even if he didn't have an explanation. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Feb 20, 2016 at 4:03

2 Answers 2

Reset to default
2
$\begingroup$

I may be wrong, but I think the effect of the ether would just be to alter the alignment of the mirrors slightly. It is kind of analogous to hitting a golf ball or kicking a football in the wind. If the wind is blowing one way, you have to aim a little the other way to correct for the wind.

For example, in the figure you give, the apparatus is moving to the right through the ether, or, in the apparatus's frame, the ether is moving to the left. When you are aligning the optics, you will try to get the spot to land on the mirror $C$. If the ether is still, you can just have the mirror at a 45 degree angle, but when the either is moving to the left, you will have to tilt the mirror so as to aim the beam a little more to the right. Similarly, for the return trip you would have to alter the alignment of mirror $C$ to correct for the either.

However, since you align the mirrors by looking at where the spot from the light is instead of trying to precisely set the angle of the mirror to 45 or 90 degrees, you wouldn't really be able to tell during the alignment whether there is an ether. The ether doesn't really affect your alignment procedure.

Hopefully this answers your question.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thanks very much for the reply. It makes perfect sense to me. The problem is that in the next example in the text, the same analysis is applied to a light clock, and it's pretty clear in that example that the beam of light isn't aimed at a particular angle in anticipation of the expected position of the mirror and receiving photocell, as it may have been in the case of the interferometer. $\endgroup$
    – spacediver
    Commented Feb 28, 2016 at 5:18
0
$\begingroup$

The only way I can reconcile this is that Feynman here is not talking about a laser beam, but rather a point source where the light spreads out in all directions

I think that the reasoning of Feynman in this lecture is based on this, because he previously in it said that Maxwell's equations demonstrated that light goes in all direction from the source.

But, as you said, what about a light beam? I am thinking a lot about that, and I have a "unsure response" .

If we think in Galilean mode we arrive to a contradiction. Because we know from Maxwell equations that light propagation does not depend on the source speed (this is, too, a conclusion of De Sitter double star experiment), but we check that light in this experiment has a crossed component "due to the traslation of the observer". This contradiction only could be fixed with a new theory (SR), I think that this itself must have been a problem in its momment, apart from the zero result of MM.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.