Quantum critical region governed by quantum critical point

Question

I am trying to understand the following statement about quantum critical regions associated with a quantum phase transition from page 4 of these lecture notes on holographic superconductors:

The QCR is characterized by the requirement that T be large compared to the dimensionally appropriate power of (g−gc). It seems reasonable to expect that the effective scale invariant field theory valid at the critical point, now generalized to nonzero T, can be used to predict the behavior of the system in the QCR.

The phase diagram of the system:

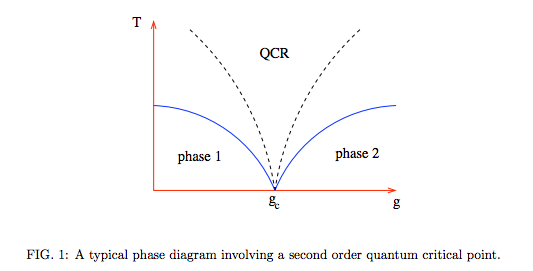
Why can we expect the field theory describing the system near the quantum critical point to also be a valid description in the quantum critical region?

Near the quantum critical point, the energy gap $\Delta$ vanishes and the coherence length $\xi$ diverges in the following way: $$ \begin{align} \Delta &\propto (g-g_c)^{z\nu}\;, \\ \xi &\propto (g-g_c)^{-\nu}\;, \end{align} $$ where $z$ is the dynamical exponent.

I understand that, at large $T$, the mass gap becomes negligible, $\Delta \ll T$, and the coherence length effectively diverges, $\xi \gg T^{-1}$, just like close to the critical point. But why should the emerging scale invariant theory be the same as the one close to the quantum critical point? Where does the condition that

T be large compared to the dimensionally appropriate power of (g−gc)

come from to characterise the critical region?

Answer 1

I have found an answer to the question in Sean Hartnoll's Lectures on holographic methods for condensed matter physics:

Quantum critical points can dominate regions of the phase diagram away from the point at which the energy gap vanishes. For instance, in regions where the deformation away from criticality due to an energy scale $\Delta$ is less important than the deformation due to a finite temperature $T$, i.e. $\Delta < T$, then the system should be described by the finite temperature quantum critical theory. This observation leads to the counterintuitive fact that the imprint of the zero temperature critical point grows as temperature is increased.

At $T \gg \Delta \propto (g-g_c)^{z\nu}$ the mass gap is effectively negligible and the theory becomes scale invariant again. Hartnoll says that the deformation due to finite temperature $T$ dominates the deformation due to the mass gap $\Delta$.