I am currently learning about Optics using an MIT OpenCourseWare Course. I have in particular a question about the application of Fermat's principle in Lecture 3, i.e. deriving the shapes of parabolic reflectors and ellipsoids of refractive material for a given focal point. The lecture notes could be found here, and the derivations in question are on the first slides.
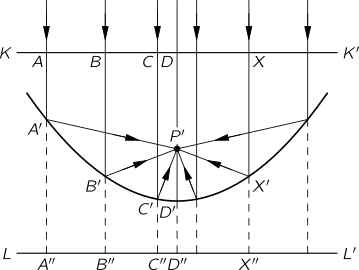
For example, consider the derivation of the paraboloidal reflector (3rd slide), he does this derivation at around minute 6 in the video.
In short, he says that all rays getting reflected and hitting the focus point F should be the same length by Fermats principle that the optical path length (or the time taken by the photons/wave fronts) should be minimal.
But I doubt that reasoning and have certain objections about his arguments.
1) First you cannot measure distance for points in infinity (as $\infty + c = \infty$), but if we replace infinity with some unspecified far away point, then we can put some plane behind F and measure distance from this plane onwards (as the rays before hitting that plane have all the same distance). So this is easily fixed.
2) In my opinion Fermats principle is just applied for the ray that goes directly through F and is reflected in the exact opposite direction (i.e. the ray on the optical axis), and that it must have this path is easily seen with Fermats principle. But the reasoning that all the other paths must have the same length (or must take the same time) is not Fermats priniple as I see it, as I see it an additional argument must be in order here, I can image that we might require that the photons that start at the same time (or the wavefronts thereof) should all meet in F, hence should all take the same time (or path length).
Am I right? Is the author a little bit sloppy here and are additional arguments in order? I have the feeling that Fermats principle is not enough, or said more provocative that it is not the crucial part of the argument (mainly that all paths should have the same length), but it just gives the magnitude of the common path (i.e. $2f$ in the lectures).
PS: A student seems to ask a similar question at around minute 11, but the answer does not seem to address it properly in my opinion (and btw. also thread infinity as a point from which we can measure distance).