Can people traveling at difforent speeds gain information at a different rate?

Question

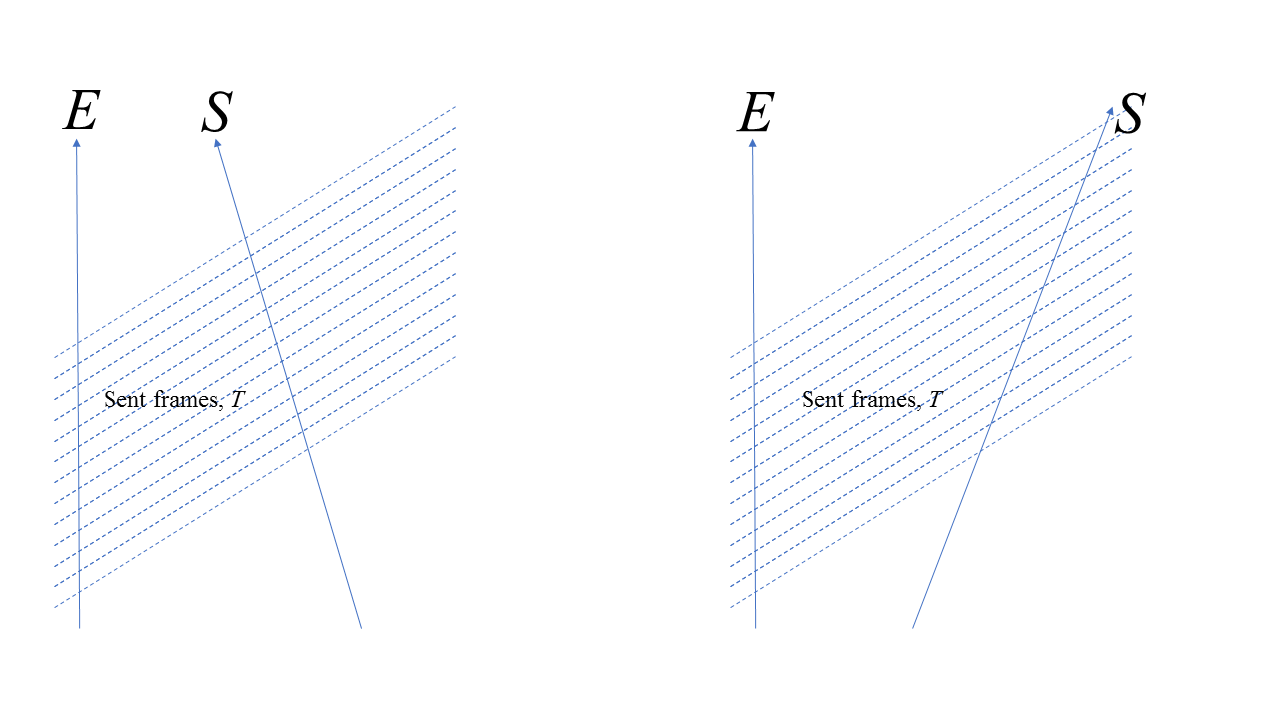
I heard that the faster you go, the slower time around you goes. For example, if you where in a rocket going very fast and you started a timer for 1 minute at the same time someone walking down the street started a timer for 1 minute, the person on the street's timer would go off just before yours. I also know that people going at very fast speeds can still get information (for this example lets say that it is from the internet).

So lets imagine that the person on earth starts streaming a TV show to the person in the rocket. Can the person in the rocket watch the TV show faster than the person on Earth? This would not make sense to me, given that they both experience time at the same speed.

Answer 1

This would not make sense to me, given that they both experience time at the same speed.

See this answer of mine for background. Although I'm not a psychologist, I think it's reasonable to surmize that the experience of time is defined by how quickly one's own bodily processes run forward relative to the rate of other physical processes in one's nearby, comoving neighborhood. This explains why the rate of one's own time progression is never perceived to be anything other than "one time unit per one time unit".

But this does not stop signals arising from sources elsewhere that one receives from progressing at different rates if the relative motion between source and receiver change.

And, your spacefarer will indeed see the television transmission progressing more swiftly, or more slowly, depending on whether their motion is towards or away from the signal source.

There are two simple ways to see this.

1. The modulated TV signal, with all its framing and timing information, can be represented by a Fourier transform. The Doppler shift induced by the relative motion means that the frequencies of all the Fourier components are scaled by the same, relative velocity dependent, scale factor. So, not only does the Doppler effect change the carrier frequency, it speeds up or slows down the arrival of frames depending on whether motion is towards or away from the source.
2. (Actually this is of course one way to deriver the Doppler shift): draw a Minkowski diagram (see my sketch below), with regular framing pulses $T$ being sent from the Earth's worldline ($E$). Then work out the Minkowski length of the successive crossings of the spacefarer's world line $S$ by these framing pulses. You can see at a snap that the rate depends on the direction.

Answer 2

My answer here is directly applicable to this question; there the traveler was watching earth through a telescope, whereas here the traveler is watching an Internet broadcast, but exactly the same analysis applies.

As in that case, the bottom line is that if you're in your rocket ship traveling away from earth, you'll see the broadcast in slow motion for two reasons: One is the relativistic time dilation and the other is that you keep running away from the broadcast source. On the other hand, if you're in your ship traveling toward the earth, then the time diation slows the broadcast down, but the fact that you're moving toward the source speeds it up, and the latter effect wins (though it takes a little algebra to show this).

@WetSavannaAnimalakaRodVance has given you the same answer, explained a bit differently, but of course his answer is the same because he's doing it right and there's only one right answer. I'm adding this one only because it's sometimes useful to see the same thing explained a couple of (slightly) different ways, and to point out that this and the other question are identical (and that one in turn is marked as a duplicate!).