Different time for same amount of kph/mph change at different speeds

Question

First of all excuse me if my english isn't perfect, and if the question is hard to understand, even I am a little confused about what do I even want to ask.

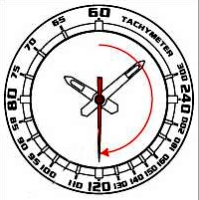
So I bought a new wristwatch not too long ago, and it has a scale called "Tachymeter". It is basicaly a speed metering tool, and works like this: You are going with for example a car, start the stopwatch and stop it after you travelled 1 kilometer. Where the stopwatch stops, the tachymeter scale will tell you the average speed. (note that it is going backwards on the kph scale, as it takes you more time to cover a fixed distance when going slower of course) Its pretty simple. Here is a picture of how it looks:

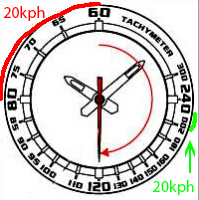
Now my problem is that I noticed that at lower speeds it takes more time for the stopwatch to "travel" across the same amount of kph than at higher speeds. For example the time it takes for it to get from 80 to 60 kph is 15 seconds, but to travel the same 20 kph difference from 220 kph to 200 kph is only 2 seconds.

Now this was my main concern, and I dont even know how to ask a question about it, but this makes me quite confused. Its like the kph unit is not linear with the amount of the elapsed time or something.

Can anyone explain to me what is going on?

Thank you for the answers!

Answer 1

https://en.wikipedia.org/wiki/Tachymeter_(watch)#Measuring_speed

The watch hand always moves at a constant speed, and you always measure a constant - 1 unit - distance (km in your case). The variable is the amount of seconds you devide the units with. E.g. if you cover 1 unit in 1 sec, and the unit is km your average speed will be 3600 km/h. If you cover 1 unit in 10 sec, and your unit is km, your average speed will be 360 km/h, and so on.

So the slower you are the less your average speed changes with any added second. If you add 1 sec to 1 sec you increased the time by 100%. If you add 1 sec to 100 sec, you just increased the time with 1%. So naturally your scale is hyperbolic in nature.