Can you measure temperature using blackbody radiation and a 13GHz receiver?

Question

I have a modified satellite dish (like used for receiving satellite TV) that I've twisted into a sort of combination radio telescope and microwave camera.

I found that I can detect objects (and make pictures of them) with it even in areas where there is no source of microwave radiation.

The obvious source is blackbody radiation, so I thought maybe I could measure the temperature by differences in the received signal.

Simply measuring the signal strength won't help - that is dependent upon the distance from the object.

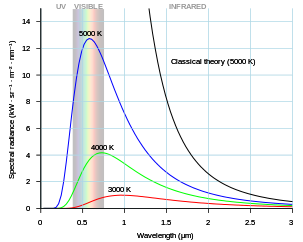
My idea was to measure at two separate bands, and use the slope of the line between the two signal strengths to determine the temperature. This image borrowed from Wikpedia's blackbody page shows that slope of the curve varies with the temperature.

My idea is to measure the intensity for the 10.7 to 11.7 GHz range and for the 11.7 to 12.75 GHz range (Astra low and high band) and calculate the slope of the average for each range - that is, the slope between two points.

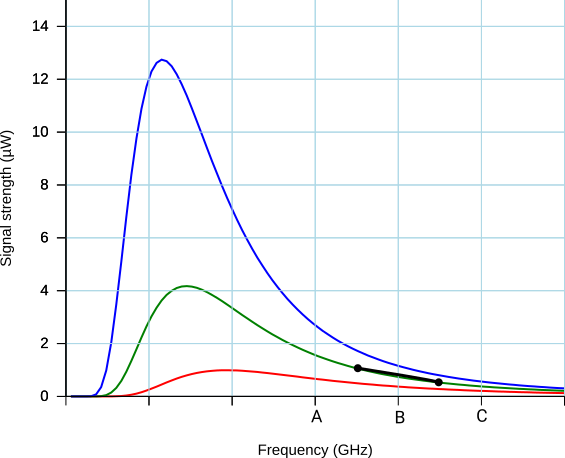
Like this:

My device can deliver the averages for the range A-B and B-C. The slope of this line is easy to figure.

Then, I would solve the blackbody equation for the given ranges and find a temperature that approximates the slope of my line over the needed ranges.

Using the slope makes it (mostly) independent of the absolute values that I measure - distance to the object is no longer a problem unless the signals are so weak they drown in the noise of the circuitry.

I haven't worked through the math yet. I wanted to ask if is even possible before I try working through the math. I'm not terribly good at that kind of thing, and can slog it out when necessary, but I'd rather not do so if the whole idea is unworkable.

There are of course restrictions to the math that I can see. The slopes will be the same at various segments depending on the temperature. So, I have to know ahead of time whether I'm measuring the temperature of a furnace or of a tree (or the moon, which is what I'm really interested in) to that I can match the slopes in appropriate temperature range.

I'm aware that there are plenty of technical difficulties involved in this. Those aren't part of the concern of this question.

I'm trying to find out if this is theoretically possible before I start worrying about the technical aspects.

Given that I can easily detect difference in intensity between different pieces of wood, I expect the equipment is up to the task.

---

Just to show how sensitive my gadget is, here is a reflection of a tree on the wall of my house taken with the scanner pointed at the wall - the tree is behind the scanner. The fuzzyness is a focus problem, not a measurement problem.

---

I found time to write a python script to do blackbody calculations last night.

It turns out that the slope of the line between the two center frequencies of the available bands is pretty much the same for all temperatures above about 10 degrees Kelvin. Above that, the differences in the slope are more than 4 places after the decimal point when the difference is expressed in dB. It gets worse the temperature rises.

At room temperature, the differences are in 5 to 6 digits after the decimal point.

My gadget can reliably resolve to 0.01 dB. Measuring a temperature the way I wanted to would mean my gadget would have to measure to 0.00001dB and better for room temperature.

The differences would be large enough (larger than 0.01dB) to measure if the temperature of the radiating object is close to absolute zero. But, that only applies to temperatures less than 3 degrees Kelvin.

Whilst monkeying around, I also checked the temperature for a radiation peak at 11GHz - turns out to be like 0.1 degree Kelvin.

So, my gadget is capable of detecting blackbody radiation, but I can't use the difference in the measurements in the two bands to measure temperature at any kind of reasonable temperatures.

Answer 1

First, let's check whether the expected power levels are large enough to actually detect. The power spectral radiance of black body radiation at frequency $\nu$ and temperature $T$ is

$$B(\nu) = \frac{2 h \nu^3}{c^2} \left( \exp \left( h \nu / k_b T \right) - 1 \right)^{-1} \, .$$

Putting in numbers, a room temperature surface of area 1 square meter emits about $$10^{-13} \, \text{mW/Hz}$$ at 11 GHz. That's roughly -130 dBm/Hz. An off the shelf spectrum analyzer from Keysight/Agilent/whatever has a noise floor of -140 dBm/Hz. Therefore, if we could collect all of the power coming from our object, we should be able to see it above the noise of an off-the-shelf detector.

Of course, collecting all of the emitted power is not possible. We lose some to the fact that we can't cover all emission angles from our object, and we lose more because our antenna isn't perfectly mode/impedance matched to the incoming radiation. I don't know much about antenna physics so I can't comment on whether or not a real system can be expected to actually image room temperature objects via 11 GHz thermal radiation.

That said, we should point out that radiation from WiFi or local radio stations might provide a larger-than-thermal background, enabling 11 GHz imaging anyway, and it's important to note that shielding ambient GHz radiation can be harder than one might expect $[a]$.

Ok, now let's get to the main question, which is whether or not we can tell the temperature of a thing by the difference in detected black body radiation power measured at 11.2 GHz and 12.2 GHz (the mid points of the ranges indicated in the question). Well, let's just plug in numbers, again for a 1 square meter object (assuming detection of all $4 \pi$ solid angle of radiated power) at 300 Kelvin. At 11.2 GHz we get $1.4 \times 10^{-13} \text{mW/Hz}$, and at 12.2 GHz we get $1.7 \times 10^{-13} \text{mW/Hz}$. This gives a ratio of 0.8 dB, which a commercial device could certainly resolve.

However, the real question is whether or not these numbers are different enough to measure at a different temperature. Let's suppose we drop the temperature to freezing, i.e. 273 Kelvin. Now at 11.2 GHz we get $1.3 \times 10^{-13} \text{mW/Hz}$ and at 12.2 GHz we get $1.6 \times 10^{-13} \text{mW/Hz}$. The ratio is 0.9 dB. That's really close to what we had at 300 Kelvin and I'd be very surprised in a home made device could tell the difference between these two cases. I'm not saying it's impossible, just probably pretty hard.

As a final tidbit, here's a useful formula for the slope of the black body spectrum

$$\frac{dB/B}{d\nu/(k_b T/h)} = 3 \frac{k_b T}{h \nu} - \frac{e^{h \nu/k_b T}}{e^{h \nu / k_b T} - 1} \, .$$

$[a]$: I once wrapped a cell phone in aluminum foil, put the phone in a microwave oven, closed the door, wrapped the microwave oven in aluminum foil, and called the phone. It rang.