7
$\begingroup$

I really cannot get how a measuring instrument with the smallest division of let's say 20 has an estimated uncertainty of half that value. Firstly, why half, and secondly, why the smallest division? Can't the instrument be more precise than that? If possible, kindly explain with diagrams, thank you a lot.

Improve this question
$\endgroup$

5 Answers 5

Reset to default
9
$\begingroup$

Uncertainty appears because of the limits of the experimental apparatus. If your measuring device can measure up to 1 unit, then the least count of the measuring device is said to be 1 unit. You cannot get any more accurate than the least count.

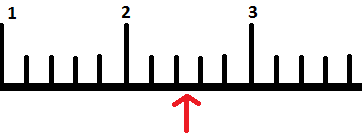
enter image description here

Suppose your scale showed a reading as shown above. It obviously lies between 2 and 3 but can you get more accurate? Yes, you can get a more accurate value. The pointer lies between the 2nd and the 3rd lines after the 2. It lies somewhere in the middle, you don't know where. Your instrument does not let you measure more accurately. As it could lie anywhere between the two division lines, the uncertainty is said to be the width of the division lines. In our case, it is $0.2$ units. This is also known as the least count of the instrument.

You will report your reading as $2.4 \pm 0.2$ units.

However, there is a smarter way to report the value but is generally not preferred. You can approximately guess which side the pointer leans to, i.e: to $2.4$ or $2.6$. If it leans more towards $2.4$, you can report the value as $2.45 \pm 0.01$ and if it leans more towards $2.6$, you can report the value as $2.55 \pm 0.05$. Your professor would probably hammer you for reporting the values that way.

A better way to report the value is $2.5 \pm 0.1$.

You can reduce the uncertainty even further by buying better equipment which can measure the quantity more accurately (smaller least count).

Improve this answer
$\endgroup$
4
  • 3
    $\begingroup$ I would have reported the value as 2.5, plus or minus 0.1 $\endgroup$
    – David White
    Commented Mar 10, 2017 at 17:51
  • $\begingroup$ Good point. Will add that to the answer. $\endgroup$
    – Yashas
    Commented Mar 10, 2017 at 17:55
  • $\begingroup$ I think the total uncertainty of that rule is 0.2 because we have to consider 0.1 on both sides of the object being measured. See my reply $\endgroup$
    – juanrga
    Commented Aug 12, 2017 at 17:41
  • $\begingroup$ In most cases, it is possible to align the scale and object accurately. Your point is valid though. There will some uncertanity at the start but that is very small in most cases. $\endgroup$
    – Yashas
    Commented Aug 13, 2017 at 2:35
4
$\begingroup$

We have to make a distinction between analog instruments and digital instruments.

For digital instruments the uncertainty is usually $\pm1$ of the last significant figure (unless you're told otherwise by your instrument sheet). When I had to measure mass in my laboratory course, if the resolution of my instrument was 1 g, I had to write $m=(125\pm1)$ g. Sometimes my error was higher than the instrumental resolution (due to specific note of the manual), but never smaller.

For analog instruments with graduating scales usually your uncertainty is half of the smallest increment you can measure. For example, consider a ruler. You're measuring a pencil and, by sight, you can see that the pencil is closest to 36 mm than it is to 35 mm or 37 mm. Maybe it's 36.2 mm, maybe it's 35.8 mm. But you're sure that it's not 35.2 mm, because you actually SEE that the closest tick is the 36 mm one. So you can conclude that your measure lies between 35.5 mm and 36.5 mm. You get $L=(36.0\pm0.5)$ mm.

This are the "rules" we used in every laboratory course. For the graduating scale measure my reference is the $1^{st}$ chapter of "An Introduction to Error Analysis" by J.R. Taylor.

Improve this answer
$\endgroup$
2
$\begingroup$

Think of reading a measurement taken with a digital instrument. Suppose you are watching your weight and when you get on the scale it reads 76.4 kg. The chances you are EXACTLY 76.4 kg are really slim (actually 0). In fact, the scale will tell you that you weigh 76.4 kg if you are anywhere between 76.35 and 76.45 kg. Thus, the error in your measurement is $\pm 0.5$ kg, and you would report your measurement as $76.4$ kg $\pm 0.5$ kg

A more precise scale might tell you you weigh 76.42 kg or 76.40 kg, in which case your error is $\pm 0.05$ kg.

Improve this answer
$\endgroup$
1
$\begingroup$

If you're looking at a pointer somewhere in the smallest division, you may be able to tell what half it is in by sight. But you'll have difficulty telling where it is more precisely than that. So the smallest quantity you can read from the scale is half of the smallest division.

Also, measurement devices can go wrong, so the accuracy might sometimes be less than the scale on the device can in principle register.

Improve this answer
$\endgroup$
1
$\begingroup$

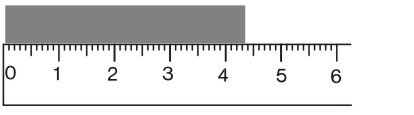
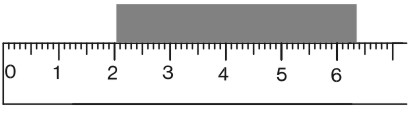
The uncertainty of a rule is the smallest division, because a length is the difference between two points

enter image description here

The right-hand end of the object is $4.35 \pm 0.05$ (in the corresponding units), but the left-hand end is subjected to the same uncertainty. Therefore, the length of the object will be

$$(4.35 \pm 0.05) - (0.00 \pm 0.05)$$

or $4.3 \pm 0.1$. The existence of an uncertainty on both sides of the object becomes more obvious if we measure the length as

enter image description here

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.