2
$\begingroup$

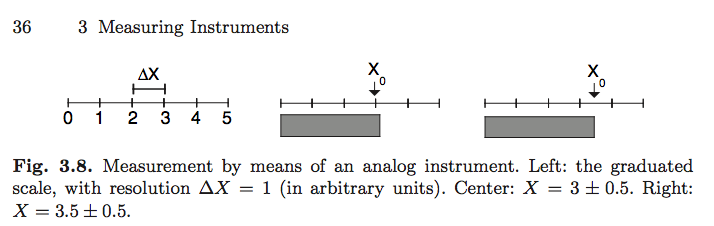
As a first approximation, the uncertainty ($\delta X$) associated to a mensurand can be expressed as $\delta X= \Delta X / 2$ with $\Delta x$ being the resolution of the instrument.

There is also a recommendation indicating that the numerical expression of the result and its uncertainty saying that "Results should be rounded to be consistent with the uncertainty given" (EURACHEM/CITAC Guide CG 4).

So, if we have an instrument like a ruler with a resolution of 1 arbitrary unit ($\Delta X =1$ ) and when measuring an object, the end of said object is very close to the 3 unit mark, why should the reported value be 3 $\pm$ 0.5 and not 3.0 $\pm$ 0.5 or even 3.1 $\pm$ 0.5?

enter image description here

Improve this question
$\endgroup$

2 Answers 2

Reset to default
2
$\begingroup$

The problem with your question (and figure) is that the resolution is well beyond the gradation of the instrument, and that is misleading.

So let's suppose can't really distinguish 3.1 from 3.5, all we know is that 3.1 is closer to 3 than 5, and while 3.5 is in the middle, we'll round it to 4.

In this case the uncertainty is the standard deviation of the process, not the extrema (or half the extrema). The standard deviation is that of a uniformly distributed random variable on [0, 1], which is:

$$ \delta X = \frac 1 {\sqrt{12}} $$

Improve this answer
$\endgroup$
1
$\begingroup$

This is because the first part of the value represent the significant digits that are certain. The answer must contain extra significant digits on top of the ones that are certain. Having taken the reading, you are 100% sure that the ones digit is $3$ and not $4$ or $2$. Writing $3.0$ would mean that you are sure of the length to be $3.0$ which you are clearly not. Same goes with writing $3.1$

Improve this answer
$\endgroup$
3
  • $\begingroup$ I see. And that is why, for the case on the right side of the Figure, where it is more obvious that the end of the object is in between the marks, the measurement can be expressed as 3.5 $\pm 0.5. Thanks $\endgroup$
    – elisa
    Commented Jan 14, 2020 at 17:52
  • $\begingroup$ If you can "eyeball" it to better than half the smallest division, maybe the +/- 0.5 is not appropriate. $\endgroup$
    – puppetsock
    Commented Jan 14, 2020 at 17:56
  • $\begingroup$ @elisa Actually, I was always taught to represent it as $3{\pm}0.1$ because the least count of the instrument is $0.1$unit. $\endgroup$
    – Sam
    Commented Jan 14, 2020 at 18:02

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.