4
$\begingroup$

I'm having trouble understanding simple error analysis of a ruler. Suppose we have this ruler.

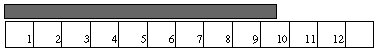
enter image description here

There is a mark for every centimeter. The precision is half a centimeter. This should mean that the rulermaker guarantees us that about 68% of the time (I don't think this is true in most cases), the true value will be in the interval $(x-0.5 \mathrm{cm}, x+0.5 \mathrm{cm})$.

This is because de ruler/marks don't have the exact lenght. If the ruler reads $2\mathrm{cm}$, when it should be $2.5\mathrm{cm}$, what would the error at the $1\mathrm{cm}$ be? If the ruler is a bit too long wouldn't this be reflected for every mark?

Is this the correct interpretation of uncertainty?

Why isn't there less error when the tip of the object we want to measure coincides with a mark of the ruler?

And if we don't measure the object from the tip of the ruler($0\mathrm{cm}$), so we have to calculate the difference, should we have to double the error?

Improve this question
$\endgroup$
2
  • $\begingroup$ I think you're confusing accuracy and precision. The ruler is only precise to within a half cm (to the eye of the user) while it's only as accurate as the spacing was made correctly. Using your picture, I can make that measurement 5 times and say that it's between, say, 10.3 and 10.5 each time. That's precision. But it really could be 15 because the hash marks are wrong, that's accuracy. Not that this is a full answer, but maybe that will help refine the question/answers. $\endgroup$
    – tpg2114
    Commented Dec 9, 2014 at 23:40
  • $\begingroup$ This is really a terrific question, and one that deserves a good answer that includes issues of discretization, estimation, calibration, shape of error function, effect of repetition, the difference between precision and accuracy, and other things. In other words - it will take some time to answer this properly. $\endgroup$
    – Floris
    Commented Dec 10, 2014 at 0:28

2 Answers 2

Reset to default
2
$\begingroup$

If you are measuring in a laboratory with a ruler like the one in your diagram then I would say for a length of $9.5 cm$ you would be able to see with your eye that the length is say $9.5 \pm 0.2 cm$ and if it actually was on one of the markings, e.g. 6, then you might estimate that the measurement was say $6.0 \pm 0.1 cm$.

Often when measuring length with a ruler we have to estimate what the length is and judge how accurately we can make the measurement.

The problem with estimation is that it is subjective. Ideally it would be good to have an objective way to measure error. For example, if you could measure something 10 times and you get slightly different values each time then the mean is your best value for the measurement and the standard deviation divided by the square root of the number of measurements is the uncertainty or error in the measurement.

If you had to measure two positions to calculate a length then you might have $$ X = A-B$$ and from that we can make an estimate of error in $X$ with $$ \delta X = \sqrt{\delta A^2 + \delta B^2}$$ but sometimes this is simplified to $$ \delta X = \delta A + \delta B$$ which is approximately correct, but a bit pessimistic.

related question/answers with reference to combining errors

Improve this answer
$\endgroup$
2
  • $\begingroup$ I know that in my basic science classes, they say to use what the measuring device can say definitely, and then estimate where it is between graduations. $\endgroup$
    – CoilKid
    Commented Dec 10, 2014 at 1:15
  • $\begingroup$ @CoilKid yes i agree. You have expressed it well. That is the point that I try to make at the beginning. $\endgroup$
    – tom
    Commented Dec 10, 2014 at 2:33
0
$\begingroup$

First, the accuracy of the ruler because of manufacturing errors is generally smaller than the reading error of the ruler. Physicists use the largest error, which in this case is the reading error. This is often answered incorrectly in Google searches on measurements. The reading error for a standard ruler with mm increments is +/- 0.1mm under perfect conditions. That is, no parallax error and the ruler is close enough to the device being measured to guess at 1/10 increments of a mm. In your example, the smallest increments are 1 cm, so this ruler should easily give a measurements error of +/- 0.1cm. In your example it looks like the 2 ends are -0.1cm and 9.5cm with errors of +-0.1cm. Thus, the total length is 9.6 +/- 0.2 cm. Both measurements of length do contribute to the error so we add the errors but this is actually an approximation being generously conservative. Really, the measurements should add in quadrature as SQRT((0.1cm^2) + (0.1cm^2)) = +/- 0.14cm. The reading error of 0.1cm is because we can intuitively picture that the largest guess one might give is 9.7cm and lowest would be 9.3cm. Thus, 96% of guesses for sure would be in the interval 9.3cm to 9.7cm and 68% of the guesses would realistically be between 9.4cm and 9.6cm. But you have to make this judgement call based on the readability of the setup. You should make it honestly. If you use a high or conservative measuring error then you will get an unnecessarily imprecise result. On the other hand, overly ambitious errors will likely give a result that is overly precise but inaccurate when the experiment is duplicated by others. For serious work, like publications, you should perform many sample measurements and calculate the error statistically. Making many measurements will also reduce the total error proportionally to the square root of the number of measurements taken.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.