On Microscopic level can you explain how the equivalent resistance of resistances connected in parallel is less than even the smallest resistance among them
$\begingroup$
$\endgroup$
3
-
$\begingroup$ Analogy: why is it easier for a given amount of water to flow through two tubes in parallel, compared to flowing through just one tube? Now what if you replace water with electrons and tubes with wires? $\endgroup$– jabiraliCommented Feb 16, 2017 at 18:15
-
$\begingroup$ But its not tube. Theses are resistance which are hindering the flow $\endgroup$– NerdCommented Feb 16, 2017 at 18:18
-
$\begingroup$ Actually, there are numerous analogies between fluid mechanics and electronics that may be intuitively helpful, including that a resistor is similar to "a constriction in the bore of the pipe which requires more pressure to pass the same amount of water. All pipes have some resistance to flow, just as all wires have some resistance to current." $\endgroup$– jabiraliCommented Feb 16, 2017 at 18:39
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
It's instructive to think of the inverse of resistance: the conductivity. This tells you "how many electrons (how much current) per unit time" a particular conductor can transport, given a voltage.
When you have multiple parallel paths, the total current that flows is the sum of the currents flowing through each. That is, the conductivity of the parallel circuit is the sum of the conductivities of all the conductors (resistors).
So the total conductivity is greater than the conductivity of any single resistor. And since conductivity is the inverse of resistance, if the conductivity is greater, the resistance is smaller.
$\begingroup$
$\endgroup$
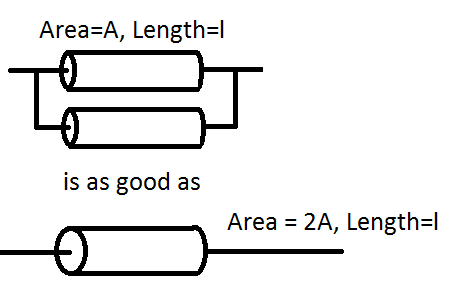
The formula used to calculate the resistance is: $$R = \rho \frac{l}{A}$$
As the area increases, resistance decreases. If you double the area, the resistance gets halved. This is obvious as increasing area would mean lesser congestion.
If you connect two identical resistors in parallel, the current flowing would see it as a resistor with twice the area.
