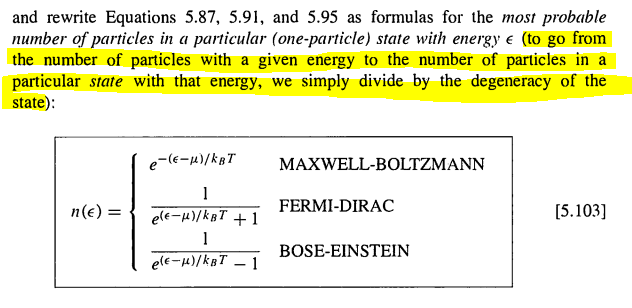The formulas in Griffiths are correct, but the explanation is pretty clumsy, because he's basically done the derivation 'in reverse'. For simplicity I'll just talk about the distinguishable particle case, but the others are similar.
The derivation in the forward direction looks like this: the Maxwell-Boltzmann distribution is the distribution that maximizes the entropy given fixed energy. Here, the entropy is defined as
$$S \sim \sum p_i \log p_i$$
and the $p_i$ are the probabilities of occupancies of each state (not each energy level!). If you carry out the constrained optimization, using a similar method to Griffiths, you'll arrive at equation 5.103.
Now, the probability of occupancy of a state only depends on its energy. Let's say that the probability of occupancy of a state at some energy is $p_n = 1/2$, and the degeneracy is $d_n = 10^6$. Then by the law of large numbers, the total occupancy $N_n$ of this entire energy level will be very close to $p_n d_n = (1/2) 10^6$. The occupancy could certainly be more or less, but the probability distribution will be peaked about this central value.
The only problem with this approach is that the definition of $S$ is a little unintuitive. So instead, Griffiths works only with occupancy numbers $N_n$, so he can just "count the number of ways" to achieve those numbers instead of dealing with the probabilities $p_n$. Then, he implicitly takes the high $d_n$ limit, so that $N_n \approx p_n d_n$, and calculates $p_n = N_n / d_n$.
The high $d_n$ limit is necessary so that the probability estimated by this ratio is accurate. For example, if $p_n = 2/3$ but $d_n = 10$, the most likely occupancy number could be $N_n = 7$. Then dividing would give the approximation $p_n \approx 0.7$. For our calculated value of $p_n$ to be good, we must take $d_n$ to infinity.
A final muddy point is that Griffiths accidentally calls the probabilities $p_n$ "the most likely occupancy numbers of a state", even though this makes no sense because $p_n$ isn't even an integer, it's a probability between $0$ and $1$. This clumsy wording is because Griffiths has swept all of the probability language under the rug in favor of occupancy numbers, but it's just not right.