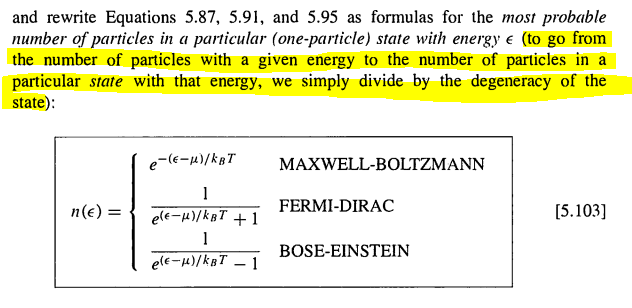This section of Griffiths Introduction to Quantum Mechanics deals with Boltzmann, Fermi-Dirac, and Bose-Einstein distributions. I don't understand this line (highlighted in yellow):

Let's talk only of Maxwell-Boltzmann here to keep it simple. Originally, we had

$$N_n=d_ne^{-(\alpha+\beta E_n)}$$

This was explained in the book to be the equation for the most probable occupation number for distinguishable particles. Then, in the image above, the author divides by $d_n$ to result in "the number of particles in a particular state with that energy", but I don't quite understand this. Could someone explain this bit in simpler terms? Or with a simple example?