When is the assumption $\nabla \cdot D = 0$ justified for a waveguide?

Question

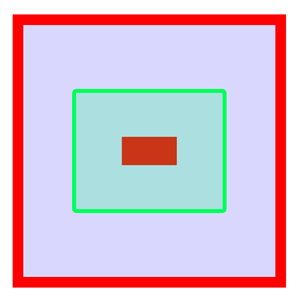
Let's say we are looking at a waveguide with a perfect electric conductor as boundary (red), filled with air and another perfect conductor (red) inside. Say this waveguide is homogeneous in the longitudinal direction and infinitely long. We can look at the transverse plane of this waveguide:

If we look at the whole plane, one can say with confidence that $$\nabla \cdot D(x,y,z) =0 $$ But if we look at the static mode transverse electromagnetic wave (TEM) and take into account only a part of this plane, say only the green area, then the formula no longer holds.

So my question is: when calculating the electrical field of a waveguide using eigenvalue equations like $ \nabla \times \nabla \times E(x,y,z) = \omega^2 E(x,y,z) $, when can one assume that $ \nabla \cdot D(x,y,z) =0 $?

I am considering a 3D case, whereas the calculation of the eigenmodes can be limited to a 2D case. For this one often sets $\nabla \cdot D=0$. And this is why I need to know the answer to my question.

Answer 1

We suppose that the inside of the inner conductor is not empty. If you have $ \nabla \cdot E(x,y,z) = 0$ on a neighborhood $V$ of the inner conductor $C_{i}$ ($C_{i} \subset V$), you have no charges in $V$. In particular, you have no charges in the conductor itself, hence the electric field is zero inside the conductor.

We suppose that $$\nabla \times \nabla \times E(x,y,z) = \omega^2 E(x,y,z).$$ It follows from the divergence free assumption and the vector calculus identity $\nabla \times \left( \nabla \times E \right) = \nabla(\nabla \cdot E) - \nabla^{2}E\ $ that $$-\nabla^2 E(x,y,z) = \omega^2 E(x,y,z).$$ Hence the field $E$ is analytical in $V$ (property of the Laplace operator).

Being zero on an open subset of $V$ (the inside of the inner conductor) and analytical on $V$, the field $E$ is zero in the whole $V$.

In conclusion, the divergence free assumption on a neighborhood of the inner conductor implies that $E(x,y,z) = 0$ in the whole waveguide, including the inner perfect conductor. Which in turn implies that in the usual case where $E$ is not zero, the divergence free assumption including the inner conductor does not hold (that is, there are some charges moving around in the inner conductor).