In circular motion is the angular velocity vector always perpendicular to centripetal acceleration?

Question

• In circular motion is the angular velocity vector always perpendicular to centripetal acceleration?

• Are there any exceptions?

• Why or why not?

Answer 1

The angular velocity vector is defined as pointing out of the paper (it is the axis about which the angular velocity rotates). It will always be perpendicular to any vector drawn on the paper.

Answer 2

The angular velocity can be defined as:

$$ \omega = \frac{\mathbf r \times \mathbf v}{|\mathbf r|^2} $$

So the angular velocity vector is always at right angles to $\mathbf r$.

If we consider circular motion, where $\mathbf r$ is the position vector from the centre of the circle, then centripetal acceleration is, by definition, always in the same direction as $\mathbf r$. That means the angular velocity is always at right angles to the centripetal acceleration.

Answer 3

Analysisng from the first point:

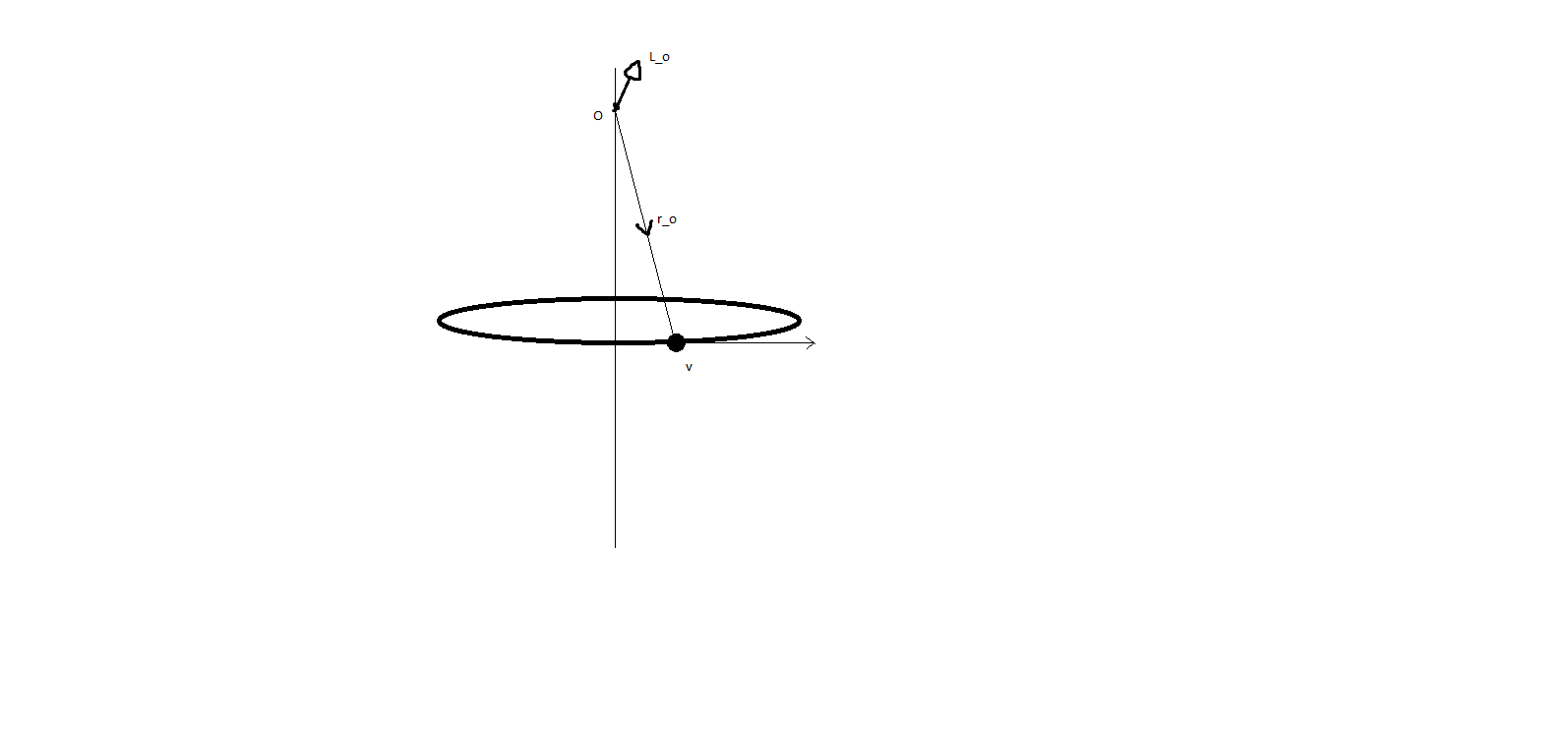
1) Assuming it is referring to planar circular motion with fixed axis, the moment of inertia tensor of the system reduces to a single scalar variable ($I_o$ suppose). In other words, we know that the angular momentum about a fixed point O in space of the particle undergoing circular motion, is given by :

$L_o = \vec r_o \times m\vec v$.There are cases, when it is not perpendicular to the centripetal acceleration.

2) For better understanding, consider the following diagram:

We chose the point O as shown. By the definition of angular momentum above, clearly, $L_o$ is a rotating vector in space, perpendicular to both $\vec v$ and $\vec r_o$ at any instant. Now, we also know that:

$\vec L_o = I_o.\vec \omega$, which implies that $\vec \omega $ is in the same direction as $\vec L_o$. Clearly, $\vec \omega$ is not perpendicular to the centripetal force in this case, which point in the radial direction in the plane of the circle.

NOTE: I may be wrong, but i could not find any flaws in my deduction. Forgive me if it is stupid, but please point out any mistake, and i will remove this answer immediately.