If Force is perpendicular to magnetic field, how do 2 magnets attract or repel?

Question

We've learnt that for a moving charge or current, magnetic field is perpendicular to the force produced. When say 2 electromagnets, which act as magnets whose reason for magnetism is not quantum mechanical but simply due to a current, are brought together, they either attract or repel depending on the orientation. But if F is perpendicular to B, how does this happen? I know I'm definitely going wrong somewhere, but cant seem to find it. Please clarify.

Answer 1

I believe you are assuming the magnetic field to have the same direction as the magnetic force. That is not correct.

• An electric field $\vec E$ is in the same direction as the electric force $\vec F_e$. We can see that directly from the formula that ties them together: $$\vec F_e=q\vec E$$ $q$ is charge. This is a direct linear relationship. The two are proportional, and the direction of one is the same as the other.

• A magnetic field $\vec B$ on the other hand is not in the same direction as the magnetic force $\vec F_m$. In fact, it is perpendicular. We again see that from the formula: $$\vec F_m=q\vec v \times \vec B$$ $q$ is a charge with speed $\vec v$. This is a cross-product; the result from a cross is always perpendicular to both vectors. $\vec F_m$ is perpendicular to both the motion direction $\vec v$ of this charge, and to the magnetic field $\vec B$. This is a 3D-consideration. Sometimes you might see another version of this formula, though, which is the case when many electrons moving - in other words, in the case of a current $\vec I$: $$\vec F_m=l\vec I \times \vec B$$ $l$ is length of the wire that carries the current. Directions are the same, as you can see; force is perpendicular to these moving electrons (the current) and to the field.

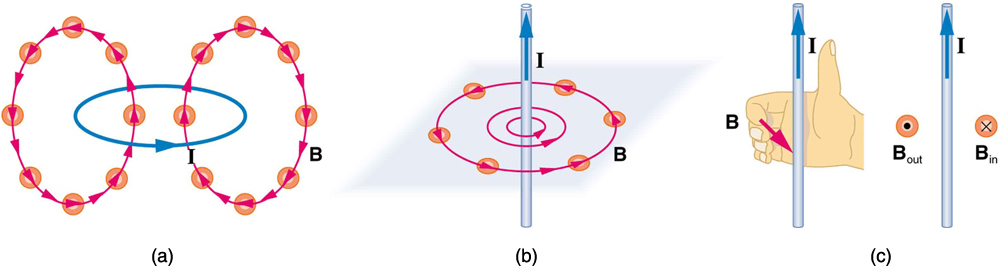
Have a look at this illustration (source):

Charge moving through a wire as a current, and they set up a magnetic field. In each case, the magnetic force is perpendicularly towards the wire or blue line. Materials being attracted are not being attracted "around" the wire, but directly into it. The force is perpendicular, even though the field is around the wire.

This is the way, people in physics use the concept of magnetic field lines. It was chosen so, since it describes the situation well - for example, you see the cirular manner, they are drawn in; magnetic field lines are never-ending, but circular. This namely describes that the magnetic force is equal in size everywhere on this circular path, which is the case in reality. But even though it is the same size on the line, it is not the same direction. That is a fundamental difference from the maybe more intuitive concept of electric field lines.

Many such field lines can be googled for objects, wires, setups, magnets etc. There are many examples out there. And every time you see them, think of the force as being perpendicular to those lines. Not along them!

I hope this explanation gives some insight and helps out the confusion a bit.