Electric field outside wire with stationary current

Question

Consider a conductor of arbitrary structure where a stationary current flow, that is

$$\nabla \cdot \vec{j}=0$$

I did not find on textbook clear explanations on two facts:

• How is the electric field outside the conductor made exactly? (in particular the normal component).
• Are there charge densities on the surface of the conductor?

---

I know that, inside the conductor $$\vec{j} = \sigma \vec{E}$$

($\sigma$ is conductivity). And that the tangential component of $E$ is always conserved.

Moreover, since the normal component of $\vec{j}$ is conserved (this follows from $\nabla \cdot \vec{j}=0$), and $\sigma_{ext}=0$ we have that $$j_{n,int}=j_{n,ext}=\sigma_{int}E_{n,int}=\sigma_{ext}E_{n,ext}=0$$

That is, there is no normal current on the surface.

But what is $E_{n,ext}$ ? I cannot conclude nothing from here.

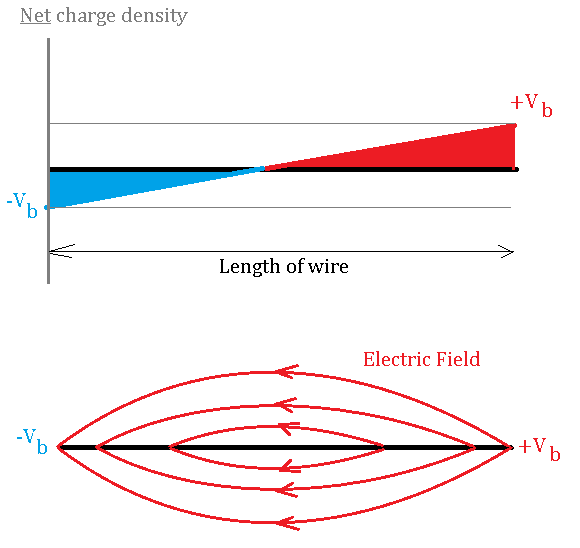
If there were surface density of charges (named $\varsigma$), then is would be $$E_{n,ext}=\frac{\varsigma}{\epsilon_0}$$ But I'm not sure about the presence of charges.

---

To sum up in my view it should be $$\begin{cases} E_{t,ext}=E_{t,int}=E_{int}=\frac{j}{\sigma} \\ E_{n,ext}=\frac{\varsigma}{\epsilon_0} \\ E_{n,int}=0 \end{cases}$$

Is this correct or are there any mistakes?

Answer 1

The question seems more tricky than I first guessed, see for example this question or that question. I suggest the following approach to stay on the safe side.

Let us suppose that the conductor is an infinite cylinder, the current $\vec{j}$ being uniform inside the conductor.

We suppose also that your assumption about a surface density of charges $\varsigma$ holds, that is, the (outward) normal component of the electric field is given by $$E_{n,ext}=\frac{\varsigma}{\epsilon_0}.$$ It corresponds to the exterior electric radial field $$\vec{E}_r(r)=\frac{\varsigma a}{\varepsilon_0 r}\vec{e}_{r}$$ where $a$ is the radius of the conductor, $r$ is the distance to the axis of the conductor and $\vec{e}_{r}$ is the usual unit vector perpendicular to the axis of the conductor.

Then we have to add the component $\vec{E}_z(r)$ associated to $\vec{E}_{t,ext} = \frac{j}{\sigma}\vec{e}_{z}$ where $\vec{e}_{z}$ is the unit vector along the axis of the conductor, supposed to be the $z$ axis. The magnetic field $\vec{B}$ does not depend on time, hence it follows from Maxwell's equations that $\Delta \vec{E}=0$ outside the conductor. Indeed, we have $$\Delta \vec{E}=\nabla (\nabla.\vec{E})−\nabla \times (\nabla \times \vec{E})=0−\nabla \times\frac{\partial \vec{B} }{\partial t}=0.$$ As $\Delta \vec{E}_r=0$ and $\vec{E} = \vec{E}_r+\vec{E}_z$, we also have $\Delta \vec{E}_z=0$. One solution is simply given by the constant field $$\vec{E}_z(r)=\frac{j}{\sigma}\vec{e}_{z}.$$ There are other solutions involving the log function, but these are not bounded when $r \to \infty$, so you may not want to consider them.

Finally, the total exterior electric field is given by $$\vec{E}(r) = \vec{E}_r(r)+\vec{E}_z(r) = \frac{\varsigma a}{\varepsilon_0 r}\vec{e}_{r}+\frac{j}{\sigma}\vec{e}_{z}.$$

Answer 2

Just my opinion on why I think there must be a net electric field around a wire with steady current.

To have a steady current in a wire, there must be a voltage gradient through the wire (which is why I wouldn't prefer considering an infinite wire length with infinite resistance).

Imagine a straight wire simply connected to two identical batteries at each end. The current is steady and there is a voltage gradient. Voltage (relative to ground) is zero in a theoretical point in the middle of the wire and gradually rises in - and + values to the right and left ends of the wire.

Let's consider the half of the wire with negative voltage.

The voltage gradient should be caused by the free electrons compressed to a density more than they have in their rest state (which is equal the charge density of the positive ions/nuclei). This increased density is due to the resistance of the wire. You need to push electrons to overcome the resistance and by pushing, you squeeze them into higher density thus having more negative charges than their rest state in which all + and - charges cancel out to zero net electric field.

For the positive voltage half of the wire, it's the same except you dont compress but vacuum the electrons, rarifying them. The voltage gradient here is caused by the excess of positive ions. The density increases more and more to the end of the wire, eventually equaling the battery voltage there.

So, there are excess of negative and positive charges at each half. This should cause a net static dipole electric field around the wire. And how much current is produced by this voltage gradient? It depends on the resistivity and length of the wire.

A hydrodynamic analogy: You have a pump with a certain pressure supply. There will be a pressure gradient determined only by the pump pressure and pipe length. So you will have a little denser, compressed water than you have in the rest state. How much flow we get, depends on the hydrodynamic resistance on the water.