0
$\begingroup$

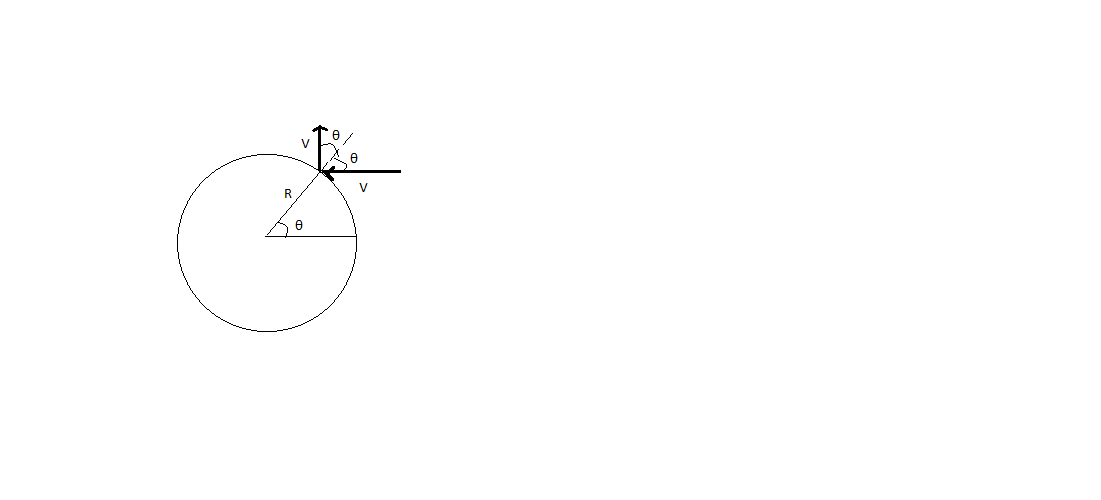
A cylinder of mass M and radius R moves with speed V in a direction perpendicular to its axis, and elastically collides (non-head on) with a particle of mass m at rest the mass M is much larger than m. The book answers this by viewing the motion from the reference frame of the cylinder and magically assumes the following (in the frame of the cylinder) enter image description here

To me this seems to be a very bold assumption. The momentum is not conserved in this frame so I really don't know how it came up with this answer. How is it possible that object maintain the same speed V after collision and why the particle does not travel radially away from the center of the cylinder instead, since that where the collision force is directed at for the particle. I can see why would the particle reverse direction if this was 1 dimensional, but I can't see why would the speed be the same and in this particular direction for the 2D case.

Improve this question
$\endgroup$
1
  • $\begingroup$ update: I understand that the sphere frame could be treated as the CM frame since its mass dominates, so the particle's velocity retain the same magnitude after the collision because the energy is also conserved in the CM frame. However, I still don't get how the angles are set up this way. $\endgroup$
    – NegativeTension
    Commented Nov 1, 2016 at 22:34

3 Answers 3

Reset to default
1
$\begingroup$

Momentum is conserved but the recoil velocity of the cylinder is so small (because $M \gg m$) that it can be neglected and the cylinder can be considered to be stationary. It is the same when we consider balls bouncing from the ground or the side of a building : the recoil velocity of the Earth is insignificant.

Improve this answer
$\endgroup$
0
$\begingroup$

Remember momentum is a vector and speed is a scalar. After the collision momentum could change because the velocity vector changed but the magnitude could have stayed the same.

Improve this answer
$\endgroup$
2
  • $\begingroup$ So the conservation of energy dictates that the magnitude of velocities is the same before and after the collision. but how about the angles why isn't the particle travelling radially away from the sphere after the collision? $\endgroup$
    – NegativeTension
    Commented Nov 1, 2016 at 22:00
  • $\begingroup$ @NegativeTension : ... Because it wasn't travelling radially towards the sphere before the collision! $\endgroup$
    – sammy gerbil
    Commented Nov 2, 2016 at 17:04
0
$\begingroup$

Because the cylinder is much more massive than the particle, we can consider it to be stationary and fixed with respect to the particle. Now what does the particle see?

Where the (point) particle hits the cylinder, it sees essentially a flat surface (the tangent to the cylinder). And so it will behave like a point particle encountering an infinitely massive flat surface: it will bounce off of it. Its velocity component perpendicular to the surface of the cylinder is "flipped", while its velocity component parallel to the surface of the cylinder (parallel to the tangent at that point) is conserved. Hence it bounces off in that particular (incidentally non-radial) direction.

In other words, it becomes clearer if you draw the tangent to the cylinder at the point of contact. The particle sees a flat surface parallel to that tangent.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.