I like to answer this question after reading the comment of @Diracology.
Before the unification between the weak and e.m. force broke, very early in the history of the Universe [when the temperature was about $10^{15}(K)$], the field connected to the Mexican Hat potential (present at every point in space) was zero and corresponding to a maximum, constant energy throughout space.
When the temperature dropped the unification between the two forces broke (after which the weak and e.m. force became two distinguishable forces), because the zero field connected to the MH potential came to lie on a random point on the circle at the rim of the MH. Each of these points corresponded (corresponds) to the same vev. It is said in another answer:
If the Higgs field had different values at different points in space i.e., if it had a spacetime variation, then the gradient term would give a positive contribution to the Hamiltonian, and hence, the total energy will not be minimized.
I don't think this is a real physical explanation, though. It just gives an explanation with the aid of math, which comes after the physics. I mean, the fact that the field doesn't fall at the same place on the rim of the hat everywhere is translated to in the "language" of math. The field doesn't "know" about the math and just falls in a configuration with least energy, which is one that has a non-random variation throughout space.
Just look at this picture:
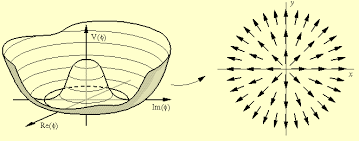
Left one obviously sees the MH potential, while on the right one can see the cross-section of a cosmic string. The arrows represent the omnipresent Higgs field. The diameter is about the same as that of a proton [$1(fm)$]. Inside the string, the same conditions are present as the conditions in the early Universe, just before the electroweak symmetry breaking so the temperature inside the string is about $10^{15}(K)$. The configuration of the Higgs field has, in this case, a zero gradient (it may seem that the gradient isn't zero, as the electric classical field around an electron, but in this case the absolute value of the field, unlike its direction, has the same value everywhere), which means the total energy is minimized.
This one example shows that the Higgs field falls does not have to fall in the same ground state at all points in space. Though such a string (of which it is thought that there is one in each Hubble volume) has (probably) never been observed, there is a theoretical possibility that it exists. When these cosmic strings (not to be confused with the strings from string theory) exist and one passes the Earth closeby, I wouldn't be able anymore to write this down (let alone if one passes **through the Earth).
There were theories that postulated two-dimensional versions of the cosmic string (textures), but these were ruled out by experiment.

