In massive atoms, the 1s and other inner electrons travel at relativistic speeds. Einstein's relativity equation predicts the mass of these electrons should increase. Is the measured mass of a mercury atom larger than 'expected' (after accounting for nuclear binding energy, etc.) due to the relativistic speed of inner electrons?
$\begingroup$
$\endgroup$
5
-
1$\begingroup$ The electrons don't "move" at relativistic speeds. That's a classical approximation. To see this very clearly, they would decay ridiculously fast through radiation by accelerating charge (circular motion is acceleration). $\endgroup$– QuantumFoolCommented Sep 28, 2016 at 4:14
-
$\begingroup$ That said, quantum field theory lets you deal with relativistic quantum mechanics. $\endgroup$– QuantumFoolCommented Sep 28, 2016 at 4:14
-
$\begingroup$ The notion of relativistic mass is somewhat obsolete, it's the total energy and momentum that get modified in relativity. Regarding your question, electron is almost 2000 times lighter than the proton, and the addition to its energy due to relativity is even smaller, so I would guess the effect is extremely small, maybe unmeasurable $\endgroup$– KosmCommented Sep 28, 2016 at 4:15
-
$\begingroup$ Yes, relativistic affects can be detected. For example rsta.royalsocietypublishing.org/content/320/1553/71 and dx.doi.org/10.1088/0953-4075/23/19/010 Quantum mechanics explains why differently though. $\endgroup$– MaxWCommented Sep 28, 2016 at 4:59
-
$\begingroup$ This is quite a good summary profmattstrassler.com/articles-and-posts/… $\endgroup$– user108787Commented Sep 28, 2016 at 6:37
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
You are stuck at the Bohr model of the atom, where electrons are supposed to exist in planetary orbits around the nucleus and a speed can be calculated from the energy of the energy levels they occupy.
This is no longer a valid model of the atom. The quantum mechanical solutions of the Schrodinger equation for the atom reproduces the Balmer etc series that was the success of the Bohr model, but has the electrons in orbitals , not in orbits, and thus no speed can be assigned to the individual electron.
Quantum mechanics predicts probability densities for particles.
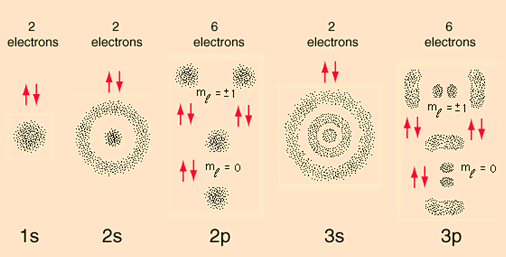
Rough sketches of the electron density for the first three shells of the hydrogen atom can give an impression of the constraints that govern the buildup of the periodic table. The limits on the occupation of the subshells arise from the quantum numbers for the atomic electrons and their relationship to each other. These sketches arise from the hydrogen wavefunctions which map the electron density.
Each dot is ticked from a calculation of the complex conjugate square of the wavefunction, which gives the probability of finding the electron in that d(Volume) cube in space.
The energy balances are all taken care of in the calculation of the individual points of each orbital, within the mathematics of the quantum mechanical equation. There is no way of getting an orbit for the electrons that would allow to calculate a speed. Even though one can get a speed from the energy level the electron occupies, it is an artifact , because the basic level is quantum mechanical and probabilistic .
You might have an argument that since the energies involved with respect to the mass of the electron would give the electron a relativistic speed if it were free, then one should use the relativistic quantum mechanical equations to get at the wavefunctions. The comment to the question which points out the difference between the mass of the electron and the mass of the nucleus its orbital has as a focus would hold, too much calculational trouble for a very tiny effect on the probability distributions.
$\begingroup$
$\endgroup$
Not really. As in Anna V's Answer one needs to be wary of thinking along the lines of the Bohr model, but the total energy of each electron is bound to the atom however you may think of the electron. Therefore, this total energy $E$ leads to the same increase in the system's rest mass $E/c^2$ independently of whether you think of the electron as an energetic point particle or as an orbital state - rest mass is not an additive property like energy (i.e. a system's rest mass is almost never the sum of the rest masses of its constituents), so that one really can't "expect" a rest mass of a composite system to be related to the rest masses of the system's constituents in a simple way without taking account of the constituents' full states. A simple illustration of this statement is a system of two counterpropagating photons: there is no center of mass rest frame for either of the rest-mass-less constituents, but the system as a whole does have a center of mass rest frame and the system's rest mass is $2\,E/c^2$, where $E$ is the energy of each constituent photon.
answered Sep 28, 2016 at 6:19