As I've been trying to wrap my head around the principles of decoherence and quantum behavior I am left wondering why fundamental particles are 'allowed' to exhibit quantum properties even in ideal conditions ( close to absolute zero and in a 'box'). If a particle/photon behaves in a probabilistic superposition state as seen in the double slit experiment then wouldn't we expect the multiple coincidental states of the particle to interact with each other and thus cause decoherence? This would in turn lead to an innate instability of any system above a zero point energy thereby making quantum properties not identifiable at all..but indeed we do have verifiable and replicable evidence of quantum mechanics.
-
$\begingroup$ I'm not exactly sure what the question is - decoherence requires interaction with some environment that produces the decohering interaction. If there's none, no decoherence happens. $\endgroup$– ACuriousMind ♦Commented Sep 29, 2016 at 17:14
-
$\begingroup$ if they "exhibit" then they are somehow measured and decoherence happens. Could you please provide a precise example ? $\endgroup$– user46925Commented Sep 29, 2016 at 17:19
-
$\begingroup$ "...wouldn't we expect the multiple coincidental states of the particle to interact with each other and thus cause decoherence? " No, and without an example calculation showing why you think that should happen, it's hard to write an answer to this question. $\endgroup$– DanielSankCommented Sep 29, 2016 at 17:34
-
$\begingroup$ I was under the assumption that a particle could indeed interact with itself as in the double slit experiment ( causing the probabilistic interference pattern) instead of being required to interact with its 'environment' to cause decoherence. Wouldn't another wave function of an individual particle be considered 'the environment' ? $\endgroup$– Cato1974Commented Sep 29, 2016 at 17:47
1 Answer
If a particle/photon behaves in a probabilistic superposition state as seen in the double slit experiment then wouldn't we expect the multiple coincidental states of the particle to interact with each other and thus cause decoherence?
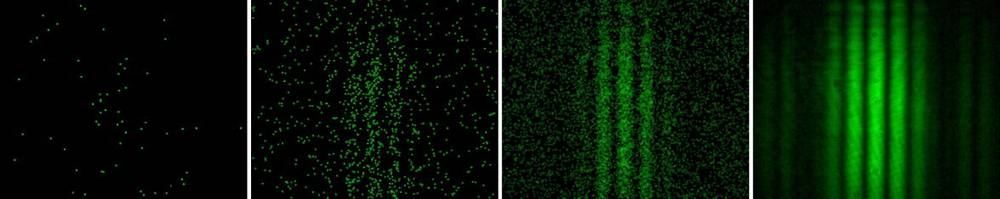
There is a misunderstanding in this statement. In the single photon at a time double slit experiment, a single photon appears as a dot on the "screen", it is not spread out in the interference pattern.
Single-photon camera recording of photons from a double slit illuminated by very weak laser light. Left to right: single frame, superposition of 200, 1’000, and 500’000 frames.
It is the accumulation of photons that shows an interference pattern, the probability density distribution for the quantum mechanical problem "photon impinging on two slits".
The probability density distribution is the square (Psi*Psi) of the quantum mechanical wavefunction.
In addition, superposition of wavefunctions can give interference effects, but there is no interaction, as in two laser beams interfering. There is no measurable photon-photon interaction, but there is superposition of the wavefunction of the two photons, and the sum gives the interference terms in the probability density distribution (Psi1+Psi2)*(Psi1+Psi2).
-
$\begingroup$ Thanks very much Anna. One further question: How would this line of thinking apply to an electron in a probability distribution cloud around a nucleus? Wouldn't we expect the multiple 'states' of the electron to interact with each other causing decoherence? Or is it that we are purely dealing with a mathematical probability distribution rather than the electron truly being in two places at once. $\endgroup$– Cato1974Commented Sep 29, 2016 at 18:37
-
$\begingroup$ Again it is the difference between a wave function and a probability distribution. If you go to the individual constituents of the atom, and want to create the state it would require second quantization, the electron field and the proton fiel (for a hydrogen atom. The proton is a very complicated entity with gluons and quarks, but let us approximate it with a field). There you could define the wave function as a superposition of all the creation and annihilation paths taken by the electron and proton . These would be in superposition. The probability of finding an electron would be the square $\endgroup$– anna vCommented Sep 30, 2016 at 3:53
-
$\begingroup$ of the wavefunction , which is what one can see here physics.stackexchange.com/questions/282843/… calculated as points . A measurement realizes the probability. The constituents exist in the wavefunction, in the mathematical model we have of physics at particle level, one needs a measurement to define interactions. $\endgroup$– anna vCommented Sep 30, 2016 at 3:56