Rotation of superfluid

Question

I have two questions related to rotation of superfluids. Firstly, what is the main reason that superfluid cannot rotate as a whole object ? (I found that it is true in Landau's Statistical Physics but without explanation.) Second question is the following. Suppose we have a rotating helium and then we cooling it in such a way that it causes transition into superfluid state. What happens with the rotation degrees of freedom ? How to describe such a phase transition ?

Answer 1

Particle on a rotating ring

For further discussion purpose, let's considere the dynamics of a quantum particle on a $r_0$ radius rotating circle at a constant angular velocity $\mathbf{\Omega}=\Omega\,\hat{e}_z$ . In cylindrical coordinates, we fix $z=0$, and we have the azimuthal angle $\theta$, which is the "good" degree of freedom describing the dynamics.

One can compute the hamiltonian of the system in the rotating frame, taking into account the angular momentum $\mathbf{\mathcal{L}}$ of the particle : $$ \mathcal{H}=-\frac{\hbar^2}{2m}\Delta-\mathbf{\Omega}\cdot\mathbf{\mathcal{L}}=-\frac{\hbar^2}{2m\,r^2_0}\frac{\mathrm{d}^2}{\mathrm{d}\theta^2}+\mathrm{i}\hbar\frac{\mathrm{d}}{\mathrm{d}\theta}=\frac{\hbar^2}{2m\,r^2_0}\left(\mathrm{i}\frac{\mathrm{d}}{\mathrm{d}\theta}+\frac{\Omega}{\Omega_c}\right)^2-\frac{1}{2}m\Omega^2r_0^2 $$ where $\Omega_c=\frac{\hbar}{m\,r^2_0}$.

It is easy to see that the spectrum is : $$E_n=\frac{\hbar^2}{2m\,r^2_0}\left(n-\frac{\Omega}{\Omega_c}\right)^2,\quad\forall\,n\in\mathbb{Z} $$ For simplicity, let fix $\Omega>0$ for the following. This tells you that the ground state $\Psi_g$ of the system depends on $\Omega$ such that : $$ \text{for}\;\Omega<\Omega_c/2,\quad\Psi_g(\theta)=\frac{1}{\sqrt{2\pi}} $$ $$ \text{for}\;\Omega_c/2<\Omega<3\Omega_c/2,\quad\Psi_g(\theta)=\frac{1}{\sqrt{2\pi}} e^{\mathrm{i}\theta} $$ $$ \text{etc}... $$ In each case, one can calcultate the associated velocity : $$ \textbf{v}=\frac{n\hbar}{mr_0}\,\hat{e}_\theta $$

Dragging a fluid

As a first experiment, we can considere a gas on a thin ring initially at rest. Then we accelerate the ring to a constant angular velocity $\mathbf{\Omega}=\Omega\,\hat{e}_z$. Let say that the ring as some roughness in its inner surface which can interact with the gas.

If the gas in a classical (viscous) gas, it can be shown that the interaction between the gas and the inner surface of the ring will drag the gas so that its velocity field will be : $$ \textbf{v}(\textbf{r})=\mathbf{\Omega}\times\textbf{r} $$

Now, if the gas is a quantum fluid (superfluid), zero viscosity implies that the gas does not interact with the ring anymore. And for a slow rotation $\Omega\ll\Omega_c$, we see that the ground state of the system as zero velocity, meaning that although the ring is rotating, it cannot drag the fluid which stays at rest.

Probing the classical/superfluid transition

As a second experiment, let us assume that we managed to rotate the superfluid with $\Omega>\Omega_c$, meaning the initial velocity field of the gas is given by : $$ \textbf{v}=\frac{\hbar}{mr_0}\,\hat{e}_\theta $$ What happens if we suddenly stop the rotation of the ring, setting $\Omega=0$?

If the fluid is classical (viscous), interactions between the fluid and the ring leads to zero velocity field and the fluid will stop rotating.

For a superfluid however, there is no viscosity leading to a possible stop of the fluid although the ground state of the system as a zero velocity field, as shown in the previous experiment. Such feature is typical of metastability, showing here the existence of permanent currents. This also implies that such system should show an hysteresis behavior.

Indeed, for $\Omega=\Omega_c/2$, the system has a degenerate ground state such that : $$ E_0(\Omega=\Omega_c/2)=E_1(\Omega=\Omega_c/2) $$ meaning that if we follow a cyclic scheme crossing that point for the rotation of the ring $$ \Omega=0\rightarrow\Omega>\Omega_c/2\rightarrow\Omega=0 $$ we can produce an hysteresis curve of currents. Reproducing such cycle for different temperature of the fluid should allow you to probe the superfluid transition (by probing the existence of permanent currents).

An example of real life experiment

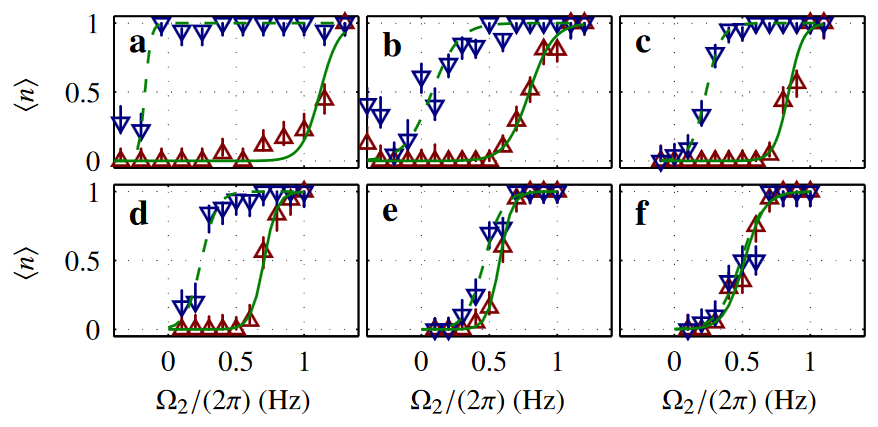
This hystereris behavior has been observed (Eckel et al., Nature, 506.7487 p200-203 (2014)) using a Bose-Einstein condensate of ultracold atoms trapped in a ring shaped dipole trap. They could excite the rotation of the condensate by pushing the atoms with a laser beam in rotation.

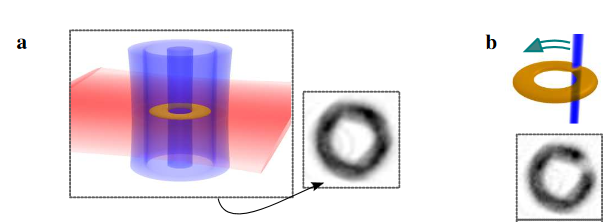
With time of flight experiment, they could measure the circulation, i.e. the $n$ index, of the momentum distribution of the atoms as a function of $\Omega$, thus showing an hysteresis.