In general, a mixed wave of the form
$$
f(x,t)=\cos(x-t)+r\cos(x+t)
$$
will not have nodes, at least in the sense of points $x_0$ for which $f(x_0,t)\equiv 0$ for all times $t$.
In general, there's relatively little to say beyond what the picture will convey:
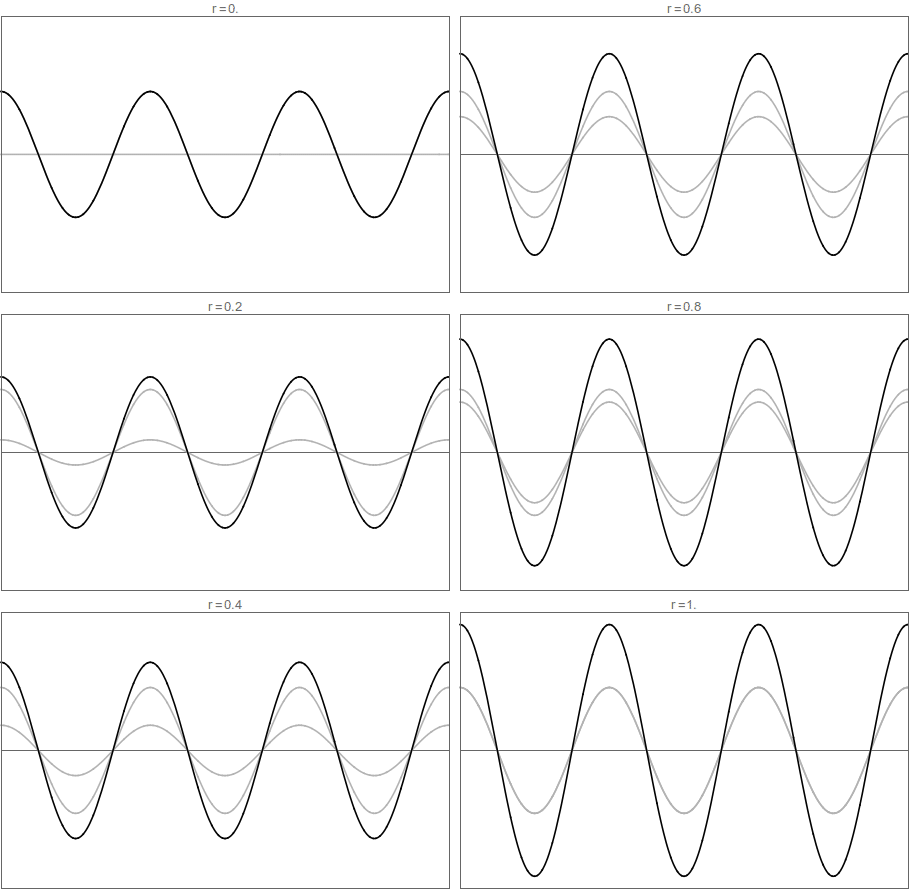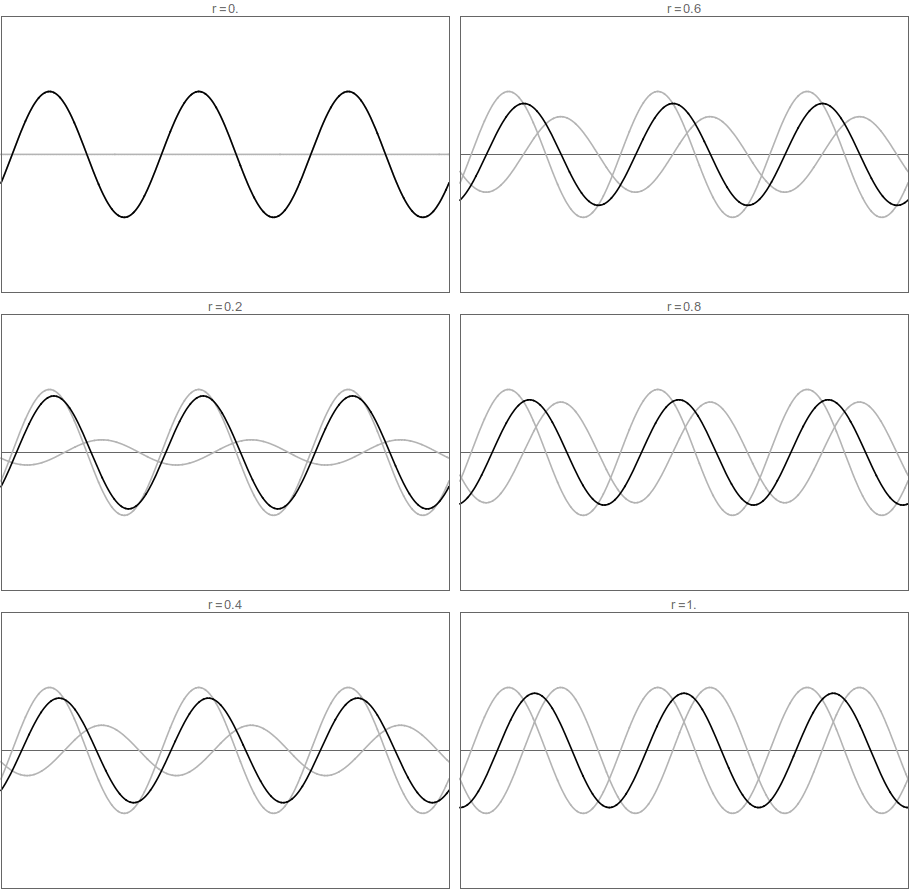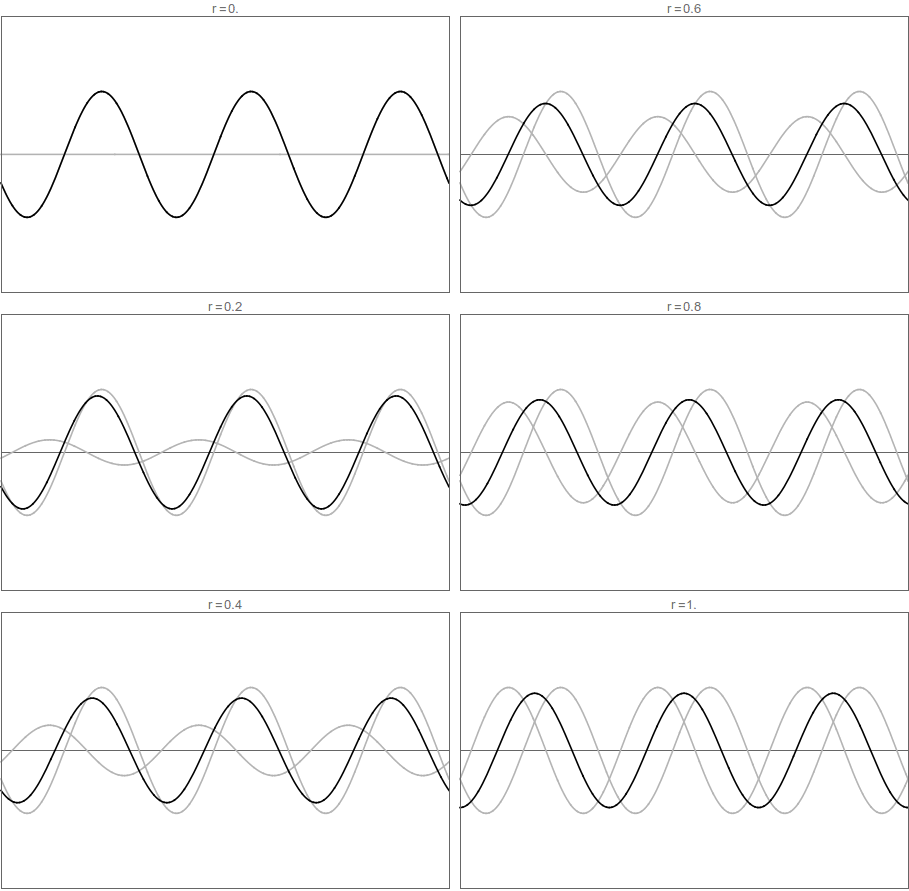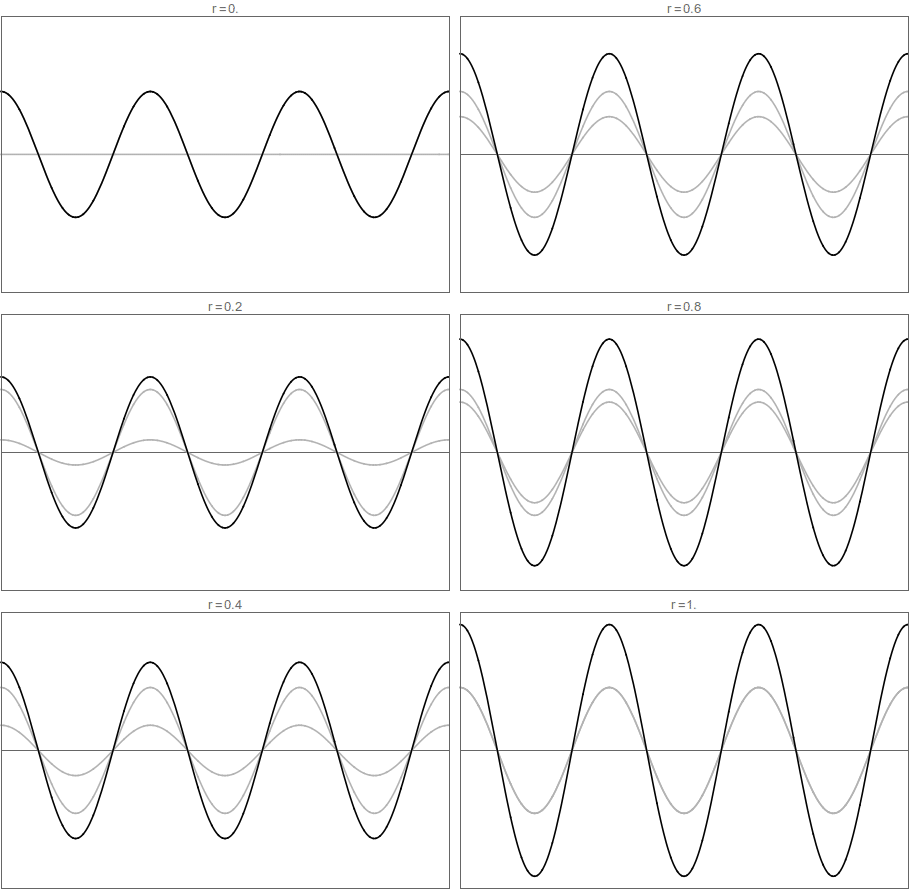
As you turn $r$ up from 0 to 1, you first start inducing modulations into the amplitude of the wave, which increases the total energy density but reduces the energy flux. Towards the end, around $r=0.7$, there is still some 'movement' of the wave, close to the times when the standing wave amplitude is small, but otherwise the pattern looks much more like the standing wave.
In terms of energy, there is a smooth increase of the total energy density coupled with a smooth decrease of the energy flux all the way down to zero. At relatively high $r$, most of the energy is confined within each 'loop', so far as they can be defined, but there is still some transmission between loops.
Depending on which regime you're in, it can be more or less helpful to decompose the wave in terms of two counter-propagating travelling waves, as above, or as a sum of a travelling and a standing wave,
$$
f(x,t)=(1-r)\cos(x-t)+2r\cos(x)\cos(t),
$$
which looks something like this:
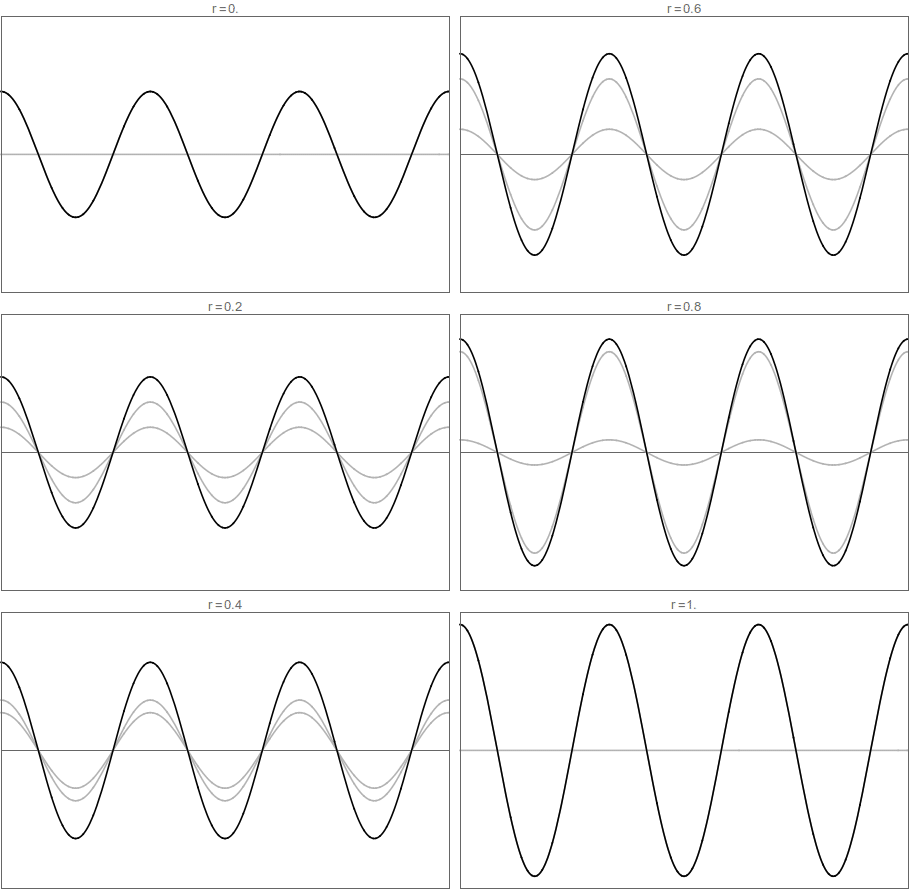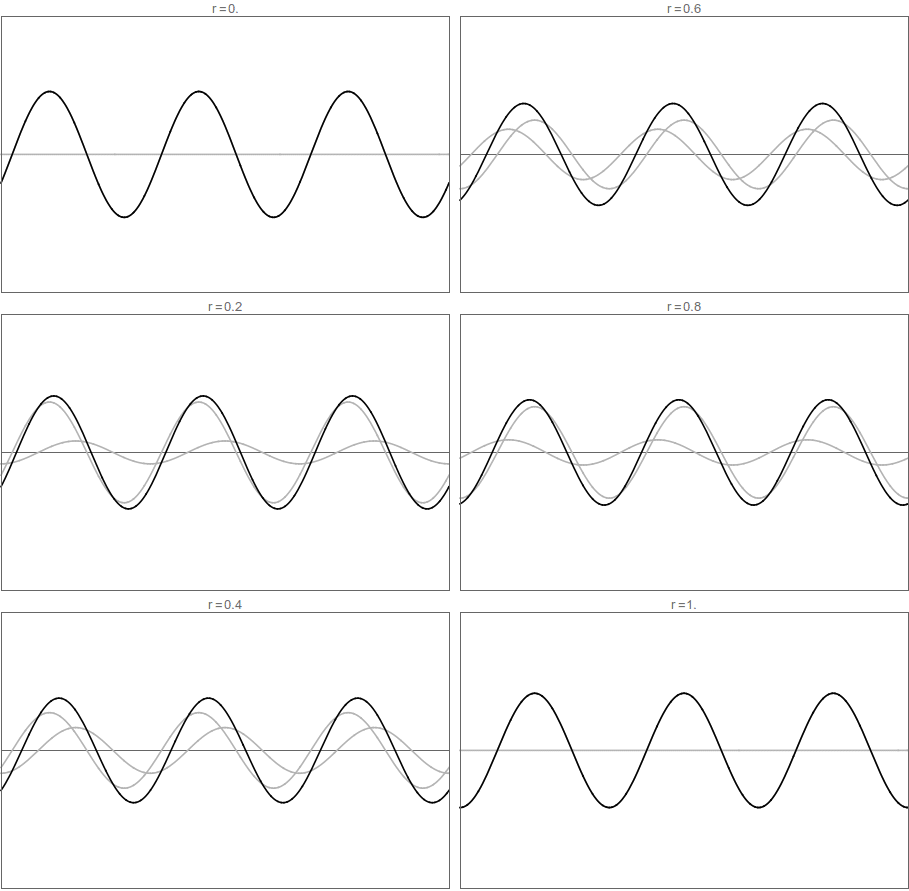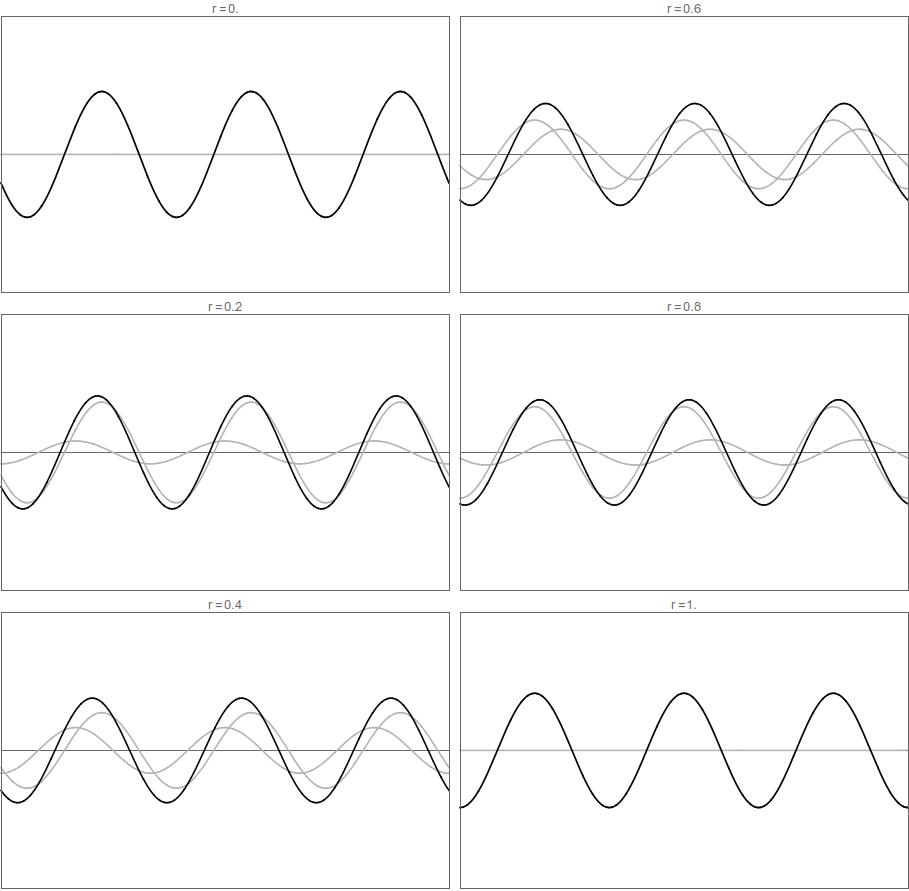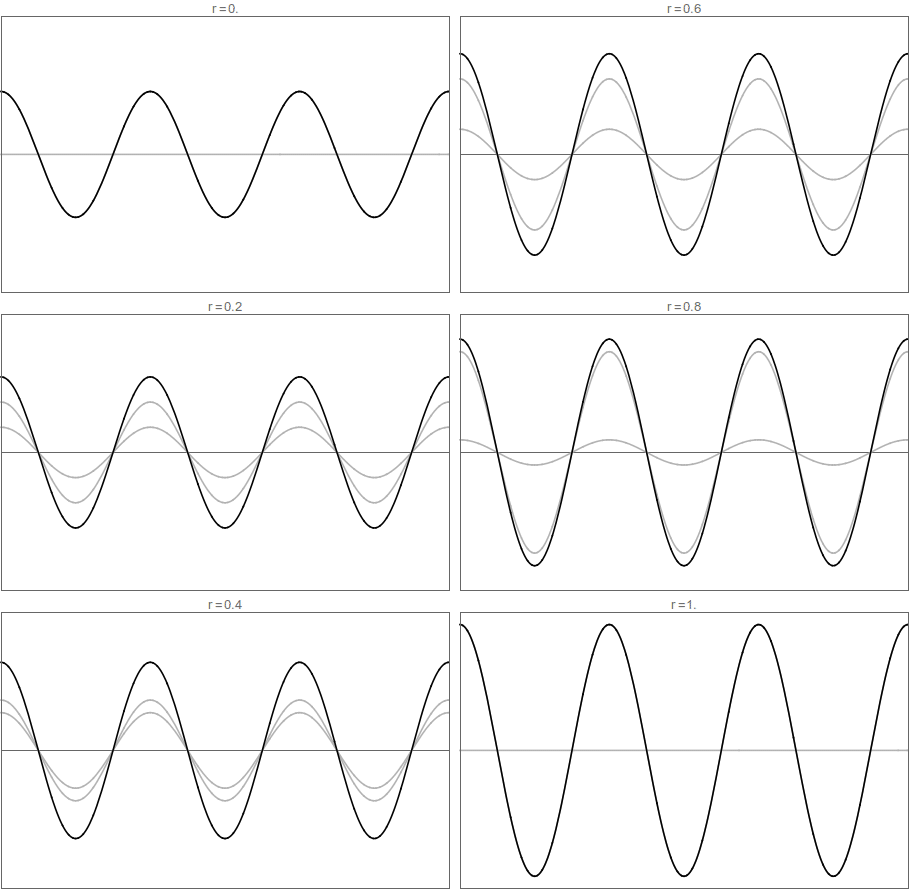
In particular, note that near the zeroes of the standing-wave component at mid-to-high $r$, the travelling wave 'carries' the antinodes to the right by half of one spatial period, which is precisely the behaviour needed to smoothly match between the uniform movement of the maxima at $r=0$ and the sudden movements by $\pi$ of the (positive!) maxima at $r=1$.


