quantum clone of orthogonal quantum states

Question

I am a little bit confused about the no-cloning theorem for two orthogonal quantum states.

In Nielson&Chuang page 24-25, it states that an unknown state $|\phi\rangle$ cannot be copied since $|\phi\rangle|0\rangle$ cannot become $|\phi\rangle|\phi\rangle$ unless $|\phi\rangle =|0\rangle$ or $|1\rangle$.

However in page 530 it says that two orthogonal states $|\phi\rangle$ and $|\psi\rangle$ can be copied, with data input either $|\phi\rangle$ or $|\psi\rangle$ and target prepared in $|0\rangle$.

my question is: does copy of this orthogonal state require target state $|0\rangle$ to be orthogonal to $|\phi\rangle$ or $|\psi\rangle$? Are $|\phi\rangle$ and $|\psi\rangle$ known states (just do not know which we will receive to copy)?

How can I implement such copy? Can I do so without a measurement?

Answer 1

No, $|\phi\rangle$ doesn't need to be orthogonal to $|0\rangle$. Neither does $|\psi\rangle$. They only need to be orthogonal to each other.

Example

Suppose you know that you will be given a state $|\omega\rangle$ that will either equal $|\phi\rangle = \frac{1}{\sqrt 2}|0\rangle + \frac{1}{\sqrt 2}|1\rangle$ or equal $|\psi\rangle = \frac{1}{\sqrt 2}|0\rangle - \frac{1}{\sqrt 2}|1\rangle$. Your goal is to create a circuit that will produce the state $|\omega\rangle|\omega\rangle$ in both cases.

Because the possible states are orthogonal, it's easy. Apply a transform that sends each possible input to a computational basis state, CNOT onto a zero'd qubit, and transform back.

Here's a circuit that performs the desired cloning:

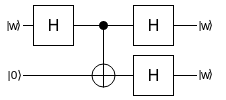
If the possible states were different, you'd need to change the circuit accordingly.

You could also just perform a measurement in the basis defined by $|\phi\rangle$ and $|\psi\rangle$. That would tell you which state you had, and then you could just produce more of those states.

KEEP IN MIND this only worked because:

1. You knew the possible states.

2. The possible states were mutually orthogonal.

If either of those conditions isn't met, you won't be able to perform the cloning. You either won't be able to figure out the basis transform to use, or there will be cross-talk between the two cases.

Summary

does copy of this orthogonal state require target state |0⟩|0⟩ to be orthogonal to |ϕ⟩|ϕ⟩ or |ψ⟩|ψ⟩?

No.

Are |ϕ⟩|ϕ⟩ and |ψ⟩|ψ⟩ known states (just do not know which we will receive to copy)?

Yes.

How can I implement such copy?

With a basis transform and a CNOT onto a zero'd qubit. Or with a measurement.

Can I do so without a measurement?

Yes.