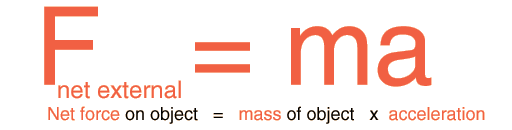Assuming mass doesn't change, force is defined as mass * acceleration. Acceleration is the change in velocity as time changes. How is it possible then to exert a force on an object that doesn't move? If velocity doesn't change, then acceleration must be 0.
$\begingroup$
$\endgroup$
2
-
$\begingroup$ As Chester said, the net force is mass times acceleration. Say you try to push an object on the ground but it doesn't move. As you exert a force on the object, the ground exerts a frictional force on the object resisting motion. Since the object doesn't accelerate, that means the vector sum of the two forces is 0. $\endgroup$– Ameet SharmaCommented May 3, 2016 at 1:52
-
$\begingroup$ "force is defined as mass * acceleration" Force is defined as first law of Newton. $\endgroup$– lucasCommented May 3, 2016 at 7:25
Add a comment
|
4 Answers
$\begingroup$
$\endgroup$
It is only NET force that is equal to mass times acceleration, not each individual force. You need to take the vector sum of the forces acting on a body to get the net force. It is possible to exert a force on a body that doesn't move if the resultant of that force together with all the other forces acting on the body sum to zero. (P.S. Michigan....GO BLUE)
answered May 3, 2016 at 0:35
$\begingroup$
$\endgroup$
force is defined as mass * acceleration.
From the well known hyperphysics web site:
The net external qualification is crucial.
answered May 3, 2016 at 3:35
$\begingroup$
$\endgroup$
Take the help of Newton's first law which says that an object would be at rest or would move at a constant velocity if there is no net force acting on the body. It is possible to exert force on a stationary body because force is a push or pull. Now, if this force is less than or equal to the frictional force or any other force which acts in the opposite direction of the applied force, then body won't move, because there is no net force on the body and remember forces like frictional force are self adjusting means that they can exert a same force as applied force in opp. direction unless the applied force crosses their maximizum value and we also can only find the maximum value for these forces, hence if the applied force is greater than these forces the the body would move.
$\begingroup$
$\endgroup$
$ F=m×a $ is not a definition; it is one form of Newton's 2nd Law of Motion. What it means is if a net force, $ F $, is applied to an unconstrained mass, $ m $, the mass will move with a motion that instantaneously has an acceleration in the same direction as the applied force, with magnitude $ a $.
If there is only one force, let us say gravity, and you let go of a rock of mass $ m $ at time $ t=0$, then the left hand side of the equation will be replaced nby Newton's Universal Law of Gravitation, with the masses being that of the earth, $ M $, and that of the rock, $ m $, and the distance being the radius of the earth, $ R $, plus the additional height to where the rock is being held, $ z $.
Then we have $ F= G× M×m/(R+z)^2=m×a $.
After dividing through by $ m $, which appears as a common factor on both sides, one is left with $ a $, the acceleration of the rock, due to the local gravitational force a distance $ z $ above the surface of the earth. As this doesn't change much for small distances, it is often taken as a near constant, designated $ g=9.8 m/s^2$.
Having solved the equation for the force, we have the acceleration, and can now determine the force of a rock of a given mass, $ m $, by applying this derived equation, $ F=m×g $.
For more involved situations the free body diagram is drawn, identifying all of the forces on each mass, taking into account any constraints, and then applying Newton's Laws of Motion to each mass, taking into account all circumstances. Things can get complicated very quickly.
For your question, let's put the rock on the ground, and find the force of gravity on the rock: it's $ F=m×g $, which we found earlier. But clearly the rock is not moving: ther is a constraing force, from the ground, pushing up on the rock. By Newton's 3rd Law of Motion this force is equal and opposite to that of gravity. From the free body diagram you would show an arrow going down for gravity, labelled $ mg $, and one pointing up, the so-called normal force, labeled $ N=-mg $. If you were to push on the rock, so that it slides, the friction would oppose your push, and would equal the magnitude of the normal force times the coefficient for friction. If you were to roll the rock, there would be a lever arm and a force, resulting in a torque, etc.
But stpping with the rock sitting still on the ground, we have found the force, and the force is not zero. you can easily convince yourself of this by putting something compressible between the rock and the ground!
answered May 3, 2016 at 0:32