Could 1 force cause a pure moment?

Question

A friend of mine told me if there is only one force, it cannot cause only rotation. I wasn't convinced so I proposed a thought experiment, and now we are both confused.

Suppose that we put a rod ( uniform density dimensions etc.) in outer space and apply two forces of equal magnitude (F) and opposite directions at the very edges of the rod and at 90 degree angles to it. They would generate a moment about the center of gravity equal to r x 2F

Now suppose we put the same rod in space and put one force with double the magnitude at 90 degrees to the rod at one end. The moment would be equal to r x 2F. Supposedly there would be some translation as well. We have increased the rotational kinetic energy by an equal amount but somehow the translational kinetic energy by different amounts. I'm sure there is an error with my reasoning somewhere, please help me.

Answer 1

The error lies in the theoretical confusion between forces/torques and energy. The kinetic energy is linked to the motion generated by forces and torques which are the causes of the motion itself. Understanding the energy value in the two situations results impossible without knowing the time course of the applied force, being the energy, and so the work, linked to the line integral of the force over a path. Maybe adding more details could be useful:

• if the bar on the left side is continuously subject to a couple of forces acting perpendicularly on its ends, then the bar rotates with a constant angular acceleration $\displaystyle{\alpha = \frac{M_O}{I_O}}$, where $I_O$ is the moment of inertia of the bar with respect to a point $O$, that could be, for the sake of simplicity, its centre of mass.
• as regards the bar on the right side, the situation is more complex, and it is preferable to decouple the two motions by virtually moving the force to the centre of mass and adding a transposition moment given by the product between the force and the distance between the desired application point and the physical application point, $2Fr$; shifting a force is always a licit operation, provided that a moment is added in order to respect the equilibrium. If then a a force is constantly acting on the right end of the bar always perpendicular to the bar, regardless of the space position of the bar, then a 'combined' motion will result, the one due to the torque $2Fr$ which cause a rotation around the center of mass like before, and another motion, due to the translation of the center of mass under the action of the force $2F$, but, owing to the fact that, rotating, the bar is changing orientation, it will be again a rotation, around the left end of the bar. Under these assumptions, now the bar is rotating like a clock needle.

Of course this situation is rather unlikely, and usually it is used to study a pure translation of the center of mass (due to the resultant of the forces) + a pure rotation around the center of mass (due to the resultant moment of all the forces w.r.t the center of mass and all the free torques). In this case is easy to compute the total kinetic energy: $$K = \frac{1}{2}mv_C^2 + \frac{1}{2}I_O\omega^2$$ or, in vectorial terms, for a rigid body described by its inertia matrix $\Gamma_O$: $$K = \frac{1}{2}m\|v_C\|^2 + \frac{1}{2}\omega^T\Gamma_O\omega$$

Answer 2

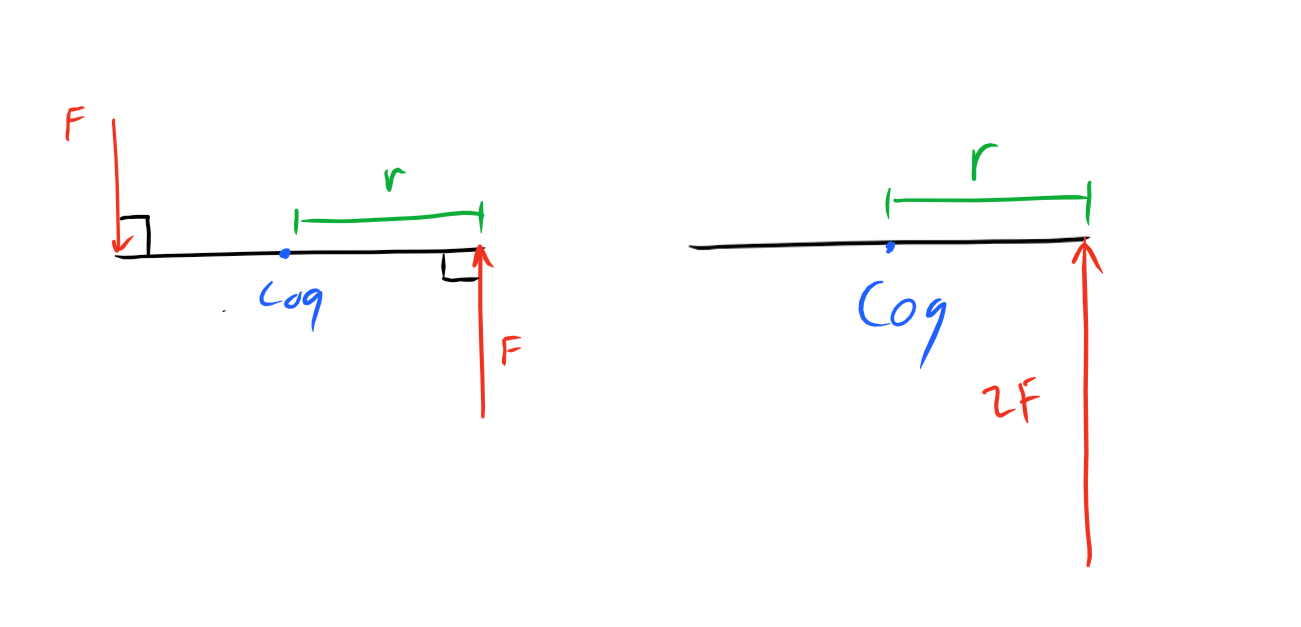
A pure moment cannot be created by one force. In real life, producing pure torques is almost impossible. There will always be a non-zero net force applied also.

In your examples the torque about the center of mass is $\tau_C = 2 r F$ in both cases, but on the second case you also have a net force applied $2 F$ that changes linear momentum also.

So your friends statement "if there is only one force, it cannot cause only rotation." is true. One force will cause rotation and translation (both angular and linear momentum).

So let us consider these forces applied over a short period of time $\Delta t$ and observe the resulting momentum and energy

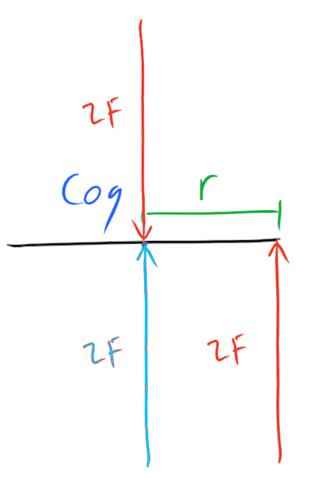
1. Force Couple of $F$
   • Sum of forces $\sum F = 0$.
   • Linear Momentum $\left. p = m v_C = 0 \right\} v_C = 0$.
   • Sum of torques about cog $\sum \tau_C = 2 r F$.
   • Angular Momentum $\left. L = \mathcal{I} \omega = 2 r F \Delta t \right\} \omega = \frac{2 r F \Delta t}{\mathcal{I}} $
   • Kinetic Energy $K = \frac{1}{2} m v_C^2 + \frac{1}{2} \mathcal{I} \omega^2 = \frac{2 F^2 r^2 \Delta t^2}{\mathcal{I}}$
2. Single Force of $2 F$
   • Sum of forces $\sum F = 2 F$.
   • Linear Momentum $\left. p = m v_C = 2 F \Delta t \right\} v_C = \frac{F \Delta t}{m}$.
   • Sum of torques about cog $\sum \tau_C = 2 r F$.
   • Angular Momentum $\left. L = \mathcal{I} \omega = 2 r F \Delta t \right\} \omega = \frac{2 r F \Delta t}{\mathcal{I}} $
   • Kinetic Energy $K = \frac{1}{2} m v_C^2 + \frac{1}{2} \mathcal{I} \omega^2 =\frac{2 F^2 \Delta t^2}{m} + \frac{2 F^2 r^2 \Delta t^2}{\mathcal{I}}$

These two scenarios are different because of their different linear momentum. In some ways rotations, linear momentum and forces are primary quantities and velocities, angular momentum and torques are secondary. See this answer for more details.

Under this viewpoint, a torque is just a force at a distance, just as angular momentum is linear momentum at a distance just as velocity is rotation at a distance. This is generally true, but there are some special (degenerate) cases to consider (at least mathematically).

• (Zero) Rotation about a point at infinity results in pure translation.
• (Zero) Linear momentum along a line at infinity results in pure angular momentum.
• (Zero) Force along a line at infinity results in a pure torque.

When you have two equal and opposite forces (or two equal and opposite momenta, like a rotating dumbbell) the equipollent force system is a single zero force at infinity or equivalently a pure torque.

Answer 3

To your diagram I have added two forces of magnitude $2F$ acting at the centre of mass of the rod.

So you can see that the rod is now being acted on by a couple (red) $2Fd$ which will produce only rotational motion and no translational motion of the centre of mass and a force (blue) $2F$ whose line of action goes through the centre of mass of the rod and so will produce only translational motion of the centre of mass and no rotation.
So with your couple two forces of magnitude $F$ and separation $d$ the rod will only gain rotational kinetic energy but with the single force $2F$ the rod gains both rotational and translational kinetic energy.

In the one force $2F$ case you can think of the work done this force as being more because as well as rotating the rod it has to move further (therefore mre work done) to "keep up" with the rod because of its translational motion.