Brightness of 2 Lightbulbs hooked up to Capacitor [closed]

Question

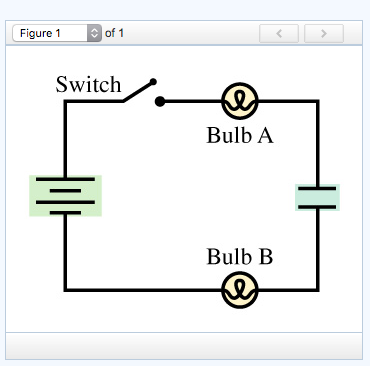
The figure shows a circuit consisting of a battery, a switch, two identical lightbulbs, and a capacitor that is initially uncharged.

a. Immediately after the switch is closed, are either or both bulbs glowing? Explain.

b. If both bulbs are glowing, which is brighter? Or are they equally bright? Explain.

c. For any bulb (A or B or both) that lights up immediately after the switch is closed, does its brightness increase with time, decrease with time, or remain unchanged? Explain.

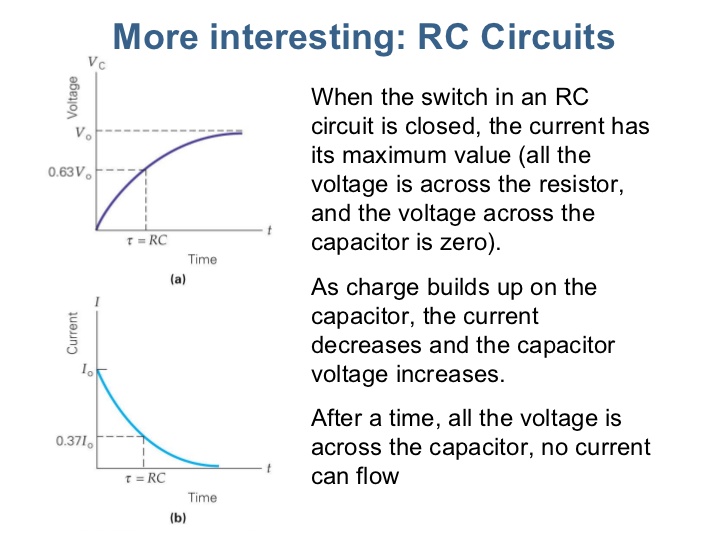
I really don't understand the concept of how a capacitor affects the flow of current in a circuit, and am having an incredibly hard time figuring out this question. At least an idea of where to start would be great.

I had thought that for part A both light bulbs would begin glowing since the capacitor isn't charged, but i have no idea how to tell which one is brighter. I also think that for part C, the brightness of the bulbs would decrease over time after the capacitor is charged because once it's charged the voltage of the capacitor equals the voltage of the battery, hence there is no voltage potential and no current. Am I on the right track at all for this???

Answer 1

a. Immediately after the switch is closed, are either or both bulbs glowing? Explain.

They will both glow as some current passes through them as the capacitor is charging.

b. If both bulbs are glowing, which is brighter? Or are they equally bright? Explain.

They are both equally bright, because an equal and opposite charge is flowing on to either plate of the capacitor, and the two light bulbs are identical.

c. For any bulb (A or B or both) that lights up immediately after the switch is closed, does its brightness increase with time, decrease with time, or remain unchanged? Explain.

Both bulb's brightnesses decrease with time. After the capacitor is fully charged, no current flows in the circuit and thus there is nothing to light the bulbs up.

Answer 2

You're on the right track. You're probably familiar with how the current decreases exponentially after closing the switch.

$$I(t)=I_0 e^{-t/\tau}$$

Where $\tau$ is the time constant of the circuit given by $\tau = RC$, and $R$ is the total resistance of the bulbs. So when the switch is closed, current will be a maximum, and the bulbs brightest. As time goes on, the bulbs will dim according to the exponential decay of the current.

To help you understand the capacitor behaviour better, the usual circuit rule of current in = current out applies. This means the current on either side of the capacitor is the same, and also the current through either bulb is the same, and thus the bulbs are equally bright. When you have charge flowing into one side of the capacitor, the same charge is flowing out the other side of the capacitor, to keep both plates of the capacitor equally and oppositely charged.

Answer 3

You can solve this problem by using Kirchhoff's two laws.

Kirchooff's current law tells you that since this is a series circuit the current in each part of the circuit will be the same all the time.
So whatever happens to bulb $A$ will also happen to bulb $B$ as they both have the same current flowing through them.

Using Kirchhoff's voltage law you have

$$V = V_A + V_B + V_C$$

where $V$ if the emf of the supply, $V_A$ is the voltage across resistor $A$, $V_B$ is the voltage across resistor $B$ and $V_C$ is the voltage across capacitor $C$ and $V_A = V_B = IR$ where $I$ is the current in the circuit and $R$ is the resistance of the bulbs.

The capacitor is initially unchanged so what is the initial value of $V_C$ and hence about $V_A$ and $V_B$?

As the capacitor charges up what happens to $V_C$ and hence to $V_A$ and $V_B$?

Answer 4

I had thought that for part A both light bulbs would begin glowing since the capacitor isn't charged, but i have no idea how to tell which one is brighter.

I would reason about this in the following way.

(1) This is a series circuit and so, the current through each circuit element (battery, bulbs, capacitor, switch) is identical.

(2) The bulbs are identical

(3) Thus, from (1) and (2), the bulbs have identical brightness. That is, two identical bulbs with identical current through glow identically bright.

The presence of the capacitor means that, after the switch is closed, the series current will 'instantly' rise to a maximum level and then decay to zero. If we model the light bulbs as ideal resistors, the decay will be exponential: $i = I_0e^{-t/\tau}$

Image credit

However, the resistance of physical light bulbs is not constant and so, in fact, the decay will not be identical with the ideal resistive case though, in practice, the difference might be insignificant.

Image credit

Answer 5

You have to keep this important thing in mind while dealing with problems of these kind. A capacitor behaves as a pure conductor immediately after connecting it with the circuit (i.e., at time t=0). As time passes the capacitor begins to lose its conductance exponentially and finally after a very long time (theoretically when time t tends to infinity) it behaves like a pure insulator(high resistance).

Coming back to your question:

a.Both bulbs will glow after immediately closing the switch

b. The first one will always glow brighter than the second one as they are connected in series , with their brightness decreasing gradually until both are out(because then no current will flow in the circuit due to the high resistance)

c. Oh well I've answered c.....

Hope this clears your doubt.